- Research Article
- Open access
- Published:
Hypersingular Marcinkiewicz Integrals along Surface with Variable Kernels on Sobolev Space and Hardy-Sobolev Space
Journal of Inequalities and Applications volume 2011, Article number: 479576 (2011)
Abstract
Let  , the authors introduce in this paper a class of the hypersingular Marcinkiewicz integrals along surface with variable kernels defined by
, the authors introduce in this paper a class of the hypersingular Marcinkiewicz integrals along surface with variable kernels defined by 
 , where
, where  with
with  . The authors prove that the operator
. The authors prove that the operator  is bounded from Sobolev space
is bounded from Sobolev space  to
to  space for
space for  , and from Hardy-Sobolev space
, and from Hardy-Sobolev space  to
to  space for
space for  . As corollaries of the result, they also prove the
. As corollaries of the result, they also prove the  boundedness of the Littlewood-Paley type operators
boundedness of the Littlewood-Paley type operators  and
and  which relate to the Lusin area integral and the Littlewood-Paley
which relate to the Lusin area integral and the Littlewood-Paley  function.
function.
1. Introduction
Let  be the
be the  -dimensional Euclidean space and
-dimensional Euclidean space and  be the unit sphere in
be the unit sphere in  equipped with the normalized Lebesgue measure
equipped with the normalized Lebesgue measure  . For
. For  , let
, let  .
.
Before stating our theorems, we first introduce some definitions about the variable kernel  . A function
. A function  defined on
defined on  is said to be in
is said to be in  ,
,  , if
, if  satisfies the following two conditions:
satisfies the following two conditions:
(1) , for any
, for any  and any
and any  ;
;
(2) .
.
In 1955, Calderón and Zygmund [1] investigated the  boundedness of the singular integrals
boundedness of the singular integrals  with variable kernel. They found that these operators connect closely with the problem about the second-order linear elliptic equations with variable coefficients. In 2002, Tang and Yang [2] gave
with variable kernel. They found that these operators connect closely with the problem about the second-order linear elliptic equations with variable coefficients. In 2002, Tang and Yang [2] gave  boundedness of the singular integrals with variable kernels associated to surfaces of the form
boundedness of the singular integrals with variable kernels associated to surfaces of the form  , where
, where  for any
for any  . That is, they considered the variable Calderón-Zygmund singular integral operator
. That is, they considered the variable Calderón-Zygmund singular integral operator  defined by
defined by

On the other hand, as a related vector-valued singular integral with variable kernel, the Marcinkiewicz singular with rough variable kernel associated with surfaces of the form  is considered. It is defined by
is considered. It is defined by

where


If  , we put
, we put  . Historically, the higher dimension Marcinkiewicz integral operator
. Historically, the higher dimension Marcinkiewicz integral operator  with convolution kernel, that is
with convolution kernel, that is  , was first defined and studied by Stein [3] in 1958. See also [4–6] for some further works on
, was first defined and studied by Stein [3] in 1958. See also [4–6] for some further works on  with convolution kernel. Recently, Xue and Yabuta [7] studied the
with convolution kernel. Recently, Xue and Yabuta [7] studied the  boundedness of the operator
boundedness of the operator  with variable kernel.
with variable kernel.
Theorem 1.1 (see [7]).
Suppose that  is positively homogeneous in
is positively homogeneous in  of degree 0, and satisfies (1.4) and
of degree 0, and satisfies (1.4) and
(2′) , for some
, for some  . Let
. Let  be a positive and monotonic (or negative and monotonic)
be a positive and monotonic (or negative and monotonic)  function on
function on  and let it satisfy the following conditions:
and let it satisfy the following conditions:
(i) for some
for some  ;
;
(ii) is monotonic on
is monotonic on  .
.
Then there is a constant C such that  , where constant
, where constant  is independent of
is independent of  .
.
Since the condition (2) implies (2′), so the  boundedness of
boundedness of  holds if
holds if  with
with  .
.
Our aim of this paper is to study the hypersingular Marcinkiewicz integral  along surfaces with variable kernel
along surfaces with variable kernel  , and with index
, and with index  , on the homogeneous Sobolev space
, on the homogeneous Sobolev space  for
for  and the homogeneous Hardy-Sobolev space
and the homogeneous Hardy-Sobolev space  for some
for some  . Let
. Let  be as above, we then define the operators
be as above, we then define the operators  by
by

Our main results are as follows.
Theorem 1.2.
Suppose that  ,
,  satisfies (1.4) and
satisfies (1.4) and  with
with  . Let
. Let  be a positive and increasing
be a positive and increasing  function on
function on  and let it satisfy the following conditions:
and let it satisfy the following conditions:
(i) ;
;
(ii) on
on  .
.
Then there is a constant C such that  , where constant
, where constant  is independent of
is independent of  .
.
Theorem 1.3.
Suppose  , and that
, and that  , with
, with  , and satisfies (1.4). Let
, and satisfies (1.4). Let  be a positive and increasing
be a positive and increasing  function on
function on  and let it satisfy the following conditions:
and let it satisfy the following conditions:
(i) ;
;
(ii) ,
,  .
.
Then, for  , there is a constant C such that
, there is a constant C such that  , where constant
, where constant  is independent of any
is independent of any  .
.
Furthermore, our result can be extended to the Littlewood-Paley type operators  and
and  with variable kernels and index
with variable kernels and index  , which relate to the Lusin area integral and the Littlewood-Paley
, which relate to the Lusin area integral and the Littlewood-Paley  function, respectively. Let
function, respectively. Let  be as above, we then define the operators
be as above, we then define the operators  and
and  for
for  , respectively by
, respectively by

with  , where
, where  . As an application of Theorem 1.2, we have the following conclusion.
. As an application of Theorem 1.2, we have the following conclusion.
Theorem 1.4.
Under the assumption of Theorem 1.2, then Theorem 1.2 still holds for  and
and  .
.
By Theorems 1.2 and 1.3 and applying the interpolation theorem of sublinear operator, we obtain the  boundedness of
boundedness of  .
.
Corollary 1.5.
Suppose  , and that
, and that  ,
,  , and satisfies (1.4). Let
, and satisfies (1.4). Let  be given as in Theorem 1.3. Then, for
be given as in Theorem 1.3. Then, for  , there exists an absolute positive constant
, there exists an absolute positive constant  such that
such that

for all  .
.
Remark 1.6.
It is obvious that the conclusions of Theorem 1.2 are the substantial improvements and extensions of Stein's results in [3] about the Marcinkiewicz integral  with convolution kernel, and of Ding's results in [8] about the Marcinkiewicz integral
with convolution kernel, and of Ding's results in [8] about the Marcinkiewicz integral  with variable kernels.
with variable kernels.
Remark 1.7.
Recently, the authors in [9] proved the boundedness of hypersingular Marcinkiewicz integral with variable kernels on homogeneous Sobolev space  for
for  and
and  without any smoothness on
without any smoothness on  . So Corollary 1.5 extended the results in [9, Theorem 5].
. So Corollary 1.5 extended the results in [9, Theorem 5].
Throughout this paper, the letter  always remains to denote a positive constant not necessarily the same at each occurrence.
always remains to denote a positive constant not necessarily the same at each occurrence.
2. The Bounedness on Sobolev Spaces
Before giving the definition of the Sobolev space, let us first recall the Triebel-Lizorkin space.
Fix a radial function  satisfying
satisfying  and
and  , and
, and  if
if  . Let
. Let  . Define the function
. Define the function  by
by  , such that
, such that  .
.
For  ,
,  , and
, and  , the homogeneous Triebel-Lizorkin space
, the homogeneous Triebel-Lizorkin space  is the set of all distributions
is the set of all distributions  satisfying
satisfying

For  , the homogeneous Sobolev spaces
, the homogeneous Sobolev spaces  is defined by
is defined by  , namely
, namely  . From [10] we know that for any
. From [10] we know that for any 

and if  is a nonnegative integer, then for any
is a nonnegative integer, then for any 

For  , we define the homogeneous Hardy-Sobolev space
, we define the homogeneous Hardy-Sobolev space  by
by  . It is well known that
. It is well known that  for
for  , one can refer [10] for the details.
, one can refer [10] for the details.
Next, let us give the main lemmas we will use in proving theorems.
Lemma 2.1 (see [11]).
Suppose that  and
and  has the form
has the form  where
where  is a solid spherical harmonic polynomial of degree
is a solid spherical harmonic polynomial of degree  . Then the Fourier transform of
. Then the Fourier transform of  has the form
has the form  , where
, where

and  ,
,  is the Bessel function.
is the Bessel function.
Lemma 2.2 (see [12]).
For  , and
, and  , there exists
, there exists  such that for any
such that for any  and
and  ,
,

Lemma 2.3.
Let  ,
,  ,
,  is a
is a  function on
function on  and let it satisfy the conditions (i) and (ii) in Theorem 1.2.
and let it satisfy the conditions (i) and (ii) in Theorem 1.2.
Denote  , if
, if

Then there exists a constant  independent of
independent of  , such that
, such that  for every integer
for every integer  .
.
Proof.
Let  , then we have
, then we have

So it suffices to show  .
.
Decompose this integral into two parts  .
.
For  , by using Lemma 2.2 and
, by using Lemma 2.2 and  , we can get
, we can get

For the other part  , applying Stirling's formula, we have
, applying Stirling's formula, we have

Also in [13], the authors proved the following inequality

So by (2.9) and (2.10),  , and noting that
, and noting that  , we have
, we have

So far we can deduce the desired conclusion of Lemma 2.3.
Proof of Theorem 1.2.
The basic idea of proof can go back to [14], for recently papers, one see [8, 15]. By the same argument as in [1], let  denote the complete system of normalized surface spherical harmonics. See [14] for instance, we can decompose
denote the complete system of normalized surface spherical harmonics. See [14] for instance, we can decompose  as following:
as following:

Denote

then we get

Then, applying Hölder inequality twice, we have for any  that
that

By [14, page 230, equation (4.4)], we can observe that the series in the first parenthesis on the right-hand side of the inequality above, for each  fixed, is equal to
fixed, is equal to  , where
, where  is the Sobolev space on
is the Sobolev space on  with
with  for any
for any  . So if we take
. So if we take  sufficiently close to 1, then by the Sobolev imbedding theorem
sufficiently close to 1, then by the Sobolev imbedding theorem  , we have
, we have

with  .
.
By Fourier transform and (2.16), we get

For  , we have
, we have

For the integral on the right hand side of the above inequality, by changing of variable, we can get

So we have

Put  and
and  , we can deduce from Lemma 2.1 that
, we can deduce from Lemma 2.1 that

where

Hence, we have

By [14], we know that  .
.
So we can get

Set  ,
,  and note that
and note that  , we can deduce that
, we can deduce that

Noting that  is increasing, by using the second mean-value theorem, we get, for some
is increasing, by using the second mean-value theorem, we get, for some  ,
,

From (2.26), it follows that

Thus using Lemma 2.3, we can deduce the desired conclusion of Theorem 1.2.
Proof of Theorem 1.4.
First, we know that  . On the other hand,
. On the other hand,

Thus, using Theorem 1.2, we can finish Theorem 1.4.
3. The Bounedness on Hardy-Sobolev Spaces
In order to prove the boundedness for operator  on Hardy-Sobolev spaces and prove Theorem 1.3, we first introduce a new kind of atomic decomposition for Hardy-Sobolev space as following which will be used next.
on Hardy-Sobolev spaces and prove Theorem 1.3, we first introduce a new kind of atomic decomposition for Hardy-Sobolev space as following which will be used next.
Definition 3.1 (see [16]).
For  , the function
, the function  is called a
is called a  atom if it satisfies the following three conditions:
atom if it satisfies the following three conditions:
(1) with a ball
with a ball  ;
;
(2) ;
;
(3) , for any polynomial
, for any polynomial  of degree
of degree  .
.
By [16], we have that every  can be written as a sum of
can be written as a sum of  atoms
atoms  , that is,
, that is,

Proof of Theorem 1.3.
Similar to the argument of Lemma 3.3 in [17] and using above atomic decomposition, it suffices to show that

with the constant  independent of any
independent of any  atom
atom  .
.
Assume  . We first note that
. We first note that

For  , using Theorem 1.2, it is not difficult to deduce that
, using Theorem 1.2, it is not difficult to deduce that

For  , we first consider the case
, we first consider the case  , according to [15, Lemma 5.5], for
, according to [15, Lemma 5.5], for  and
and  atom
atom  with support
with support  , one has
, one has

Using Minkowski inequality and Hölder inequality for integrals, and (3.5), we can get

For the integral on the right hand side of the above inequality, by changing of variable and noting that  ,
,  , we can get
, we can get

By (3.7), we can get

Thus by (3.5) and the condition  ,
,

As for  , similar to the argument of
, similar to the argument of  , we can easily get
, we can easily get  . So far the proof of Theorem 1.3 has been finished.
. So far the proof of Theorem 1.3 has been finished.
References
Calderón AP, Zygmund A: On a problem of Mihlin. Transactions of the American Mathematical Society 1955, 78: 209–224.
Tang L, Yang D: Boundedness of singular integrals of variable rough Calderón-Zygmund kernels along surfaces. Integral Equations and Operator Theory 2002,43(4):488–502. 10.1007/BF01212707
Stein EM: On the functions of Littlewood-Paley, Lusin, and Marcinkiewicz. Transactions of the American Mathematical Society 1958, 88: 430–466. 10.1090/S0002-9947-1958-0112932-2
Tao XX, Wei RY: Boundedness of commutators related to Marcinkiewicz integrals with variable kernels in Herz-type Hardy spaces. Acta Mathematica Scientia 2009,29(6):1508–1517.
Fan D, Sato S: Weak type
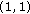 estimates for Marcinkiewicz integrals with rough kernels. The Tohoku Mathematical Journal 2001,53(2):265–284. 10.2748/tmj/1178207481
estimates for Marcinkiewicz integrals with rough kernels. The Tohoku Mathematical Journal 2001,53(2):265–284. 10.2748/tmj/1178207481Sakamoto M, Yabuta K: Boundedness of Marcinkiewicz functions. Studia Mathematica 1999,135(2):103–142.
Xue Q, Yabuta K:
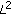 -boundedness of Marcinkiewicz integrals along surfaces with variable kernels: another sufficient condition. Journal of Inequalities and Applications 2007, 2007:-14.
-boundedness of Marcinkiewicz integrals along surfaces with variable kernels: another sufficient condition. Journal of Inequalities and Applications 2007, 2007:-14.Ding Y, Lin C-C, Shao S: On the Marcinkiewicz integral with variable kernels. Indiana University Mathematics Journal 2004,53(3):805–821. 10.1512/iumj.2004.53.2406
Chen J, Ding Y, Fan D: Littlewood-Paley operators with variable kernels. Science in China Series A 2006,49(5):639–650. 10.1007/s11425-006-0639-y
Frazier M, Jawerth B, Weiss G: Littlewood-Paley Theory and the Study of Function Spaces, CBMS Regional Conference Series in Mathematics. Volume 79. American Mathematical Society, Washington, DC, USA; 1991:viii+132.
Stein EM, Weiss G: Introduction to Fourier Analysis on Euclidean Spaces. Princeton University Press, Princeton, NJ, USA; 1971:x+297. Princeton Mathematical Series, No. 3
Ding Y, Li R: An estimate of Bessel function and its application. Science in China Series A 2008,38(1):78–87.
Aguilera NE, Harboure EO: Some inequalities for maximal operators. Indiana University Mathematics Journal 1980,29(4):559–576. 10.1512/iumj.1980.29.29042
Calderón A-P, Zygmund A: On singular integrals with variable kernels. Applicable Analysis 1977–1978,7(3):221–238.
Chen J, Fan D, Ying Y: Certain operators with rough singular kernels. Canadian Journal of Mathematics 2003,55(3):504–532. 10.4153/CJM-2003-021-4
Han Y-S, Paluszyński M, Weiss G: A new atomic decomposition for the Triebel-Lizorkin spaces. In Harmonic Analysis and Operator Theory (Caracas, 1994), Contemporary Mathematics. Volume 189. American Mathematical Society, Providence, RI, USA; 1995:235–249.
Hofmann S, Mayboroda S: Hardy and BMO spaces associated to divergence form elliptic operators. Mathematische Annalen 2009,344(1):37–116. 10.1007/s00208-008-0295-3
Acknowledgments
This project supported by the National Natural Science Foundation of China under Grant no. 10747141, Zhejiang Provincial National Natural Science Foundation of China under Grant no. Y604056, and Science Foundation of Shaoguan University under Grant no. 200915001.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Ruiying, W., Yin, L. Hypersingular Marcinkiewicz Integrals along Surface with Variable Kernels on Sobolev Space and Hardy-Sobolev Space. J Inequal Appl 2011, 479576 (2011). https://doi.org/10.1155/2011/479576
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1155/2011/479576
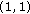 estimates for Marcinkiewicz integrals with rough kernels. The Tohoku Mathematical Journal 2001,53(2):265–284. 10.2748/tmj/1178207481
estimates for Marcinkiewicz integrals with rough kernels. The Tohoku Mathematical Journal 2001,53(2):265–284. 10.2748/tmj/1178207481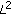 -boundedness of Marcinkiewicz integrals along surfaces with variable kernels: another sufficient condition. Journal of Inequalities and Applications 2007, 2007:-14.
-boundedness of Marcinkiewicz integrals along surfaces with variable kernels: another sufficient condition. Journal of Inequalities and Applications 2007, 2007:-14.