- Research Article
- Open access
- Published:
Mixed Monotone Iterative Technique for Abstract Impulsive Evolution Equations in Banach Spaces
Journal of Inequalities and Applications volume 2010, Article number: 293410 (2010)
Abstract
By constructing a mixed monotone iterative technique under a new concept of upper and lower solutions, some existence theorems of mild  -periodic (
-periodic ( -quasi) solutions for abstract impulsive evolution equations are obtained in ordered Banach spaces. These results partially generalize and extend the relevant results in ordinary differential equations and partial differential equations.
-quasi) solutions for abstract impulsive evolution equations are obtained in ordered Banach spaces. These results partially generalize and extend the relevant results in ordinary differential equations and partial differential equations.
1. Introduction and Main Result
Impulsive differential equations are a basic tool for studying evolution processes of real life phenomena that are subjected to sudden changes at certain instants. In view of multiple applications of the impulsive differential equations, it is necessary to develop the methods for their solvability. Unfortunately, a comparatively small class of impulsive differential equations can be solved analytically. Therefore, it is necessary to establish approximation methods for finding solutions. The monotone iterative technique of Lakshmikantham et al. (see [1–3]) is such a method which can be applied in practice easily. This technique combines the idea of method of upper and lower solutions with appropriate monotone conditions. Recent results by means of monotone iterative method are obtained in [4–7] and the references therein. In this paper, by using a mixed monotone iterative technique in the presence of coupled lower and upper  -quasisolutions, we consider the existence of mild
-quasisolutions, we consider the existence of mild  -periodic (
-periodic ( -quasi)solutions for the periodic boundary value problem (PBVP) of impulsive evolution equations
-quasi)solutions for the periodic boundary value problem (PBVP) of impulsive evolution equations

in an ordered Banach space  , where
, where  is a closed linear operator and
is a closed linear operator and  generates a
generates a  -semigroup
-semigroup  in
in  ;
;  only satisfies weak Carathé odory condition,
only satisfies weak Carathé odory condition,  ,
,  is a constant;
is a constant;  ;
;  is an impulsive function,
is an impulsive function,  ;
;  denotes the jump of
denotes the jump of  at
at  , that is,
, that is,  , where
, where  and
and  represent the right and left limits of
represent the right and left limits of  at
at  , respectively. Let
, respectively. Let  is continuous at
is continuous at  and left continuous at
and left continuous at  , and
, and  exists,
exists,  . Evidently,
. Evidently,  is a Banach space with the norm
is a Banach space with the norm  . Let
. Let  ,
,  . Denote by
. Denote by  the Banach space generated by
the Banach space generated by  with the norm
with the norm  . An abstract function
. An abstract function  is called a solution of the PBVP(1.1) if
is called a solution of the PBVP(1.1) if  satisfies all the equalities of (1.1).
satisfies all the equalities of (1.1).
Let  be an ordered Banach space with the norm
be an ordered Banach space with the norm  and the partial order "
and the partial order " ", whose positive cone
", whose positive cone  is normal with a normal constant
is normal with a normal constant  . Let
. Let  . If functions
. If functions  satisfy
satisfy


we call  coupled lower and upper
coupled lower and upper  -quasisolutions of the PBVP(1.1). Only choosing "
-quasisolutions of the PBVP(1.1). Only choosing " " in (1.2) and (1.3), we call
" in (1.2) and (1.3), we call  coupled
coupled  -periodic
-periodic  -quasisolution pair of the PBVP(1.1). Furthermore, if
-quasisolution pair of the PBVP(1.1). Furthermore, if  , we call
, we call  an
an  -periodic solution of the PBVP(1.1).
-periodic solution of the PBVP(1.1).
Definition 1.1.
Abstract functions  are called a coupled mild
are called a coupled mild  -periodic
-periodic  -quasisolution pair of the PBVP(1.1) if
-quasisolution pair of the PBVP(1.1) if  and
and  satisfy the following integral equations:
satisfy the following integral equations:

where  and
and  for any
for any  ,
,  is an identity operator. If
is an identity operator. If  , then
, then  is called a mild
is called a mild  -periodic solution of the PBVP(1.1).
-periodic solution of the PBVP(1.1).
Without impulse, the PBVP(1.1) has been studied by many authors, see [8–11] and the references therein. In particular, Shen and Li [11] considered the existence of coupled mild  -periodic quasisolution pair for the following periodic boundary value problem (PBVP) in
-periodic quasisolution pair for the following periodic boundary value problem (PBVP) in  :
:

where  is continuous. Under one of the following situations:
is continuous. Under one of the following situations:
(i) is a compact semigroup,
is a compact semigroup,
(ii) is regular in
is regular in  and
and  is continuous in operator norm for
is continuous in operator norm for  ,
,
they built a mixed monotone iterative method for the PBVP(1.5), and they proved that, if the PBVP(1.5) has coupled lower and upper quasisolutions (i.e.,  and without impulse in (1.2) and (1.3))
and without impulse in (1.2) and (1.3))  and
and  with
with  , nonlinear term
, nonlinear term  satisfies one of the following conditions:
satisfies one of the following conditions:
(F1) is mixed monotone,
is mixed monotone,
(F2)There exists a constant  such that
such that

and  is nonincreasing on
is nonincreasing on  .
.
Then the PBVP(1.5) has minimal and maximal coupled mild  -periodic quasisolutions between
-periodic quasisolutions between  and
and  , which can be obtained by monotone iterative sequences from
, which can be obtained by monotone iterative sequences from  and
and  . But conditions
. But conditions  and
and  are difficult to satisfy in applications except some special situations.
are difficult to satisfy in applications except some special situations.
In this paper, by constructing a mixed monotone iterative technique under a new concept of upper and lower solutions, we will discuss the existence of mild  -periodic (
-periodic ( -quasi) solutions for the impulsive evolution Equation(1.1) in an ordered Banach space
-quasi) solutions for the impulsive evolution Equation(1.1) in an ordered Banach space  . In our results, we will delete conditions
. In our results, we will delete conditions  and
and  for the operator semigroup
for the operator semigroup  , and improve conditions
, and improve conditions  and
and  for nonlinearity
for nonlinearity  . In addition, we only require that the nonlinear term
. In addition, we only require that the nonlinear term  satisfies weak Carathé odory condition:
satisfies weak Carathé odory condition:
(1)for each  is strongly measurable.
is strongly measurable.
(2)for a.e. is subcontinuous, namely, there exists
is subcontinuous, namely, there exists  with mes
with mes  such that
such that

for any  , and
, and  .
.
Our main result is as follows:
Theorem 1.2.
Let  be an ordered and weakly sequentially complete Banach space, whose positive cone
be an ordered and weakly sequentially complete Banach space, whose positive cone  is normal,
is normal,  be a closed linear operator and
be a closed linear operator and  generate a positive
generate a positive  -semigroup
-semigroup  in
in  . If the PBVP(1.1) has coupled lower and upper
. If the PBVP(1.1) has coupled lower and upper  -quasisolutions
-quasisolutions  and
and  with
with  , nonlinear term
, nonlinear term  and impulsive functions
and impulsive functions  's satisfy the following conditions
's satisfy the following conditions
(H1) There exist constants  and
and  such that
such that

for any  , and
, and  .
.
(H2) Impulsive function  is continuous, and for any
is continuous, and for any  , it satisfies
, it satisfies

for any  , and
, and  .
.
then the PBVP(1.1) has minimal and maximal coupled mild  -periodic
-periodic  -quasisolutions between
-quasisolutions between  and
and  , which can be obtained by monotone iterative sequences starting from
, which can be obtained by monotone iterative sequences starting from  and
and  .
.
Evidently, condition  contains conditions
contains conditions  and
and  . Hence, even without impulse in PBVP(1.1), Theorem 1.2 still extends the results in [10, 11].
. Hence, even without impulse in PBVP(1.1), Theorem 1.2 still extends the results in [10, 11].
The proof of Theorem 1.2 will be shown in the next section. In Section 2, we also discuss the existence of mild  -periodic solutions for the PBVP(1.1) between coupled lower and upper
-periodic solutions for the PBVP(1.1) between coupled lower and upper  -quasisolutions (see Theorem 2.3). In Section 3, the results obtained will be applied to a class of partial differential equations of parabolic type.
-quasisolutions (see Theorem 2.3). In Section 3, the results obtained will be applied to a class of partial differential equations of parabolic type.
2. Proof of the Main Results
Let  be a Banach space,
be a Banach space,  be a closed linear operator, and
be a closed linear operator, and  generate a
generate a  -semigroup
-semigroup  in
in  . Then there exist constants
. Then there exist constants  and
and  such that
such that

Definition 2.1.
A  -semigroup
-semigroup  is said to be exponentially stable in
is said to be exponentially stable in  if there exist constants
if there exist constants  and
and  such that
such that

Let  . Denote by
. Denote by  the Banach space of all continuous
the Banach space of all continuous  -value functions on interval
-value functions on interval  with the norm
with the norm  . It is well-known ([12, Chapter 4, Theorem
. It is well-known ([12, Chapter 4, Theorem  ]) that for any
]) that for any  and
and  , the initial value problem(IVP) of linear evolution equation
, the initial value problem(IVP) of linear evolution equation

has a unique classical solution  expressed by
expressed by

If  and
and  , the function
, the function  given by (2.4) belongs to
given by (2.4) belongs to  . We call it a mild solution of the IVP(2.3).
. We call it a mild solution of the IVP(2.3).
To prove Theorem 1.2, for any  , we consider the periodic boundary value problem (PBVP) of linear impulsive evolution equation in
, we consider the periodic boundary value problem (PBVP) of linear impulsive evolution equation in 

where  .
.
Lemma 2.2.
Let  be an exponentially stable
be an exponentially stable  -semigroup in
-semigroup in  . Then for any
. Then for any  and
and  , the linear PBVP(2.5) has a unique mild solution
, the linear PBVP(2.5) has a unique mild solution  given by
given by

where  .
.
Proof.
For any  , we first show that the initial value problem (IVP) of linear impulsive evolution equation
, we first show that the initial value problem (IVP) of linear impulsive evolution equation

has a unique mild solution  given by
given by

where  and
and  .
.
Let  . Let
. Let  . If
. If  is a mild solution of the linear IVP(2.7), then the restriction of
is a mild solution of the linear IVP(2.7), then the restriction of  on
on  satisfies the initial value problem (IVP) of linear evolution equation without impulse
satisfies the initial value problem (IVP) of linear evolution equation without impulse

Hence, on  ,
,  can be expressed by
can be expressed by

Iterating successively in the above equality with  for
for  , we see that
, we see that  satisfies (2.8).
satisfies (2.8).
Inversely, we can verify directly that the function  defined by (2.8) is a solution of the linear IVP(2.7). Hence the linear IVP(2.7) has a unique mild solution
defined by (2.8) is a solution of the linear IVP(2.7). Hence the linear IVP(2.7) has a unique mild solution  given by (2.8).
given by (2.8).
Next, we show that the linear PBVP(2.5) has a unique mild solution  given by (2.6).
given by (2.6).
If a function  defined by (2.8) is a solution of the linear PBVP(2.5), then
defined by (2.8) is a solution of the linear PBVP(2.5), then  , namely,
, namely,

Since  is exponentially stable, we define an equivalent norm in
is exponentially stable, we define an equivalent norm in  by
by

Then  and
and  , and especially,
, and especially,  . It follows that
. It follows that  has a bounded inverse operator
has a bounded inverse operator  , which is a positive operator when
, which is a positive operator when  is a positive semigroup. Hence we choose
is a positive semigroup. Hence we choose  . Then
. Then  is the unique initial value of the IVP(2.7) in
is the unique initial value of the IVP(2.7) in  , which satisfies
, which satisfies  . Combining this fact with (2.8), it follows that (2.6) is satisfied.
. Combining this fact with (2.8), it follows that (2.6) is satisfied.
Inversely, we can verify directly that the function  defined by (2.6) is a solution of the linear PBVP(2.5). Therefore, the conclusion of Lemma 2.2 holds.
defined by (2.6) is a solution of the linear PBVP(2.5). Therefore, the conclusion of Lemma 2.2 holds.
Evidently,  is also an ordered Banach space with the partial order "
is also an ordered Banach space with the partial order " " reduced by positive function cone
" reduced by positive function cone  .
.  is also normal with the same normal constant
is also normal with the same normal constant  . For
. For  with
with  , we use
, we use  to denote the order interval
to denote the order interval  in
in  , and
, and  to denote the order interval
to denote the order interval  in
in  . From Lemma 2.2, if
. From Lemma 2.2, if is a positive
is a positive  -semigroup,
-semigroup,  and
and  , then the mild solution
, then the mild solution  of the linear PBVP(2.5) satisfies
of the linear PBVP(2.5) satisfies  .
.
Proof of Theorem 1.2.
We first show that  for any
for any  and
and  . Since
. Since  for any
for any  , from the assumption
, from the assumption  , we have
, we have

Namely,  . From the normality of cone
. From the normality of cone  in
in  , we have
, we have

Combining this fact with the fact that  is strongly measurable, it follows that
is strongly measurable, it follows that  . Therefore, for any
. Therefore, for any  , we consider the periodic boundary value problem(PBVP) of impulsive evolution equation in
, we consider the periodic boundary value problem(PBVP) of impulsive evolution equation in 

where  . Let
. Let  be large enough such that
be large enough such that  (otherwise, replacing
(otherwise, replacing  by
by  , the assumption
, the assumption  still holds). Then
still holds). Then  generates an exponentially stable
generates an exponentially stable  -semigroup
-semigroup  . Obviously,
. Obviously,  is a positive
is a positive  -semigroup and
-semigroup and  for
for  . From Lemma 2.2, the PBVP(2.15) has a unique mild solution
. From Lemma 2.2, the PBVP(2.15) has a unique mild solution  given by
given by

Let  . We define an operator
. We define an operator  by
by

Then the coupled mild  -periodic
-periodic  -quasisolution of the PBVP(1.1) is equivalent to the coupled fixed point of operator
-quasisolution of the PBVP(1.1) is equivalent to the coupled fixed point of operator  .
.
Next, we will prove that the operator  has coupled fixed points on
has coupled fixed points on  . For this purpose, we first show that
. For this purpose, we first show that  is a mixed monotone operator and
is a mixed monotone operator and  . In fact, for any
. In fact, for any  , from assumptions
, from assumptions  and
and  , we have
, we have

Since  is a positive
is a positive  -semigroup, it follows that
-semigroup, it follows that  is a positive operator. Then
is a positive operator. Then  . Hence from (2.17) we see that
. Hence from (2.17) we see that  , which implies that
, which implies that  is a mixed monotone operator. Since
is a mixed monotone operator. Since

from Lemma 2.2 and (1.2), we have

for  . Especially, we have
. Especially, we have

Combining this inequality with  , it follows that
, it follows that

On the other hand, from (2.17), we have

Therefore,  for all
for all  . It implies that
. It implies that  . Similarly, we can prove that
. Similarly, we can prove that  .
.
Now, we define sequences  and
and  by the iterative scheme
by the iterative scheme

Then from the mixed monotonicity of operator  , we have
, we have

Therefore, for any  ,
,  and
and  are monotone order-bounded sequences in
are monotone order-bounded sequences in  . Noticing that
. Noticing that  is a weakly sequentially complete Banach space, then
is a weakly sequentially complete Banach space, then  and
and  are relatively compact in
are relatively compact in  . Combining this fact with the monotonicity of (2.25) and the normality of cone
. Combining this fact with the monotonicity of (2.25) and the normality of cone  in
in  , it follows that
, it follows that  and
and  are uniformly convergent in
are uniformly convergent in  . Let
. Let

Then  are strongly measurable, and
are strongly measurable, and  for any
for any  . Hence,
. Hence,  .
.
At last, we show that  and
and  are coupled mild
are coupled mild  -periodic
-periodic  -quasisolutions of the PBVP(1.1). For any
-quasisolutions of the PBVP(1.1). For any  , from subcontinuity of
, from subcontinuity of  and continuity of
and continuity of  's, there exists
's, there exists  with mes
with mes such that
such that

Hence, for any  and
and  , denote by
, denote by  the adjoint operator of
the adjoint operator of  , then
, then  , and
, and

On the other hand, we have

From Lebesgue's dominated convergence theorem, we have

Hence, from (2.17), we have

On the other hand, it follows from (2.26) that  . Hence
. Hence  . By the uniqueness of limits, we can deduce that
. By the uniqueness of limits, we can deduce that

By the arbitrariness of  , we have
, we have

Similarly, we can prove that  . Therefore,
. Therefore,  is coupled mild
is coupled mild  -periodic
-periodic  -quasisolution pair of the PBVP(1.1).
-quasisolution pair of the PBVP(1.1).
Now, we discuss the existence of mild  -periodic solutions for the PBVP(1.1) on
-periodic solutions for the PBVP(1.1) on  . We assume that the following assumptions are also satisfied:
. We assume that the following assumptions are also satisfied:
(H3) there exists a constant  with
with  such that
such that

for any  , where
, where  ,
,
(H4) there exist positive constants  with
with  such that
such that

for any 
Then we have the following existence and uniqueness result in general ordered Banach space.
Theorem 2.3.
Let  be an ordered Banach space, whose positive cone
be an ordered Banach space, whose positive cone  is normal,
is normal,  be a closed linear operator, and
be a closed linear operator, and  generate a positive
generate a positive  -semigroup
-semigroup  in
in  . If the PBVP(1.1) has coupled lower and upper
. If the PBVP(1.1) has coupled lower and upper  -quasisolution
-quasisolution  and
and  with
with  , nonlinear term
, nonlinear term  and impulsive functions
and impulsive functions  's satisfy the following assumptions:
's satisfy the following assumptions:
(H1)* there exist constants  and
and  such that
such that

for any  , and
, and  .
.
And  , then the PBVP(1.1) has a unique mild
, then the PBVP(1.1) has a unique mild  -periodic solution
-periodic solution  on
on  .
.
Proof.
From the proof of Theorem 1.2, when the conditions  and
and  are satisfied, the iterative sequences
are satisfied, the iterative sequences  and
and  defined by (2.24) satisfy (2.25). We show that there exists a unique
defined by (2.24) satisfy (2.25). We show that there exists a unique  such that
such that  . For any
. For any  , from
, from  , (2.17), (2.24) and (2.25), we have
, (2.17), (2.24) and (2.25), we have

By means of the normality of cone  in
in  , we have
, we have

Therefore

by Repeating the using of the above inequality, we can obtain that

as  . Then there exists a unique
. Then there exists a unique  such that
such that  . Therefore, let
. Therefore, let  in (2.24), from the continuity of operator
in (2.24), from the continuity of operator  , we have
, we have  , which means that
, which means that  is a unique mild
is a unique mild  -periodic solution of the PBVP(1.1).
-periodic solution of the PBVP(1.1).
3. An Example
Let  be a bounded domain with a sufficiently smooth boundary
be a bounded domain with a sufficiently smooth boundary  . Let
. Let  , and
, and  ,
,  . Consider the existence of mild solutions for the boundary value problem of parabolic type:
. Consider the existence of mild solutions for the boundary value problem of parabolic type:

where  is the Laplace operator,
is the Laplace operator,  . Let
. Let  equipped with the
equipped with the  -norm
-norm  ,
,  . Then
. Then  is a generating normal cone in
is a generating normal cone in  . Consider the operator
. Consider the operator  defined by
defined by

Then  generates an analytic semigroup
generates an analytic semigroup  in
in  . By the maximum principle of the equations of parabolic type, it is easy to prove that
. By the maximum principle of the equations of parabolic type, it is easy to prove that  is a positive
is a positive  -semigroup in
-semigroup in  . Let
. Let  be the first eigenvalue of operator
be the first eigenvalue of operator  and
and  be a corresponding positive eigenvector. For solving the problem (3.1), the following assumptions are needed.
be a corresponding positive eigenvector. For solving the problem (3.1), the following assumptions are needed.
(i) There exists a constant  such that
such that
(a)  ,
,  ,
,  ,
, 
(b)  ,
,  ,
,  ,
,  ,
, 
(ii)
(a) The partial derivative of  on
on  is continuous on any bounded domain.
is continuous on any bounded domain.
(b) The partial derivative of  on
on  has upper bound, and
has upper bound, and  .
.
-
(iii)
For any
 with
with 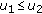 , we have
, we have

Let  and
and  be defined by
be defined by  and by
and by  . Then the problem (3.1) can be transformed into the PBVP(1.1). Assumption
. Then the problem (3.1) can be transformed into the PBVP(1.1). Assumption  implies that
implies that  and
and  are coupled lower and upper
are coupled lower and upper  -quasisolutions of the PBVP(1.1). From assumption (ii)(a), there exists a constant
-quasisolutions of the PBVP(1.1). From assumption (ii)(a), there exists a constant  such that, for any
such that, for any  , we have
, we have

This implies that

for any  and
and  . Hence for any
. Hence for any  and
and  , we have
, we have

Therefore, for any  with
with  , from the assumption
, from the assumption  , we have
, we have

That is, assumption  is satisfied. From
is satisfied. From  , it is easy to see that assumption
, it is easy to see that assumption  is satisfied. Therefore, the following result is deduced from Theorem 1.2.
is satisfied. Therefore, the following result is deduced from Theorem 1.2.
Theorem 3.1.
If the assumptions  are satisfied, then the problem (3.1) has coupled mild
are satisfied, then the problem (3.1) has coupled mild  -periodic
-periodic  -quasisolution pair on
-quasisolution pair on  .
.
Remark 3.2.
In applications of partial differential equations, we often choose Banach space  as working space, which is weakly sequentially complete. Hence the result in Theorem 1.2 is more valuable in applications. In particular, we obtain a unique mild
as working space, which is weakly sequentially complete. Hence the result in Theorem 1.2 is more valuable in applications. In particular, we obtain a unique mild  -periodic solution of the PBVP(1.1) in general ordered Banach space in Theorem 2.3.
-periodic solution of the PBVP(1.1) in general ordered Banach space in Theorem 2.3.
Remark 3.3.
If  , then the coupled lower and upper
, then the coupled lower and upper  -quasisolutions are equivalent to coupled lower and upper quasisolutions of the PBVP(1.1). Since condition
-quasisolutions are equivalent to coupled lower and upper quasisolutions of the PBVP(1.1). Since condition  contains conditions
contains conditions  and
and  , even without impulse in PBVP(1.1), the results in this paper still extend the results in [10, 11].
, even without impulse in PBVP(1.1), the results in this paper still extend the results in [10, 11].
References
Du SW, Lakshmikantham V: Monotone iterative technique for differential equations in a Banach space. Journal of Mathematical Analysis and Applications 1982, 87(2):454–459. 10.1016/0022-247X(82)90134-2
Lakshmikantham V, Vatsala AS: Quasisolutions and monotone method for systems of nonlinear boundary value problems. Journal of Mathematical Analysis and Applications 1981, 79(1):38–47. 10.1016/0022-247X(81)90006-8
Ladde GS, Lakshmikantham V, Vatsala AS: Monotone Iterative Techniques for Nonlinear Differential Equations, Monographs, Advanced Texts and Surveys in Pure and Applied Mathematics. Volume 27. Pitman, Boston, Mass, USA; 1985:x+236.
Lan H-Y: Monotone method for a system of nonlinear mixed type implicit impulsive integro-differential equations in Banach spaces. Journal of Computational and Applied Mathematics 2008, 222(2):531–543. 10.1016/j.cam.2007.11.021
Ahmad B, Sivasundaram S: The monotone iterative technique for impulsive hybrid set valued integro-differential equations. Nonlinear Analysis: Theory, Methods & Applications 2006, 65(12):2260–2276. 10.1016/j.na.2006.01.033
Li Y, Liu Z: Monotone iterative technique for addressing impulsive integro-differential equations in Banach spaces. Nonlinear Analysis: Theory, Methods & Applications 2007, 66(1):83–92. 10.1016/j.na.2005.11.013
Ahmad B, Alsaedi A: Existence of solutions for anti-periodic boundary value problems of nonlinear impulsive functional integro-differential equations of mixed type. Nonlinear Analysis: Theory, Methods & Applications 2009, 3(4):501–509.
Lakshmikantham V, Leela S: Existence and monotone method for periodic solutions of first-order differential equations. Journal of Mathematical Analysis and Applications 1983, 91(1):237–243. 10.1016/0022-247X(83)90102-6
Li YX: Existence and uniqueness of positive periodic solutions for abstract semilinear evolution equations. Journal of Systems Science and Mathematical Sciences 2005, 25(6):720–728.
Li YX: Periodic solutions of semilinear evolution equations in Banach spaces. Acta Mathematica Sinica 1998, 41(3):629–636.
Shen PL, Li FY: Coupled periodic solutions of nonlinear evolution equations in Banach spaces. Acta Mathematica Sinica 2000, 43(4):685–694.
Pazy A: Semigroups of Linear Operators and Applications to Partial Differential Equations, Applied Mathematical Sciences. Volume 44. Springer, New York, NY, USA; 1983:viii+279.
Acknowledgments
The author is very grateful to the reviewers for their helpful comments and suggestions. Research supported by NNSF of China (10871160), the NSF of Gansu Province (0710RJZA103), and Project of NWNUKJCXGC-3-47.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Yang, H. Mixed Monotone Iterative Technique for Abstract Impulsive Evolution Equations in Banach Spaces. J Inequal Appl 2010, 293410 (2010). https://doi.org/10.1155/2010/293410
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1155/2010/293410
 with
with 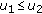 , we have
, we have