- Research Article
- Open access
- Published:
On Linear Maps Preserving g-Majorization from  to
to 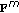
Journal of Inequalities and Applications volume 2010, Article number: 254819 (2010)
Abstract
Let  and
and  be the usual spaces of n-dimensional column and m-dimensional row vectors on
be the usual spaces of n-dimensional column and m-dimensional row vectors on  , respectively, where
, respectively, where  is the field of real or complex numbers. In this paper, the relations gs-majorization, lgw-majorization, and rgw-majorization are considered on
is the field of real or complex numbers. In this paper, the relations gs-majorization, lgw-majorization, and rgw-majorization are considered on  and
and  . Then linear maps
. Then linear maps  preserving lgw-majorization or gs-majorization and linear maps
preserving lgw-majorization or gs-majorization and linear maps  , preserving rgw-majorization are characterized.
, preserving rgw-majorization are characterized.
1. Introduction
Majorization is a topic of much interest in various areas of mathematics and statistics. If  and
and  are
are  -vectors of real numbers such that
-vectors of real numbers such that  for some doubly stochastic matrix
for some doubly stochastic matrix  , then we say that
, then we say that  is (vector) majorized by
is (vector) majorized by  ; see [1]. Marshall and Olkin
; see [1]. Marshall and Olkin s text [2] is the standard general reference for majorization. Some kinds of majorization such as multivariate or matrix majorization were motivated by the concepts of vector majorization and were introduced in [3]. Let
s text [2] is the standard general reference for majorization. Some kinds of majorization such as multivariate or matrix majorization were motivated by the concepts of vector majorization and were introduced in [3]. Let  and
and  be two vector spaces over a field
be two vector spaces over a field  , and let
, and let  be a relation on both
be a relation on both  and
and  . We say that a linear map
. We say that a linear map  , preserves the relation
, preserves the relation  if
if

The problem of describing these preserving linear maps is one of the most studied linear preserver problems. A lot of effort has been done in [4–9] and [10–12] to characterize the structure of majorization preserving linear maps on certain spaces of matrices. A complex  matrix
matrix  is said to be g-row (or g-column) stochastic, if
is said to be g-row (or g-column) stochastic, if  (or
(or  ), where
), where  (or
(or  ). A complex
). A complex  matrix
matrix  is said to be g-doubly stochastic if it is both g-row and g-column stochastic. The notaions of generalized majorization (g-majorization) were motivated by the matrix majorization and were introduced in [4–6] as follows.
is said to be g-doubly stochastic if it is both g-row and g-column stochastic. The notaions of generalized majorization (g-majorization) were motivated by the matrix majorization and were introduced in [4–6] as follows.
Definition 1.1.
Let  and
and  be two vectors in
be two vectors in  . It is said that
. It is said that
(1) is gs-majorized by
is gs-majorized by  if there exists an
if there exists an  g-doubly stochastic matrix
g-doubly stochastic matrix  such that
such that  , and denoted by
, and denoted by  ;
;
(2) is lgw-majorized by
is lgw-majorized by  if there exists an
if there exists an  g-row stochastic matrix
g-row stochastic matrix  such that
such that  , and denoted by
, and denoted by  ;
;
(3) is rgw-majorized by
is rgw-majorized by  if there exists an
if there exists an  g-row stochastic matrix
g-row stochastic matrix  such that
such that  , and denoted by
, and denoted by  (here
(here  is the transpose of
is the transpose of  ).
).
Linear maps from  to
to  that preserve left matrix majorization or weak majorization were already characterized in [10, 11]. In this paper we characterize all linear maps preserving
that preserve left matrix majorization or weak majorization were already characterized in [10, 11]. In this paper we characterize all linear maps preserving  from
from  to
to  and all linear maps preserving
and all linear maps preserving  or
or  from
from  to
to  .
.
Throughout this paper, the standard bases of  and
and  are denoted by
are denoted by  and
and  , respectively. The notation
, respectively. The notation  is used for the sum of the components of a vector
is used for the sum of the components of a vector  or
or  . The vector space of all
. The vector space of all  complex matrices is denoted by
complex matrices is denoted by  . The notations
. The notations  and
and  are used for the
are used for the  matrix with rows
matrix with rows  and columns
and columns  . The sets of g-row and g-column stochastic
. The sets of g-row and g-column stochastic  matrices are denoted by
matrices are denoted by  and
and  , respectively. The set of g-doubly stochastic
, respectively. The set of g-doubly stochastic  matrices is denoted by
matrices is denoted by  . The symbol
. The symbol  is used for the
is used for the  matrix with all entries equal to one. The notation
matrix with all entries equal to one. The notation  is used for the matrix representation of the linear map
is used for the matrix representation of the linear map  with respect to the standard bases of
with respect to the standard bases of  and
and  where
where  .
.
2. Main Results
In this section we state some preliminary lemmas to describe the linear maps preserving  from
from  to
to  and the linear maps preserving
and the linear maps preserving  or
or  from
from  to
to  .
.
Lemma 2.1.
Let  be a linear map. Then
be a linear map. Then  preserves the subspace
preserves the subspace  if and only if
if and only if  .
.
Proof.
Let  . Assume that
. Assume that  for some
for some  . If
. If  and
and  , then
, then  , so
, so  preserves the subspace
preserves the subspace  . Conversely, assume that
. Conversely, assume that  preserves the subspace
preserves the subspace  . Then
. Then  for every
for every  . Therefore
. Therefore  where
where  for every
for every  .
.
The following lemma gives an equivalent condition for  on
on  .
.
Lemma 2.2 (see [4,  ]).
]).
Let  and let
and let  . Then
. Then  if and only if
if and only if  .
.
The following theorem characterizes all linear maps which preserve  from
from  to
to  . It is clear that every
. It is clear that every  preserves
preserves  , so assume that
, so assume that  .
.
Theorem 2.3.
A nonzero linear map  preserves
preserves  if and only if
if and only if  and
and  .
.
Proof.
Put  . Let
. Let  for some
for some  . If
. If  it is clear that
it is clear that  preserves
preserves  . If
. If  ,
,  and
and  then
then  and by Lemma 2.2,
and by Lemma 2.2,  . So
. So  and hence
and hence  by Lemma 2.1. Therefore
by Lemma 2.1. Therefore  by Lemma 2.2 and so
by Lemma 2.2 and so  preserves
preserves  . Now, we prove the necessity of the conditions. Let
. Now, we prove the necessity of the conditions. Let  be a linear preserver of
be a linear preserver of  . If
. If  , then
, then  by Lemma 2.2. So
by Lemma 2.2. So  and hence
and hence  by Lemma 2.2. Therefore
by Lemma 2.2. Therefore  preserves the subspace
preserves the subspace  and so
and so  by Lemma 2.1. If
by Lemma 2.1. If  , then there exists a nonzero vector
, then there exists a nonzero vector  such that
such that  . If
. If  then
then  for every
for every  , by Lemma 2.2. Then
, by Lemma 2.2. Then  for every
for every  and hence
and hence  which is a contradiction. Therefore
which is a contradiction. Therefore  and hence
and hence  for every
for every  , by Lemma 2.2. Then
, by Lemma 2.2. Then  and so
and so  for every
for every  . Put
. Put  . Thus
. Thus  and hence
and hence  .
.
We use the following lemmas to find the structure of linear preservers of lgw-majorization.
Remark 2.4 (see [7,  ]).
]).
If  , then
, then  , for all
, for all  .
.
Lemma 2.5.
Let  be a linear map. If
be a linear map. If  implies
implies  , then T preserves
, then T preserves  .
.
Proof.
Let  and
and  . If
. If  then
then  and it is clear that
and it is clear that  . If
. If  so
so  by the hypothesis and hence
by the hypothesis and hence  , by Remark 2.4. Therefore
, by Remark 2.4. Therefore  preserves
preserves  .
.
Lemma 2.6.
Let  be a nonzero singular linear map. Then
be a nonzero singular linear map. Then  preserves
preserves  if and only if
if and only if  and
and  .
.
Proof.
Let  be a linear preserver of
be a linear preserver of  . If
. If  and
and  , then
, then  and
and  , for all
, for all  by Remark 2.4. So
by Remark 2.4. So  , for all
, for all  , which is a contradiction. Therefore
, which is a contradiction. Therefore  and since
and since  ,
,  . If
. If  , then there exists
, then there exists  such that
such that  and
and  . Therefore
. Therefore  , for all
, for all  , and hence
, and hence  for all
for all  , which is a contradiction. So
, which is a contradiction. So  . The converse follows from Lemma 2.5.
. The converse follows from Lemma 2.5.
Proposition 2.7.
Let  be a nonzero linear preserver of
be a nonzero linear preserver of  . Then
. Then  .
.
Proof.
If  is injective, then
is injective, then  . If
. If  is not injective, we obtain
is not injective, we obtain  by Lemma 2.6 and
by Lemma 2.6 and  . Therefore
. Therefore  , by the rank and nullity theorem.
, by the rank and nullity theorem.
Theorem 2.8.
Let  be a nonzero linear map and
be a nonzero linear map and  . Then
. Then  preserves
preserves  if and only if one of the following holds:
if and only if one of the following holds:
(i) ,
,
(ii) and
and  .
.
Proof.
If (i) or (ii) holds, it is easy to show that  preserves
preserves  by Lemmas 2.5 and 2.6. Conversely, assume that
by Lemmas 2.5 and 2.6. Conversely, assume that  preserves
preserves  . If (i) does not hold, we show that (ii) holds. Since (i) does not hold, there exists a nonzero vector
. If (i) does not hold, we show that (ii) holds. Since (i) does not hold, there exists a nonzero vector  such that
such that  for some
for some  . If
. If  , then
, then  , for all
, for all  by Remark 2.4. So
by Remark 2.4. So  , for all
, for all  and hence
and hence  , which is a contradiction. Then
, which is a contradiction. Then  for some nonzero
for some nonzero  , and hence
, and hence  . Therefore,
. Therefore,  and
and  .
.
The following examples show that Proposition 2.7 does not hold for  or
or  .
.
Example 2.9.
For any positive integer  , the linear map
, the linear map  defined by
defined by  , preserves
, preserves  .
.
Example 2.10.
The linear map  defined by
defined by  , where
, where  , preserves rgw-majorization.
, preserves rgw-majorization.
We use the following statements to find the structure of linear preservers of gs-majorization.
Lemma 2.11 (see [6,  ]).
]).
Let x and y be two distinct vectors in  . Then
. Then  if and only if
if and only if  and
and  .
.
Lemma 2.12.
If a linear map  preserves
preserves  , then
, then  .
.
Proof.
Let  . For every
. For every  , it is clear that
, it is clear that  by Lemma 2.11. Then
by Lemma 2.11. Then  and hence there exists
and hence there exists  such that
such that  . So
. So  and therefore
and therefore  .
.
Theorem 2.13.
Let  be a linear map. Then
be a linear map. Then  preserves
preserves  if and only if one of the following holds:
if and only if one of the following holds:
(i)there exists some  such that
such that  ,
,  ,
,
(ii) for some
for some  and
and  ,
,
(iii) and
and 
Proof.
Let  . Assume that
. Assume that  preserves
preserves  . So
. So  by Lemma 2.12. Now, we consider two cases.
by Lemma 2.12. Now, we consider two cases.
Case 1  .
.
Suppose there exists  such that
such that  for some
for some  . If
. If  , then
, then  . So
. So  and hence
and hence  . For every
. For every  ,
,  by Lemma 2.11. Then
by Lemma 2.11. Then  and hence
and hence  , for all
, for all 
 . Then
. Then  , for some
, for some  and hence
and hence  for all
for all  . If
. If  , consider the basis
, consider the basis  for
for  . For every
. For every  ,
,  , by Lemma 2.11. Consequently
, by Lemma 2.11. Consequently  for every
for every  and hence
and hence  for all
for all  , where
, where  . Therefore, (i) holds in this case.
. Therefore, (i) holds in this case.
Case 2  .
.
Assume that  implies
implies  . Since
. Since  , we have
, we have  for every
for every  . Thus it follows that
. Thus it follows that  for every
for every  , where
, where  is the
is the  column of
column of  and hence
and hence  . If
. If  , then there exists
, then there exists  such that
such that  and hence
and hence  . By the hypothesis of this case,
. By the hypothesis of this case,  . Then (ii) holds. If
. Then (ii) holds. If  it is clear (iii) holds.
it is clear (iii) holds.
Conversly, if (i) or (iii) holds it is easy to show that  preserves gs-majorization. Suppose that (ii) holds. Then there exists
preserves gs-majorization. Suppose that (ii) holds. Then there exists  such that
such that  . Assume that
. Assume that  . If
. If  then
then  by Lemma 2.11. If
by Lemma 2.11. If  , then there exists
, then there exists  such that
such that  and hence
and hence  . Therefore,
. Therefore,  , and hence
, and hence  . Then
. Then  and hence
and hence  preserves gs-majorization.
preserves gs-majorization.
Corollary 2.14.
If  preserves
preserves  and
and  then
then  .
.
Proof.
If  is injective it is clear that
is injective it is clear that  . Assume that
. Assume that  is not injective, so there exists a nonzero vector
is not injective, so there exists a nonzero vector  such that
such that  . If
. If  , then by Case 1 in the proof of Theorem 2.13,
, then by Case 1 in the proof of Theorem 2.13,  for some
for some  . Therefore,
. Therefore,  , which is a contradiction. So
, which is a contradiction. So  and hence
and hence  . It is clear that
. It is clear that  , from which and the rank and nullity theorem, we obtain
, from which and the rank and nullity theorem, we obtain  , completing the proof.
, completing the proof.
References
Bhatia R: Matrix Analysis, Graduate Texts in Mathematics. Volume 169. Springer, New York, NY, USA; 1997:xii+347.
Marshall AW, Olkin I: Inequalities: Theory of Majorization and Its Applications. Academic Press, New York, NY, USA; 1972.
Dahl G: Matrix majorization. Linear Algebra and Its Applications 1999, 288(1–3):53–73.
Armandnejad A: Right gw-majorization on . Bulletin of the Iranian Mathematical Society 2009, 35(2):69–76.
Armandnejad A, Salemi A: Strong linear preservers of GW-majorization on . Journal of Dynamical Systems and Geometric Theories 2007, 5(2):165–168.
Armandnejad A, Salemi A: The structure of linear preservers of gs-majorization. Bulletin of the Iranian Mathematical Society 2006, 32(2):31–42.
Armandnejad A, Salemi A: On linear preservers of lgw-majorization on . to appear in Bulletin of the Malaysian Mathematical Society to appear in Bulletin of the Malaysian Mathematical Society
Armandnejad A, Afshin HR: Linear functions preserving multivariate and directional majorization. to appear in Iranian Journal of Mathematical Sciences and Informatics to appear in Iranian Journal of Mathematical Sciences and Informatics
Armandnejad A, Heydari H: Linear functions preserving gd-majorization from to . to appear in Bulletin of the Iranian Mathematical Society to appear in Bulletin of the Iranian Mathematical Society
Hasani AM, Vali MA: Linear maps which preserve or strongly preserve weak majorization. Journal of Inequalities and Applications 2007, 2007:-4.
Khalooei F, Salemi A: The structure of linear preservers of left matrix majorization on . Electronic Journal of Linear Algebra 2009, 18: 88–97.
Hasani AM, Radjabalipour M: The structure of linear operators strongly preserving majorizations of matrices. Electronic Journal of Linear Algebra 2006, 15: 260–268.
Acknowledgment
The authors would like to sincerely thank the referees for their constructive comments and suggestions which made some of the proofs simpler and clearer. This work has been supported by Vali-e-Asr university of Rafsanjan, grant no. 2740.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Armandnejad, A., Heydari, H. On Linear Maps Preserving g-Majorization from  to
to 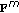 .
J Inequal Appl 2010, 254819 (2010). https://doi.org/10.1155/2010/254819
.
J Inequal Appl 2010, 254819 (2010). https://doi.org/10.1155/2010/254819
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1155/2010/254819