- Research
- Open access
- Published:
A more accurate half-discrete Hilbert-type inequality with a non-homogeneous kernel
Journal of Inequalities and Applications volume 2012, Article number: 292 (2012)
Abstract
By means of weight functions and the improved Euler-Maclaurin summation formula, a more accurate half-discrete Hilbert-type inequality with a non-homogeneous kernel and a best constant factor is given. A best extension, some equivalent forms, the operator expressions as well as some particular cases are also considered.
MSC:26D15, 47A07.
1 Introduction
Assuming that , , , we have the following Hilbert’s integral inequality (cf. [1]):
where the constant factor π is best possible. If , , , then we have the following analogous discrete Hilbert’s inequality:
with the same best constant factor π. Inequalities (1) and (2) are important in analysis and its applications (cf. [2–4]).
In 1998, by introducing an independent parameter , Yang [5] gave an extension of (1). For generalizing the results from [5], Yang [6] gave some best extensions of (1) and (2) as follows. If , , , is a non-negative homogeneous function of degree −λ satisfying , , , , , and , , then
where the constant factor is best possible. Moreover, if is finite and is decreasing for (), then for , , and , , , we have
where the constant is still the best value. Clearly, for , , , , (3) reduces to (1), while (4) reduces to (2).
Some other results about integral and discrete Hilbert-type inequalities can be found in [7–16]. On half-discrete Hilbert-type inequalities with the general non-homogeneous kernels, Hardy et al. provided a few results in Theorem 351 of [1]. But they did not prove that the constant factors are best possible. In 2005, Yang [17] gave a result with the kernel by introducing a variable and proved that the constant factor is best possible. Very recently, Yang [18] and [19] gave the following half-discrete Hilbert’s inequality with the best constant factor:
Chen [20] and Yang [21] gave two more accurate half-discrete Mulholland’s inequalities by using Hadamard’s inequality.
In this paper, by means of weight functions and the improved Euler-Maclaurin summation formula, a more accurate half-discrete Hilbert-type inequality with a non-homogeneous kernel and a best constant factor is given as follows. For , , ,

Moreover, a best extension of (6), some equivalent forms, the operator expressions as well as some particular inequalities are considered.
2 Some lemmas
Lemma 1 If , , (), () are decreasing continuous functions satisfying , , define a function as follows:
Then there exists such that

where is a Bernoulli function of the first order. In particular, for , , we have and
for , , if , then it follows and
Proof Define a decreasing continuous function as
Then it follows

Since , is a non-constant decreasing continuous function with , by the improved Euler-Maclaurin summation formula (cf. [6], Theorem 2.2.2), it follows
and then in view of the above results and by simple calculation, we have (7). □
Lemma 2 If , , , and and are weight functions given by


then we have
Proof Substituting in (10), and by simple calculation, we have
For fixed , we find

By the Euler-Maclaurin summation formula (cf. [6]), it follows

-
(i)
For , we obtain , and
Setting , wherefrom , and

then by (7), we find
In view of (11) and the above results, since for , namely , it follows
-
(ii)
For , we obtain , and
Since for , , by the improved Euler-Maclaurin summation formula (cf. [6]), it follows
In view of (13) and the above results, for , we find
Hence, for , we have , and then (12) follows. □
Lemma 3 Let the assumptions of Lemma 2 be fulfilled and, additionally, let , , , , be a non-negative measurable function in . Then we have the following inequalities:


Proof Setting , by Hölder’s inequality (cf. [22]) and (12), it follows

Then by the Lebesgue term-by-term integration theorem (cf. [23]), we have
Hence, (14) follows. By Hölder’s inequality again, we have
By the Lebesgue term-by-term integration theorem, we have
and in view of (12), inequality (15) follows. □
Lemma 4 Let the assumptions of Lemma 2 be fulfilled and, additionally, let , , . Setting , ; , , and , , then we have


Proof We find
and then (16) is valid. We obtain
and so (17) is valid. □
3 Main results
We introduce the functions
wherefrom and .
Theorem 5 If , , , , , , , , and , then we have the following equivalent inequalities:



where the constant is the best possible in the above inequalities.
Proof The two expressions for I in (18) follow from the Lebesgue term-by-term integration theorem. By (14) and (12), we have (19). By Hölder’s inequality, we have
Then by (19), we have (18). On the other hand, assume that (18) is valid. Setting
where . By (14), we find . If , then (19) is trivially valid; if , then by (18) we have
therefore ; that is, (19) is equivalent to (18). On the other hand, by (12) we have . Then in view of (15), we have (20). By Hölder’s inequality, we find
Then by (20), we have (18). On the other hand, assume that (18) is valid. Setting
then . By (15), we find . If , then (20) is trivially valid; if , then by (18), we have
therefore ; that is, (20) is equivalent to (18). Hence, (18), (19) and (20) are equivalent.
If there exists a positive number k () such that (18) is valid as we replace with k, then, in particular, it follows that . In view of (16) and (17), we have
and (). Hence, is the best value of (18).
By the equivalence of the inequalities, the constant factor in (19) and (20) is the best possible. □
Remark 1 (i) Define the first type half-discrete Hilbert-type operator as follows. For , we define by
Then by (19), and so is a bounded operator with . Since by Theorem 5 the constant factor in (19) is best possible, we have .
-
(ii)
Define the second type half-discrete Hilbert-type operator as follows. For , we define by
Then by (20), and so is a bounded operator with . Since by Theorem 5 the constant factor in (20) is best possible, we have .
Remark 2 (i) For , (18) reduces to (6). Since we find

then for in (18), we have the following inequality:

Hence, (18) is a more accurate inequality of (21).
-
(ii)
For in (18), we have , , , and
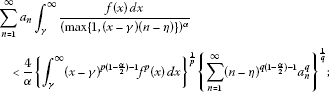 (22)
(22)
for in (18), we have , , , and

for in (18), we have , , , and

References
Hardy GH, Littlewood JE, Pólya G: Inequalities. Cambridge University Press, Cambridge; 1934.
Mitrinović DS, Pečarić JE, Fink AM: Inequalities Involving Functions and Their Integrals and Derivatives. Kluwer Academic, Boston; 1991.
Yang B: Hilbert-Type Integral Inequalities. Bentham Science Publishers, Sharjah; 2009.
Yang B: Discrete Hilbert-Type Inequalities. Bentham Science Publishers, Sharjah; 2011.
Yang B: On Hilbert’s integral inequality. J. Math. Anal. Appl. 1998, 220: 778–785. 10.1006/jmaa.1997.5877
Yang B: The Norm of Operator and Hilbert-Type Inequalities. Science Press, Beijing; 2009.
Yang B, Brnetić I, Krnić M, Pečarić J: Generalization of Hilbert and Hardy-Hilbert integral inequalities. Math. Inequal. Appl. 2005, 8(2):259–272.
Krnić M, Pečarić J: Hilbert’s inequalities and their reverses. Publ. Math. (Debr.) 2005, 67(3–4):315–331.
Jin J, Debnath L: On a Hilbert-type linear series operator and its applications. J. Math. Anal. Appl. 2010, 371: 691–704. 10.1016/j.jmaa.2010.06.002
Azar L: On some extensions of Hardy-Hilbert’s inequality and applications. J. Inequal. Appl. 2008., 2008: Article ID 546829
Yang B, Rassias T: On the way of weight coefficient and research for Hilbert-type inequalities. Math. Inequal. Appl. 2003, 6(4):625–658.
Arpad B, Choonghong O: Best constant for certain multilinear integral operator. J. Inequal. Appl. 2006., 2006: Article ID 28582
Kuang J, Debnath L: On Hilbert’s type inequalities on the weighted Orlicz spaces, pacific. J. Appl. Math. 2007, 1(1):95–103.
Zhong W: The Hilbert-type integral inequality with a homogeneous kernel of λ -degree. J. Inequal. Appl. 2008., 2008: Article ID 917392
Yang B: A new Hilbert-type operator and applications. Publ. Math. (Debr.) 2010, 76(1–2):147–156.
Li Y, He B: On inequalities of Hilbert’s type. Bull. Aust. Math. Soc. 2007, 76(1):1–13. 10.1017/S0004972700039423
Yang B: A mixed Hilbert-type inequality with a best constant factor. Int. J. Pure Appl. Math. 2005, 20(3):319–328.
Yang B: A half-discrete Hilbert’s inequality. J. Guangdong Educ. Inst. 2011, 31(3):1–7.
Yang B, Chen Q: A half-discrete Hilbert-type inequality with a homogeneous kernel and an extension. J. Inequal. Appl. 2011., 2011: Article ID 124. doi:10.1186/1029–242X-2011–124
Chen Q, Yang B: On a more accurate half-discrete Mulholland’s inequality and an extension. J. Inequal. Appl. 2012., 2012: Article ID 70. doi:10.1186/1029–242X-2012–70
Yang B: A new half-discrete Mulholland-type inequality with parameters. Ann. Funct. Anal. 2012, 3(1):142–150.
Kuang J: Applied Inequalities. Shangdong Science Technic Press, Jinan; 2004.
Kuang J: Introduction to Real Analysis. Hunan Education Press, Chansha; 1996.
Acknowledgements
This work is supported by Guangdong Natural Science Foundation (No. 7004344).
Author information
Authors and Affiliations
Corresponding author
Additional information
Competing interests
The authors declare that they have no competing interests.
Authors’ contributions
BY carried out the molecular genetic studies participated in the sequence alignment and drafted the manuscript. XL conceived of the study and participated in its design and coordination. All authors read and approved the final manuscript.
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Yang, B., Liu, X. A more accurate half-discrete Hilbert-type inequality with a non-homogeneous kernel. J Inequal Appl 2012, 292 (2012). https://doi.org/10.1186/1029-242X-2012-292
Received:
Accepted:
Published:
DOI: https://doi.org/10.1186/1029-242X-2012-292