- Research Article
- Open access
- Published:
Lyapunov Stability of Quasilinear Implicit Dynamic Equations on Time Scales
Journal of Inequalities and Applications volume 2011, Article number: 979705 (2011)
Abstract
This paper studies the stability of the solution  for a class of quasilinear implicit dynamic equations on time scales of the form
for a class of quasilinear implicit dynamic equations on time scales of the form  . We deal with an index concept to study the solvability and use Lyapunov functions as a tool to approach the stability problem.
. We deal with an index concept to study the solvability and use Lyapunov functions as a tool to approach the stability problem.
1. Introduction
The stability theory of quasilinear differential-algebraic equations (DAEs for short)

with  being a given
being a given  -matrix function, has been an intensively discussed field in both theory and practice. This problem can be seen in many real problems, such as in electric circuits, chemical reactions, and vehicle systems. März in [1] has dealt with the question whether the zero-solution of (1.1) is asymptotically stable in the Lyapunov sense with
-matrix function, has been an intensively discussed field in both theory and practice. This problem can be seen in many real problems, such as in electric circuits, chemical reactions, and vehicle systems. März in [1] has dealt with the question whether the zero-solution of (1.1) is asymptotically stable in the Lyapunov sense with  , with
, with  being constant and small perturbation
being constant and small perturbation  .
.
Together with the theory of DAEs, there has been a great interest in singular difference equation (SDE) (also referred to as descriptor systems, implicit difference equations)

This model appears in many practical areas, such as the Leontiev dynamic model of multisector economy, the Leslie population growth model, and singular discrete optimal control problems. On the other hand, SDEs occur in a natural way of using discretization techniques for solving DAEs and partial differential-algebraic equations, and so forth, which have already attracted much attention from researchers (cf. [2–4]). When  , in [5], the authors considered the solvability of Cauchy problem for (1.2); the question of stability of the zero-solution of (1.2) has been considered in [6] where the nonlinear perturbation
, in [5], the authors considered the solvability of Cauchy problem for (1.2); the question of stability of the zero-solution of (1.2) has been considered in [6] where the nonlinear perturbation  is small and does not depend on
is small and does not depend on  .
.
Further, in recent years, to unify the presentation of continuous and discrete analysis, a new theory was born and is more and more extensively concerned, that is, the theory of the analysis on time scales. The most popular examples of time scales are  and
and  . Using "language" of time scales, we rewrite (1.1) and (1.2) under a unified form
. Using "language" of time scales, we rewrite (1.1) and (1.2) under a unified form

with  in time scale
in time scale  and
and  being the derivative operator on
being the derivative operator on  . When
. When  , (1.3) is (1.1); if
, (1.3) is (1.1); if  , we have a similar equation to (1.2) if it is rewritten under the form
, we have a similar equation to (1.2) if it is rewritten under the form  .
.
The purpose of this paper is to answer the question whether results of stability for (1.1) and (1.2) can be extended and unified for the implicit dynamic equations of the form (1.3). The main tool to study the stability of this implicit dynamic equation is a generalized direct Lyapunov method, and the results of this paper can be considered as a generalization of (1.1) and (1.2).
The organization of this paper is as follows. In Section 2, we present shortly some basic notions of the analysis on time scales and give the solvability of Cauchy problem for quasilinear implicit dynamic equations

with small perturbation  and for quasilinear implicit dynamic equations of the style
and for quasilinear implicit dynamic equations of the style

with the assumption of differentiability for  . The main results of this paper are established in Section 3 where we deal with the stability of (1.5). The technique we use in this section is somewhat similar to the one in [6–8]. However, we need some improvements because of the complicated structure of every time scale.
. The main results of this paper are established in Section 3 where we deal with the stability of (1.5). The technique we use in this section is somewhat similar to the one in [6–8]. However, we need some improvements because of the complicated structure of every time scale.
2. Nonlinear Implicit Dynamic Equations on Time Scales
2.1. Some Basic Notations of the Theory of the Analysis on Time Scales
A time scale is a nonempty closed subset of the real numbers  , and we usually denote it by the symbol
, and we usually denote it by the symbol  . We assume throughout that a time scale
. We assume throughout that a time scale  is endowed with the topology inherited from the real numbers with the standard topology. We define the forward jump operator and the backward jump operator
is endowed with the topology inherited from the real numbers with the standard topology. We define the forward jump operator and the backward jump operator by
by  (supplemented by
(supplemented by  ) and
) and  (supplemented by
(supplemented by  ). The graininess
). The graininess is given by
is given by  . A point
. A point  is said to be right-dense if
is said to be right-dense if  , right-scattered if
, right-scattered if  , left-dense if
, left-dense if  , left-scattered if
, left-scattered if  , and isolated if
, and isolated if  is right-scattered and left-scattered. For every
is right-scattered and left-scattered. For every  , by
, by  , we mean the set
, we mean the set  . The set
. The set  is defined to be
is defined to be  if
if  does not have a left-scattered maximum; otherwise, it is
does not have a left-scattered maximum; otherwise, it is  without this left-scattered maximum. Let
without this left-scattered maximum. Let  be a function defined on
be a function defined on  , valued in
, valued in  . We say that
. We say that  is delta differentiable (or simply: differentiable) at
is delta differentiable (or simply: differentiable) at  provided there exists a vector
provided there exists a vector  , called the derivative of
, called the derivative of  , such that for all
, such that for all  there is a neighborhood
there is a neighborhood  around
around  with
with  for all
for all  . If
. If  is differentiable for every
is differentiable for every  , then
, then  is said to be differentiable on
is said to be differentiable on . If
. If  , then delta derivative is
, then delta derivative is  from continuous calculus; if
from continuous calculus; if  , the delta derivative is the forward difference,
, the delta derivative is the forward difference,  , from discrete calculus. A function
, from discrete calculus. A function  defined on
defined on  is rd-continuous if it is continuous at every right-dense point and if the left-sided limit exists at every left-dense point. The set of all rd-continuous functions from
is rd-continuous if it is continuous at every right-dense point and if the left-sided limit exists at every left-dense point. The set of all rd-continuous functions from  to a Banach space
to a Banach space  is denoted by
is denoted by  . A matrix function
. A matrix function  from
from  to
to  is said to be regressive if
is said to be regressive if  for all
for all  , and denote
, and denote  the set of regressive functions from
the set of regressive functions from  to
to  . Moreover, denote
. Moreover, denote  the set of positively regressive functions from
the set of positively regressive functions from  to
to  , that is, the set
, that is, the set  .
.
Let  and let
and let  be a rd-continuous
be a rd-continuous  -matrix function and
-matrix function and  rd-continuous function. Then, for any
rd-continuous function. Then, for any  , the initial value problem (IVP)
, the initial value problem (IVP)

has a unique solution  defined on
defined on  . Further, if
. Further, if  is regressive, this solution exists on
is regressive, this solution exists on  .
.
The solution of the corresponding matrix-valued IVP  ,
,  always exists for
always exists for  , even
, even  is not regressive. In this case,
is not regressive. In this case,  is defined only with
is defined only with  (see [12, 13]) and is called the Cauchy operator of the dynamic equation (2.1). If we suppose further that
(see [12, 13]) and is called the Cauchy operator of the dynamic equation (2.1). If we suppose further that  is regressive, the Cauchy operator
is regressive, the Cauchy operator  is defined for all
is defined for all  .
.
We now recall the chain rule for multivariable functions on time scales, this result has been proved in [14]. Let  and
and  be continuously differentiable. Then
be continuously differentiable. Then  is delta differentiable and there holds
is delta differentiable and there holds

where  is the derivative (in the second variable of the function
is the derivative (in the second variable of the function  ) in normal meaning and
) in normal meaning and  is the scalar product.
is the scalar product.
We refer to [12, 15] for more information on the analysis on time scales.
2.2. Linear Equations with Small Nonlinear Perturbation
Let  be a time scale. We consider a class of nonlinear equations of the form
be a time scale. We consider a class of nonlinear equations of the form

The homogeneous linear implicit dynamic equations (LIDEs) associated to (2.3) are

where  and
and  is rd-continuous in
is rd-continuous in  . In the case where the matrices
. In the case where the matrices  are invertible for every
are invertible for every  , we can multiply both sides of (2.3) by
, we can multiply both sides of (2.3) by  to obtain an ordinary dynamic equation
to obtain an ordinary dynamic equation

which has been well studied. If there is at least a  such that
such that  is singular, we cannot solve explicitly the leading term
is singular, we cannot solve explicitly the leading term  . In fact, we are concerned with a so-called ill-posed problem where the solutions of Cauchy problem may exist only on a submanifold or even they do not exist. One of the ways to solve this equation is to impose some further assumptions stated under the form of indices of the equation.
. In fact, we are concerned with a so-called ill-posed problem where the solutions of Cauchy problem may exist only on a submanifold or even they do not exist. One of the ways to solve this equation is to impose some further assumptions stated under the form of indices of the equation.
We introduce the so-called index-1 of (2.4). Suppose that rank  for all
for all  and let
and let  such that
such that  is an isomorphism between
is an isomorphism between  and
and  ;
;  . Let
. Let  be a projector onto
be a projector onto  satisfying
satisfying  . We can find such operators
. We can find such operators  and
and  by the following way: let matrix
by the following way: let matrix  possess a singular value decomposition
possess a singular value decomposition

where  ,
,  are orthogonal matrices and
are orthogonal matrices and  is a diagonal matrix with singular values
is a diagonal matrix with singular values  on its main diagonal. Since
on its main diagonal. Since  , on the above decomposition of
, on the above decomposition of  , we can choose the matrix
, we can choose the matrix  to be in
to be in  (see [16]). Hence, by putting
(see [16]). Hence, by putting  and
and  , we obtain
, we obtain  and
and  as the requirement.
as the requirement.
Let

and  .
.
Under these notations, we have the following Lemma.
Lemma 2.2.
The following assertions are equivalent
(i) ;
;
(ii)the matrix  is nonsingular;
is nonsingular;
(iii) , for all
, for all  .
.
Proof.
(i) (ii) Let
(ii) Let  and
and  such that
such that  . This equation implies
. This equation implies  . Since
. Since  and
and  , it follows that
, it follows that  . Hence,
. Hence,  which implies
which implies  . This means that
. This means that  . Thus,
. Thus,  , that is, the matrix
, that is, the matrix  is nonsingular.
is nonsingular.
(ii) (iii) It is obvious that
(iii) It is obvious that  . We see that
. We see that  and
and  . Thus,
. Thus,  and we have
and we have  .
.
Let  , that is,
, that is,  and
and  . Since
. Since  , there is a
, there is a  such that
such that  and since
and since  ,
,  . Therefore,
. Therefore,  . Hence,
. Hence,  which follows that
which follows that  . Thus,
. Thus,  and then
and then  . So, we have that (iii). (iii)
. So, we have that (iii). (iii) (i) is obvious.
(i) is obvious.
Lemma 2.2 is proved.
Lemma 2.3.
Suppose that the matrix  is nonsingular. Then, there hold the following assertions:
is nonsingular. Then, there hold the following assertions:






Proof.
-
(1)
Noting that
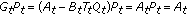 , we get (2.8).
, we get (2.8). -
(2)
From
 , it follows
, it follows 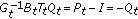 . Thus, we have (2.9).
. Thus, we have (2.9). -
(3)

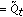 and
and 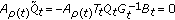 . This means that
. This means that  is a projector onto
is a projector onto 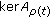 . From the proof of (iii), Lemma 2.2, we see that
. From the proof of (iii), Lemma 2.2, we see that 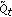 is the projector onto
is the projector onto  along
along 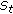 .
. -
(4)
Since
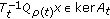 for any
for any  ,
, 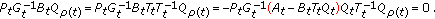 (2.14)
(2.14)Therefore,
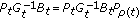 so we have (2.11). Finally,
so we have (2.11). Finally,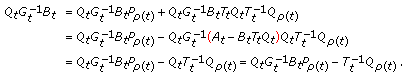 (2.15)
(2.15)Thus, we get (2.12).
-
(5)
Let
 be another linear transformation from
be another linear transformation from  onto
onto 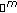 satisfying
satisfying 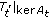 to be an isomorphism from
to be an isomorphism from 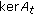 onto
onto  and
and  a projector onto
a projector onto 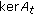 . Denote
. Denote 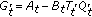 . It is easy to see that
. It is easy to see that 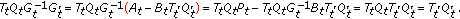 (2.16)
(2.16)
Therefore,  . The proof of Lemma 2.3 is complete.
. The proof of Lemma 2.3 is complete.
Definition 2.4.
The LIDE (2.4) is said to be index-1 if for all  , the following conditions hold:
, the following conditions hold:
(i)rank  ,
,
(ii) ker .
.
Now, we add the following assumptions.
Hypothesis 2.5.
-
(1)
The homogeneous LIDE (2.4) is of index-1.
-
(2)
 is rd-continuous and satisfies the Lipschitz condition,
is rd-continuous and satisfies the Lipschitz condition,  (2.17)
(2.17)
where

Remark 2.6.
By the item (2.13) of Lemma 2.3, the condition (2.18) is independent from the choice of  and
and  .
.
We assume further that we can choose the projector function  onto
onto  such that
such that  for all right-dense and left-scattered
for all right-dense and left-scattered  ;
;  is differentiable at every
is differentiable at every  and
and  is rd-continuous. For each
is rd-continuous. For each  , we have
, we have  . Therefore,
. Therefore,

and the implicit equation (2.3) can be rewritten as

Thus, we should look for solutions of (2.3) from the space  :
:

Note that  does not depend on the choice of the projector function since the relations
does not depend on the choice of the projector function since the relations  and
and  are true for each two projectors
are true for each two projectors  and
and  along the space
along the space  .
.
We now describe shortly the decomposition technique for (2.3) as follows.
Since (2.3) has index-1 and by virtue of Lemma 2.2, we see that the matrices  are nonsingular for all
are nonsingular for all  . Multiplying (2.3) by
. Multiplying (2.3) by  and
and  , respectively, it yields
, respectively, it yields

Therefore, by using the results of Lemma 2.3, we get

By denoting  , (2.23) becomes a dynamic equation on time scale
, (2.23) becomes a dynamic equation on time scale

and an algebraic relation

For fixed  and
and  , we consider a mapping
, we consider a mapping  given by
given by

We see that

for any  . Since
. Since  ,
,  is a contractive mapping. Hence, by the fixed point theorem, there exists a mapping
is a contractive mapping. Hence, by the fixed point theorem, there exists a mapping  satisfying
satisfying

and it is easy to see that  is rd-continuous in
is rd-continuous in  .
.
Moreover,

This deduces

Thus,  is Lipschitz continuous with the Lipschitz constant
is Lipschitz continuous with the Lipschitz constant  . Substituting
. Substituting  into (2.24), we obtain
into (2.24), we obtain

It is easy to see that the right-hand side of (2.31) satisfies the Lipschitz condition with the Lipschitz constant

Applying the global existence theorem (see [12]), we see that (2.31), with the initial condition  has a unique solution
has a unique solution  .
.
Thus, we get the following theorem.
Theorem 2.7.
Let Hypothesis 2.5 and the assumptions on the projector  be satisfied. Then, (2.3) with the initial condition
be satisfied. Then, (2.3) with the initial condition

has a unique solution. This solution is expressed by

where  is the solution of (2.31) with
is the solution of (2.31) with  .
.
We now describe the solution space of the implicit dynamic equation (2.3). Denote

Lemma 2.8.
There hold the following statements:
(i) ,
,
(ii)If  for all
for all  then
then  .
.
Proof.
(i) Let  , that is,
, that is,  . We have
. We have

Hence,

From

it yields

Conversely, suppose that  , that is, there exists
, that is, there exists  such that
such that  . We have to prove
. We have to prove

or equivalently,

Indeed,

where we have already used a result of Lemma 2.3 that  is a projector onto
is a projector onto  . So
. So  .
.
(ii) Let  . Then
. Then  and
and  . Since
. Since  , we have
, we have  . This means that
. This means that  . From the assumption
. From the assumption  , it follows that
, it follows that  . The fact
. The fact  implies that
implies that  . Thus
. Thus  . The lemma is proved.
. The lemma is proved.
Remark 2.9.
-
(1)
By virtue of Lemma 2.8, we find out that the solution space
 is independent from the choice of projector
is independent from the choice of projector  and operator
and operator  .
. -
(2)
Since
 and
and 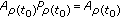 , the initial condition (2.33) is equivalent to the condition
, the initial condition (2.33) is equivalent to the condition . This implies that the initial condition is not also dependent on choice of projectors.
. This implies that the initial condition is not also dependent on choice of projectors. -
(3)
Noting that if
 is a solution of (2.3) with the initial condition (2.33), then
is a solution of (2.3) with the initial condition (2.33), then  for all
for all 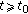 . Conversely, let
. Conversely, let  and let
and let 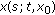 ,
, 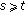 , be the solution of (2.3) satisfying the initial condition
, be the solution of (2.3) satisfying the initial condition  . We see that
. We see that  . This means that there exists a solution of (2.3) passing
. This means that there exists a solution of (2.3) passing  .
.
2.3. Quasilinear Implicit Dynamic Equations
Now we consider a quasilinear implicit dynamic equation of the form

with  and
and  assumed to be continuously differentiable in the variable
assumed to be continuously differentiable in the variable  and continuous in
and continuous in  .
.
Suppose that rank  for all
for all  . We keep all assumptions on the projector
. We keep all assumptions on the projector  and operator
and operator  stated in Section 2.2.
stated in Section 2.2.
Equation (2.43) is said to be of index-1 if the matrix

is invertible for every  and
and  .
.
Denote

Further introduce the set

containing all solutions of (2.43). The subspace  manifests its geometrical meaning
manifests its geometrical meaning

where  is the tangent space of
is the tangent space of  at the point
at the point  .
.
Suppose that (2.43) is of index-1. Then, by Lemma 2.2, this condition is equivalent to one of the following conditions:
(1) ,
,
(2) .
.
(3)Let  be a matrix such that the matrix
be a matrix such that the matrix  is invertible (we can choose
is invertible (we can choose  , e.g.). From the relation
, e.g.). From the relation

it follows that

is invertible.
Lemma 2.10.
Suppose that the bounded linear operator triplet:  is given, where
is given, where  are Banach spaces. Then the operator
are Banach spaces. Then the operator  is invertible if and only if
is invertible if and only if  is invertible.
is invertible.
Proof .
See [17, Lemma 1].
By virtue of (2.49) and Lemma 2.10, we get that

Now we come to split (2.43). Multiplying both sides of (2.43) by  and
and  , respectively, and putting
, respectively, and putting  , we obtain
, we obtain

Consider the function

We see that

where  .
.
Let  be a vector satisfying
be a vector satisfying  . Paying attention to
. Paying attention to  , we have
, we have

Therefore, by (2.50) we get  . This means that
. This means that  is an isomorphism of
is an isomorphism of  . By the implicit function theorem, equation
. By the implicit function theorem, equation  has a unique solution
has a unique solution  . Moreover, the function
. Moreover, the function  is continuous in
is continuous in  and continuously differentiable in
and continuously differentiable in  . Its derivative is
. Its derivative is

Then, by substituting  into the first equation of (2.51) we come to
into the first equation of (2.51) we come to

It is obvious that the ordinary dynamic equation (2.56) with the initial condition

is locally uniquely solvable and the solution  of (2.43) with the initial condition (2.33) can be expressed by
of (2.43) with the initial condition (2.33) can be expressed by  .
.
Now suppose further that  satisfies the Lipschitz condition in
satisfies the Lipschitz condition in  and we can find a matrix
and we can find a matrix  such that
such that

is bounded for all  and
and  . Then, the right-hand side of (2.56) also satisfies the Lipschitz condition. Thus, from the global existence theorem (see [12]), (2.56) with the initial condition (2.57) has a unique solution defined on
. Then, the right-hand side of (2.56) also satisfies the Lipschitz condition. Thus, from the global existence theorem (see [12]), (2.56) with the initial condition (2.57) has a unique solution defined on  .
.
Therefore, we have the following theorem.
Theorem 2.11.
Given an index-1 quasilinear implicit dynamic equation (2.43), then there holds the following.
-
(1)
Equation (2.43) is locally solvable, that is, for any
 ,
,  , there exists a unique solution
, there exists a unique solution 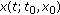 of (2.43), defined on
of (2.43), defined on 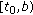 with some
with some  ,
, 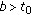 , satisfying the initial condition (2.33).
, satisfying the initial condition (2.33). -
(2)
Moreover, if
 satisfies the Lipschitz condition in
satisfies the Lipschitz condition in  and we can find a matrix
and we can find a matrix  such that
such that  (2.59)
(2.59)
is bounded, then this solution is defined on  and we have the expression
and we have the expression

where  is the solution of (2.56) with
is the solution of (2.56) with  .
.
Remark 2.12.
-
(1)
We note that the expression
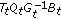 depends only on choosing the matrix
depends only on choosing the matrix 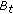 .
. -
(2)
The assumption that
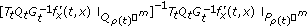 is bounded for a matrix function
is bounded for a matrix function  seems to be too strong. In Section 3, we show a condition for the global solvability via Lyapunov functions.
seems to be too strong. In Section 3, we show a condition for the global solvability via Lyapunov functions. -
(3)
If
 , there exists
, there exists  satisfying
satisfying 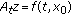 . Hence,
. Hence,  . Therefore, by the same argument as in Section 2.2, we can prove that for every
. Therefore, by the same argument as in Section 2.2, we can prove that for every  , there is a unique solution passing through
, there is a unique solution passing through 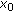 .
.
3. Stability Theorems of Implicit Dynamic Equations
For the reason of our purpose, in this section we suppose that  is an upper unbounded time scale, that is,
is an upper unbounded time scale, that is,  . For a fixed
. For a fixed  , denote
, denote  .
.
Consider an implicit dynamic equation of the form

where  and
and  .
.
First, we suppose that for each  , (3.1) with the initial condition
, (3.1) with the initial condition

has a unique solution defined on  . The condition ensuring the existence of a unique solution can be refered to Section 2. We denote the solution with the initial condition (3.2) by
. The condition ensuring the existence of a unique solution can be refered to Section 2. We denote the solution with the initial condition (3.2) by  . Remember that we look for the solution of (3.1) in the space
. Remember that we look for the solution of (3.1) in the space  . Let
. Let  for all
for all  , which follows that (3.1) has the trivial solution
, which follows that (3.1) has the trivial solution  .
.
We mention again that  . Noting that if
. Noting that if  is the solution of (3.1) and (3.2) then
is the solution of (3.1) and (3.2) then  for all
for all  .
.
Definition 3.1.
The trivial solution  of (3.1) is said to be
of (3.1) is said to be
(1) -stable (resp.,
-stable (resp.,  -stable) if, for each
-stable) if, for each  and
and  , there exists a positive
, there exists a positive  such that
such that  (resp.,
(resp.,  ) implies
) implies  for all
for all  ,
,
(2) -uniformly (resp.,
-uniformly (resp.,  -uniformly) stable if it is
-uniformly) stable if it is  -stable (resp.,
-stable (resp.,  -stable) and the number
-stable) and the number  mentioned in the part (1). of this definition is independent of
mentioned in the part (1). of this definition is independent of  ,
,
(3) -asymptotically (resp.,
-asymptotically (resp.,  -asymptotically) stable if it is stable and for each
-asymptotically) stable if it is stable and for each  , there exist positive
, there exist positive  such that the inequality
such that the inequality  (resp.,
(resp.,  ) implies
) implies  . If
. If  is independent of
is independent of  , then the corresponding stability is
, then the corresponding stability is  -uniformly asymptotically (
-uniformly asymptotically ( -uniformly asymptotically) stable,
-uniformly asymptotically) stable,
(4) -uniformly globally asymptotically (resp.,
-uniformly globally asymptotically (resp.,  -uniformly globally asymptotically) stable if for any
-uniformly globally asymptotically) stable if for any  there exist functions
there exist functions  ,
,  such that
such that  (resp.,
(resp.,  ) implies
) implies  for all
for all  and if
and if  (resp.,
(resp.,  ) then
) then  for all
for all  ,
,
(5)P-exponentially stable if there is positive constant  with
with  such that for every
such that for every  there exists an
there exists an  , the solution of (3.1) with the initial condition
, the solution of (3.1) with the initial condition  satisfies
satisfies  . If the constant
. If the constant  can be chosen independent of
can be chosen independent of  , then this solution is called
, then this solution is called  -uniformly exponentially stable.
-uniformly exponentially stable.
Remark 3.2.
From  and
and  , the notions of
, the notions of  -stable and
-stable and  -stable as well as
-stable as well as  -asymptotically stable and
-asymptotically stable and  -asymptotically stable are equivalent. Therefore, in the following theorems we will omit the prefixes
-asymptotically stable are equivalent. Therefore, in the following theorems we will omit the prefixes  and
and  when talking about stability and asymptotical stability. However, the concept of
when talking about stability and asymptotical stability. However, the concept of  -uniform stability implies
-uniform stability implies  -uniform stability if the matrices
-uniform stability if the matrices  are uniformly bounded and
are uniformly bounded and  -uniform stability implies
-uniform stability implies  -uniform stability if the matrices
-uniform stability if the matrices  are uniformly bounded.
are uniformly bounded.
Denote

and  is the domain of definition of
is the domain of definition of  .
.
Proposition 3.3.
The trivial solution  of (3.1) is
of (3.1) is  -uniformly (resp.,
-uniformly (resp.,  -uniformly) stable if and only if there exists a function
-uniformly) stable if and only if there exists a function  such that for each
such that for each  and any solution
and any solution  of (3.1) the inequality
of (3.1) the inequality

holds, provided  (resp.,
(resp.,  ).
).
Proof.
We only need to prove the proposition for the  -uniformly stable case.
-uniformly stable case.
Sufficiency. Suppose there exists a function  satisfying (3.4) for each
satisfying (3.4) for each  ; we take
; we take  such that
such that  , that is,
, that is,  . If
. If  is an arbitrary solution of (3.1) and
is an arbitrary solution of (3.1) and  , then
, then  , for all
, for all  .
.
Necessity. Suppose that the trivial solution  of (3.1) is
of (3.1) is  -uniformly stable, that is, for each
-uniformly stable, that is, for each  there exists
there exists  such that for each
such that for each  the inequality
the inequality  implies
implies  , for all
, for all  . For the sake of simplicity in computation, we choose
. For the sake of simplicity in computation, we choose  . Denote
. Denote

It is clear that  is an increasing positive function in
is an increasing positive function in  . Further,
. Further,  and by definition, there holds
and by definition, there holds

By putting

it is seen that

Let  be the inverse function of
be the inverse function of  . It is clear that
. It is clear that  also belongs to
also belongs to  .
.
For  , we denote
, we denote  . If
. If  , then
, then  by
by  (remember that
(remember that  does not imply that
does not imply that  ). Consider the case where
). Consider the case where  . If
. If  , then by the relations (3.6) and (3.8) we have
, then by the relations (3.6) and (3.8) we have  . In particular,
. In particular,  which is a contradiction. Thus
which is a contradiction. Thus  , this implies
, this implies  , provided
, provided  .
.
The proposition is proved.
Similarly, we have the following proposition.
Proposition 3.4.
The trivial solution  of (3.1) is
of (3.1) is  -stable (resp.,
-stable (resp.,  -stable) if and only if for each
-stable) if and only if for each  and any solution
and any solution  of (3.1) there exists a function
of (3.1) there exists a function  such that there holds the following:
such that there holds the following:

provided  (resp.,
(resp.,  ).
).
In order to use the Lyapunov function technique related to (3.1), we suppose that  . By using (2.3), we can define the derivative of the function
. By using (2.3), we can define the derivative of the function  along every solution curve as follows:
along every solution curve as follows:

Remark 3.5.
Note that when the function  is independent of
is independent of  and even if the vector field associated with the implicit dynamic equation (3.1) is autonomous, the derivative
and even if the vector field associated with the implicit dynamic equation (3.1) is autonomous, the derivative  may depend on
may depend on  .
.
Theorem 3.6.
Assume that there exist a constant  and a function
and a function  being rd-continuous and a function
being rd-continuous and a function  ,
,  defined on
defined on  satisfying
satisfying
(1) for all
for all  and
and  ,
,
(2) , for any
, for any  and
and  .
.
Assume further that (3.1) is locally solvable. Then, (3.1) is globally solvable, that is, every solution with the initial condition (3.2) is defined on  .
.
Proof.
Denote

By the condition (2), we have

Therefore, for all 

From the condition (1), it follows that

or

The last inequality says that the solution  can be lengthened on
can be lengthened on  , that is, (3.1) is globally solvable.
, that is, (3.1) is globally solvable.
Theorem 3.7.
Assume that there exist a function  being rd-continuous and a function
being rd-continuous and a function  ,
,  defined on
defined on  satisfying the conditions
satisfying the conditions
(1) for all
for all  ,
,
(2) for all
for all  and
and  ,
,
(3) for any
for any  and
and  .
.
Assume further that (3.1) is locally solvable. Then the trivial solution of (3.1) is stable.
Proof.
By virtue of Theorem 3.6 and the conditions (2) and (3), it follows that (3.1) is globally solvable. Suppose on the contrary that the trivial solution  of (3.1) is not stable. Then, there exists an
of (3.1) is not stable. Then, there exists an  such that for all
such that for all  there exists a solution
there exists a solution  of (3.1) satisfying
of (3.1) satisfying  and
and  for some
for some  . Put
. Put  .
.
By the assumption that  and
and  is rd-continuous, we can find
is rd-continuous, we can find  such that if
such that if  then
then  . With given
. With given  , let
, let  be a solution of (3.1) such that
be a solution of (3.1) such that  and
and  for some
for some  .
.
Since  and by the condition (3),
and by the condition (3),

Therefore,  . Further,
. Further,  and by the condition (2) we have
and by the condition (2) we have  . This is a contradiction. The theorem is proved.
. This is a contradiction. The theorem is proved.
Theorem 3.8.
Assume that there exist a function  being rd-continuous and functions
being rd-continuous and functions  ,
,  defined on
defined on  ,
,  such that
such that

satisfying the conditions
(1) uniformly in
uniformly in  ,
,
(2) for all
for all  and
and  ,
,
(3) for any
for any  and
and  .
.
Further, (3.1) is locally solvable. Then the trivial solution of (3.1) is asymptotically stable.
Proof.
Also from Theorem 3.6 and the conditions (2) and (3), it implies that (3.1) is globally solvable.
And since  , the trivial solution of (3.1) is stable by Theorem 3.7. Consider a bounded solution
, the trivial solution of (3.1) is stable by Theorem 3.7. Consider a bounded solution  of (3.1). First, we show that
of (3.1). First, we show that  Assume on the contrary that
Assume on the contrary that  . From the condition (1), it follows that
. From the condition (1), it follows that  By the condition (3), we have
By the condition (3), we have

as  , which gets a contradiction.
, which gets a contradiction.
Thus,  Further, from the condition (3) for any
Further, from the condition (3) for any  we get
we get

This means that  is a decreasing function. Consequently,
is a decreasing function. Consequently,

which follows that  by the condition (2).
by the condition (2).
Theorem 3.9.
Suppose that there exist a function  ,
,  defined on
defined on  , and a function
, and a function  such that
such that
(1) uniformly in
uniformly in  and
and  for all
for all  and
and  ,
,
(2) for any
for any  and
and  .
.
Assume further that (3.1) is locally solvable. Then, the trivial solution of (3.1) is  -uniformly stable.
-uniformly stable.
Proof.
The proof is similar to the one of Theorem 3.7 with a remark that since  uniformly in
uniformly in  , we can find
, we can find  such that if
such that if  then
then 
The proof is complete.
Remark 3.10.
The conclusion of Theorem 3.9 is still true if the condition (1) is replaced by "there exist two functions ,
, defined on
defined on and a function
and a function such that
such that for all
for all and
and ".
".
We present a theorem of uniform global asymptotical stability.
Theorem 3.11.
If there exist functions  ,
,  defined on
defined on  , and a function
, and a function  satisfying
satisfying
(1) for all
for all  and
and  ,
,
(2) for any
for any  and
and  .
.
Assume further that (3.1) is locally solvable. Then, the trivial solution of (3.1) is  -uniformly globally asymptotically stable.
-uniformly globally asymptotically stable.
Proof.
Let  be given. Define
be given. Define  and
and

( is not necessary in
is not necessary in  ).
).
Let  be a solution of (3.1) with
be a solution of (3.1) with  . From the condition (2), we see that
. From the condition (2), we see that

Therefore,

Hence,  for all
for all  .
.
Because the trivial solution of (3.1) is  -uniformly stable, we only need to show that there exists a
-uniformly stable, we only need to show that there exists a  such that
such that  . Assume that such a
. Assume that such a  does not exist, that is
does not exist, that is  for all
for all  . From the condition (2), we get
. From the condition (2), we get

Since  ,
,

which contradicts the definition of  in (3.21). The proof is complete.
in (3.21). The proof is complete.
When  is not differentiable, one supposes that there exists a
is not differentiable, one supposes that there exists a  -differentiable projector
-differentiable projector  onto
onto  and
and  is rd-continuous on
is rd-continuous on  ; moreover,
; moreover,  for all
for all  . Let
. Let  .
.
We choose matrix functions  such that
such that  is an isomorphism between
is an isomorphism between  and
and  and the matrix
and the matrix  is invertible. Define
is invertible. Define

where  (see (2.51)).
(see (2.51)).
From now on we remain following the above assumptions on the operators  whenever
whenever  is mentioned.
is mentioned.
By the same argument as Theorem 3.6, we have the following theorem.
Theorem 3.12.
Assume that there exist a constant  and a function
and a function  being rd-continuous and a function
being rd-continuous and a function  ,
,  defined on
defined on  satisfying
satisfying
(1) for all
for all  and
and  ,
,
(2) , for any
, for any  and
and  .
.
Assume further that (3.1) is locally solvable. Then, (3.1) is globally solvable.
Theorem 3.13.
Assume that (3.1) is locally solvable. Then, the trivial solution  of (3.1) is stable if there exist a function
of (3.1) is stable if there exist a function  being rd-continuous and a function
being rd-continuous and a function  ,
,  defined on
defined on  such that
such that
(1) for all
for all  ,
,
(2) for all
for all  and
and  ,
,
(3) for all
for all  and
and  .
.
Proof.
Assume that there is a function  satisfying the assertions (1), (2), and (3) but the trivial solution
satisfying the assertions (1), (2), and (3) but the trivial solution  of (3.1) is not stable. Then, there exist a positive
of (3.1) is not stable. Then, there exist a positive  and a
and a  such that
such that  ; there exists a solution
; there exists a solution  of (3.1) satisfying
of (3.1) satisfying  and
and  , for some
, for some  . Let
. Let  . Since
. Since  , it is possible to find a
, it is possible to find a  satisfying
satisfying  when
when  . Consider the solution
. Consider the solution  satisfying
satisfying  and
and  for a
for a  .
.
From the assumption (3), it follows that

This implies

We get a contradiction because  when
when  .
.
The proof of the theorem is complete.
Theorem 3.14.
Assume that (3.1) is locally solvable. If there exist two functions  ,
,  defined on
defined on  and a function
and a function  being rd-continuous such that
being rd-continuous such that
(1) for all
for all  and
and  ,
,
(2) for all
for all  and
and  ,
,
then the trivial solution of (3.1) is  -uniformly stable.
-uniformly stable.
Proof.
The proof is similar to the one of Theorem 3.9.
Theorem 3.15.
If there exist functions  ,
,  defined on
defined on  and a function
and a function  satisfying
satisfying
(1) for all
for all  and
and  ,
,
(2) for any
for any  and
and  .
.
Assume further that (3.1) is locally solvable. Then, the trivial solution of (3.1) is  -uniformly globally asymptotically stable.
-uniformly globally asymptotically stable.
Proof.
Similarly to the proof of Theorem 3.11.
It is difficult to establish the inverse theorem for Theorems from 3.7 to 3.15, that is, if the trivial solution of (3.1) is stable, there exists a function  satisfying the assertions in the above theorems. However, if the structure of the time scale
satisfying the assertions in the above theorems. However, if the structure of the time scale  is rather simple we have the following theorem.
is rather simple we have the following theorem.
Theorem 3.16.
Suppose that  contains no right-dense points and the trivial solution
contains no right-dense points and the trivial solution  of (3.1) is
of (3.1) is  -uniformly stable. Then, there exists a function
-uniformly stable. Then, there exists a function  being rd-continuous satisfying the conditions (1), (2), and (3) of Theorem 3.13, where
being rd-continuous satisfying the conditions (1), (2), and (3) of Theorem 3.13, where  is an open neighborhood of 0 in
is an open neighborhood of 0 in  .
.
Proof.
Suppose the trivial solution of (3.1) is  -uniformly stable. Due to Proposition 3.3, there exist functions
-uniformly stable. Due to Proposition 3.3, there exist functions  such that for any solution
such that for any solution  of (3.1), we have
of (3.1), we have

provided  .
.
Let  and
and  . For any
. For any  satisfying
satisfying  and
and  , we put
, we put

where  is the unique solution of (3.1) satisfying the initial condition
is the unique solution of (3.1) satisfying the initial condition  . It is seen that
. It is seen that  is defined for all
is defined for all  satisfying
satisfying  ,
,  , and
, and  .
.
Let  . By the definition,
. By the definition,  . From (2.60),
. From (2.60),  for all
for all  . In particular,
. In particular,  . Thus,
. Thus,  . Hence, we have the assertion (2) of the theorem.
. Hence, we have the assertion (2) of the theorem.
Due to the unique solvability of (3.1), we have  with
with  . Therefore,
. Therefore,  and
and

This implies

The proof is complete.
Now we give an example on using Lyapunov functions to test the stability of equations. The following result finds out that the stability of a linear equation will be ensured if nonlinear perturbations are sufficiently small Lipschitz.
Consider a nonlinear equation of the form (2.3)

where  and
and  are constant matrices with ind
are constant matrices with ind  ,
,  , and
, and  satisfing the Lipschitz condition
satisfing the Lipschitz condition

where  is sufficiently small. Let
is sufficiently small. Let  be defined by (2.9) with
be defined by (2.9) with  and
and  . By Theorem 2.7, we see that there exists a unique solution satisfying the condition
. By Theorem 2.7, we see that there exists a unique solution satisfying the condition  for any
for any  .
.
Besides, also consider the homogeneous equation associated to (3.33)

and suppose this equation has index-1. As in Section 2, multiplying (3.33) by  we get
we get

where  .
.
Note that the general solution of (3.35) is

in there  is denoted the set of right-scattered points of the interval
is denoted the set of right-scattered points of the interval  .
.
Denote  . It is easy to show that the trivial solution
. It is easy to show that the trivial solution  of (3.35) is
of (3.35) is  -uniformly exponentially stable if and only if
-uniformly exponentially stable if and only if  , where
, where  is the domain of uniform exponential stability of
is the domain of uniform exponential stability of  . On the exponential stable domain of a time scale, we can refer to [10, 18, 19]. By the definition of exponential stability, it implies that the graininess function of the time scale
. On the exponential stable domain of a time scale, we can refer to [10, 18, 19]. By the definition of exponential stability, it implies that the graininess function of the time scale  is upper bounded. Let
is upper bounded. Let  .
.
We denote the set

and suppose  . Since
. Since  , this condition implies that (3.35) is
, this condition implies that (3.35) is  -uniformly exponentially stable.
-uniformly exponentially stable.
If  , define
, define

where the matrix  is supposed to be symmetric positive definite. It is clear that
is supposed to be symmetric positive definite. It is clear that  is symmetric positive definite.
is symmetric positive definite.
Since  , the above series is convergent. Further, for any
, the above series is convergent. Further, for any  we have
we have

Thus,

Letting  and paying attention to
and paying attention to  , we obtain
, we obtain

In the case where  and
and  is symmetric positive definite, by putting
is symmetric positive definite, by putting

we can examine easily that the matrix  also satisfies (3.42),
also satisfies (3.42),  is symmetric and positive definite.
is symmetric and positive definite.
Theorem 3.17.
Suppose that  and the homogeneous equation (3.35) is of index-1 and the constant
and the homogeneous equation (3.35) is of index-1 and the constant  is sufficiently small. Then, the trivial solution
is sufficiently small. Then, the trivial solution  of (3.33) is
of (3.33) is  -uniformly globally asymptotically stable.
-uniformly globally asymptotically stable.
Proof.
Let  be a symmetric and positive definite (constant) matrix satisfying (3.42). Consider the Lyapunov function
be a symmetric and positive definite (constant) matrix satisfying (3.42). Consider the Lyapunov function  . The derivative of
. The derivative of  along the solution of (3.33) is
along the solution of (3.33) is

From the Lipschitz condition and (2.25), it is seen that  where
where  . Therefore,
. Therefore,

Combining this inequality and the above appreciation, we see that when  is sufficiently small there exists
is sufficiently small there exists  such that
such that

By Theorem 3.15, (3.33) is  -uniformly globally asymptotically stable.
-uniformly globally asymptotically stable.
Example 3.18.
Let  and consider
and consider

with

We have  span
span , rank
, rank  for all
for all  . It is easy to verify that
. It is easy to verify that  is the canonical projector onto
is the canonical projector onto  ,
,  . Let us choose
. Let us choose  . We see that
. We see that

Since  ,
,  , (3.47) has index-1.
, (3.47) has index-1.
It is obvious that  . Further,
. Further,  . Thus, according to Theorem 2.7 for each
. Thus, according to Theorem 2.7 for each  , (3.47) with the initial condition
, (3.47) with the initial condition  has the unique solution.
has the unique solution.
It is easy to compute,  ,
,  ,
,  , where
, where  , and
, and  .
.
Therefore,  satisfies
satisfies  . Moreover, we have
. Moreover, we have

Let the Lyapunov function be  .
.
Put  , we have
, we have  and
and

Hence,

We have for any solution  of (3.47) and
of (3.47) and  (noting that
(noting that  ),
),
(+) if  is right-scattered then
is right-scattered then 
 ,
,
(+)if  is right-dense then
is right-dense then  , where
, where  .
.
In both two cases, we have  , so the trivial solution of (3.47) is
, so the trivial solution of (3.47) is  -uniformly stable by Theorem 3.14.
-uniformly stable by Theorem 3.14.
Note that if we let  then the result is still true. Indeed, by the simple calculations we obtain
then the result is still true. Indeed, by the simple calculations we obtain
(a) , where
, where  defined by
defined by  ,
,
(b) . Thus,
. Thus,

Therefore, having the above result is obvious.
4. Conclusion
We have studied some criteria ensuring the stability for a class of quasilinear dynamic equations on time scales. So far, the inverse theorem of the theorems of the stability in Section 3 of this paper is still an open problem for an arbitrary time scale meanwhile it is true for discrete and continuous time scales.
References
März R: Criteria for the trivial solution of differential algebraic equations with small nonlinearities to be asymptotically stable. Journal of Mathematical Analysis and Applications 1998,225(2):587–607. 10.1006/jmaa.1998.6055
Campbell SL: Singular Systems of Differential Equations I, II, Research Notes in Mathematics. Volume 40. Pitman, London, UK; 1980:vii+176.
Griepentrog E, März R: Differential-Algebraic Equations and Their Numerical Treatment. Volume 88. Teubner, Leipzig, Germany; 1986:220.
Kunkel P, Mehrmann V: Differential-Algebraic Equations, EMS Textbooks in Mathematics. European Mathematical Society House, Zürich, Switzerland; 2006:viii+377.
Loi LC, Du NH, Anh PK: On linear implicit non-autonomous systems of difference equations. Journal of Difference Equations and Applications 2002,8(12):1085–1105. 10.1080/1023619021000053962
Anh PK, Hoang DS: Stability of a class of singular difference equations. International Journal of Difference Equations 2006,1(2):181–193.
Kloeden PE, Zmorzynska A: Lyapunov functions for linear nonautonomous dynamical equations on time scales. Advances in Difference Equations 2006, 2006: 1–10.
Lakshmikantham V, Sivasundaram S, Kaymakcalan B: Dynamic Systems on Measure Chains, Mathematics and its Applications. Volume 370. Kluwer Academic, Dordrecht, The Netherlands; 1996:x+285.
Advances in Dynamic Equations on Time Scales. Birkhäuse, Boston, Mass, USA; 2003:xii+348.
Bohner M, Stević S: Linear perturbations of a nonoscillatory second-order dynamic equation. Journal of Difference Equations and Applications 2009,15(11–12):1211–1221. 10.1080/10236190903022782
Bohner M, Stević S: Trench's perturbation theorem for dynamic equations. Discrete Dynamics in Nature and Society 2007, 2007:-11.
Hilger S: Analysis on measure chains—a unified approach to continuous and discrete calculus. Results in Mathematics 1990,18(1–2):18–56.
Pötzsche C: Exponential dichotomies of linear dynamic equations on measure chains under slowly varying coefficients. Journal of Mathematical Analysis and Applications 2004,289(1):317–335. 10.1016/j.jmaa.2003.09.063
Pötzsche C: Chain rule and invariance principle on measure chains. Journal of Computational and Applied Mathematics 2002,141(1–2):249–254. 10.1016/S0377-0427(01)00450-2
Pötzsche C: Analysis Auf Maßketten. Universität Augsburg; 2002.
Dai L: Singular Control Systems, Lecture Notes in Control and Information Sciences. Volume 118. Springer, Berlin, Germany; 1989:x+332.
Du NH, Linh VH: Stability radii for linear time-varying differential-algebraic equations with respect to dynamic perturbations. Journal of Differential Equations 2006,230(2):579–599. 10.1016/j.jde.2006.07.004
Doan TS, Kalauch A, Siegmund S, Wirth FR: Stability radii for positive linear time-invariant systems on time scales. Systems & Control Letters 2010,59(3–4):173–179. 10.1016/j.sysconle.2010.01.002
Pötzsche C, Siegmund S, Wirth F: A spectral characterization of exponential stability for linear time-invariant systems on time scales. Discrete and Continuous Dynamical Systems 2003,9(5):1223–1241.
Acknowledgment
This work was done under the support of NAFOSTED no 101.02.63.09.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Du, N., Liem, N., Chyan, C. et al. Lyapunov Stability of Quasilinear Implicit Dynamic Equations on Time Scales. J Inequal Appl 2011, 979705 (2011). https://doi.org/10.1155/2011/979705
Received:
Accepted:
Published:
DOI: https://doi.org/10.1155/2011/979705
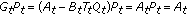 , we get (2.8).
, we get (2.8). , it follows
, it follows 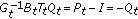 . Thus, we have (2.9).
. Thus, we have (2.9).
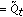 and
and 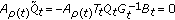 . This means that
. This means that  is a projector onto
is a projector onto 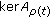 . From the proof of (iii), Lemma 2.2, we see that
. From the proof of (iii), Lemma 2.2, we see that 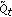 is the projector onto
is the projector onto  along
along 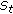 .
.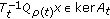 for any
for any  ,
, 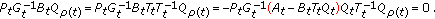
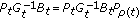 so we have (2.11). Finally,
so we have (2.11). Finally,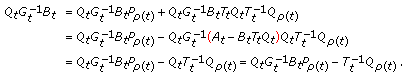
 be another linear transformation from
be another linear transformation from  onto
onto 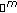 satisfying
satisfying 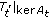 to be an isomorphism from
to be an isomorphism from 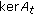 onto
onto  and
and  a projector onto
a projector onto 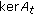 . Denote
. Denote 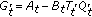 . It is easy to see that
. It is easy to see that 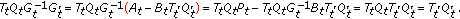
 is rd-continuous and satisfies the Lipschitz condition,
is rd-continuous and satisfies the Lipschitz condition, 
 is independent from the choice of projector
is independent from the choice of projector  and operator
and operator  .
. and
and 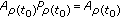 , the initial condition (2.33) is equivalent to the condition
, the initial condition (2.33) is equivalent to the condition . This implies that the initial condition is not also dependent on choice of projectors.
. This implies that the initial condition is not also dependent on choice of projectors. is a solution of (2.3) with the initial condition (2.33), then
is a solution of (2.3) with the initial condition (2.33), then  for all
for all 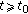 . Conversely, let
. Conversely, let  and let
and let 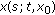 ,
, 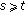 , be the solution of (2.3) satisfying the initial condition
, be the solution of (2.3) satisfying the initial condition  . We see that
. We see that  . This means that there exists a solution of (2.3) passing
. This means that there exists a solution of (2.3) passing  .
. ,
,  , there exists a unique solution
, there exists a unique solution 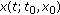 of (2.43), defined on
of (2.43), defined on 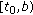 with some
with some  ,
, 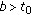 , satisfying the initial condition (2.33).
, satisfying the initial condition (2.33). satisfies the Lipschitz condition in
satisfies the Lipschitz condition in  and we can find a matrix
and we can find a matrix  such that
such that 
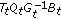 depends only on choosing the matrix
depends only on choosing the matrix 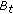 .
.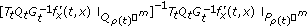 is bounded for a matrix function
is bounded for a matrix function  seems to be too strong. In Section 3, we show a condition for the global solvability via Lyapunov functions.
seems to be too strong. In Section 3, we show a condition for the global solvability via Lyapunov functions. , there exists
, there exists  satisfying
satisfying 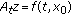 . Hence,
. Hence,  . Therefore, by the same argument as in Section 2.2, we can prove that for every
. Therefore, by the same argument as in Section 2.2, we can prove that for every  , there is a unique solution passing through
, there is a unique solution passing through 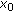 .
.