- Research Article
- Open access
- Published:
Positive Semidefinite Matrices, Exponential Convexity for Majorization, and Related Cauchy Means
Journal of Inequalities and Applications volume 2010, Article number: 728251 (2010)
Abstract
We prove positive semidefiniteness of matrices generated by differences deduced from majorization-type results which implies exponential convexity and log-convexity of these differences and also obtain Lyapunov's and Dresher's inequalities for these differences. We introduce new Cauchy means and show that these means are monotone.
1. Introduction and Preliminaries
Let  ,
,  denote two sequences of positive real numbers with
denote two sequences of positive real numbers with  . The well-known Jensen inequality for convex function [1, page 43] gives that, for
. The well-known Jensen inequality for convex function [1, page 43] gives that, for  or
or  ,
,

and vice versa for  .
.
In [2], the following generalization of this theorem is given.
Theorem 1.1.
For  ,
,

where

For fixed  let
let

denote two  -tuples. Let
-tuples. Let

be their ordered components.
Definition 1.2 (see [1, page 319]).

is said to majorize  (or
(or  is said to be majorized by
is said to be majorized by  ), in symbol,
), in symbol,  , if
, if

holds for  and
and

Note that (1.6) is equivalent to

for  .
.
The following theorem is well-known as the majorization theorem and a convenient reference for its proof is given by Marshall and Olkin [3, page11] (see also [1, page 320]).
Theorem 1.3.
Let  be an interval in
be an interval in  , and let
, and let  be two
be two  -tuples such that
-tuples such that  ,
,  . Then
. Then

holds for every continuous convex function  if and only if
if and only if  holds.
holds.
Remark 1.4 (see [4]).
If  is a strictly convex function, then equality in (1.9) is valid iff
is a strictly convex function, then equality in (1.9) is valid iff  .
.
The following theorem can be regarded as a generalization of Theorem 1.3 and is proved by Fuchs in [5] (see also [1, page 323]).
Theorem 1.5.
Let  be two decreasing real n-tuples, and let
be two decreasing real n-tuples, and let  be a real
be a real  -tuple such that
-tuple such that

Then for every continuous convex function  , one has
, one has

Definition 1.6.
A function  is exponentially convex function if it is continuous and
is exponentially convex function if it is continuous and

for all  and all choices
and all choices  and
and  ,
,  such that
such that  ,
,  .
.
The following proposition is given in [6].
Proposition 1.7.
Let  . The following propositions are equivalent.
. The following propositions are equivalent.
(i) is exponentially convex.
is exponentially convex.
(ii) is continuous and
is continuous and

for every  , every
, every  , and every
, and every  ,
,  .
.
Corollary 1.8.
If  is exponentially convex, then
is exponentially convex, then

for every  and every
and every  ,
,  .
.
Corollary 1.9.
If  is exponentially convex function, then
is exponentially convex function, then  is a
is a  -convex function in Jensens sense:
-convex function in Jensens sense:

In this paper, we prove positive semidefiniteness of matrices generated by differences deduced from majorization-type results (1.9), (1.11), (4.2), and (4.5) which implies exponential convexity and log-convexity of these differences and also obtain Lyapunov's and Dresher's inequalities for these differences. In [7], new Cauchy means are introduced. By using these means, a generalization of (1.2) was given (see [7]). In the present paper, we give related results in discrete and indiscrete cases and some new means of the Cauchy type.
2. Main Results
Lemma 2.1.
Define the function

Then  , that is,
, that is,  is convex for
is convex for 
Definition 2.2.
It is said that a positive function  is
is  -convex in the Jensen sense on some interval
-convex in the Jensen sense on some interval  if
if

holds for every  .
.
The following lemma gives an equivalent condition for convexity of function  [1, page 2].
[1, page 2].
Lemma 2.3.
If  is convex on an interval
is convex on an interval  , then
, then

holds for every  ,
,  .
.
Theorem 2.4.
Let  and
and  be two positive
be two positive  -tuples,
-tuples,  ,
,

and all  's and
's and  's are not equal.
's are not equal.
Then the following statements are valid.
(a)For every  and
and  , the matrix
, the matrix  is a positive semidefinite matrix. Particularly
is a positive semidefinite matrix. Particularly

for 
(b)The function  is exponentially convex.
is exponentially convex.
(c)The function  is
is  -convex on
-convex on  and the following inequality holds for
and the following inequality holds for  :
:

Proof.
-
(a)
Consider the function
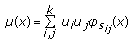 (2.7)
(2.7)
for  ,
,  ,
,  ,
,  , where
, where  and
and  is defined in (2.1).
is defined in (2.1).
We have

This shows that  is a convex function for
is a convex function for  .
.
Using Theorem 1.3,

This implies that

or equivalently

From last inequality, it follows that the matrix  is a positive semidefinite matrix, that is, (2.5) is valid.
is a positive semidefinite matrix, that is, (2.5) is valid.
-
(b)
Note that
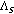 is continuous for
is continuous for  . Then by using Proposition 1.7, we get exponentially convexity of the function
. Then by using Proposition 1.7, we get exponentially convexity of the function 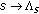 .
. -
(c)
Since
 is continuous and strictly convex function for
is continuous and strictly convex function for 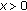 and all
and all  's and
's and 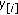 's are not equal, therefore by Theorem 1.3 with
's are not equal, therefore by Theorem 1.3 with  we have
we have

This implies

that is,  is a positive-valued function.
is a positive-valued function.
A simple consequence of Corollary 1.9 is that  is
is  -convex; then by definition
-convex; then by definition

which is equivalent to (2.6).
Theorem 2.5.
Let  be defined as in Theorem 2.4 and
be defined as in Theorem 2.4 and  such that
such that  ,
,  ,
,  , and
, and  . Then
. Then

Proof.
For a convex function  , a simple consequence of (2.3) is the following inequality [1, page 2]:
, a simple consequence of (2.3) is the following inequality [1, page 2]:

with  . Since by Theorem 2.4(c) and
. Since by Theorem 2.4(c) and  is
is  -convex, we can set in (2.16)
-convex, we can set in (2.16)  , and
, and  . We get
. We get

from which (2.15) follows.
Theorem 2.6.
Let  and
and  be two positive decreasing
be two positive decreasing  -tuples, let
-tuples, let  be a real
be a real  -tuple and let
-tuple and let

such that conditions (1.10) are satisfied and  is positive.
is positive.
Then the following statements are valid.
(a)For every  and
and  , the matrix
, the matrix  is a positive semidefinite matrix. Particularly
is a positive semidefinite matrix. Particularly

for 
(b)The function  is exponentially convex.
is exponentially convex.
(c)The function  is
is  -convex on
-convex on  and the following inequality holds for
and the following inequality holds for  :
:

Proof.
As in the proof of Theorem 2.4, we use Theorem 1.5 instead of Theorem 1.3.
Theorem 2.7.
Let  be defined as in Theorem 2.6 and
be defined as in Theorem 2.6 and  such that
such that  ,
,  ,
,  , and
, and  . Then
. Then

Proof.
Similar to the proof of Theorem 2.5.
3. Cauchy Means
Let us note that (2.15) and (2.21) have the form of some known inequalities between means (e.g., Stolarsky means, Gini means, etc). Here we will prove that expressions on both sides of (2.15) and (2.21) are also means.
Lemma 3.1.
Let  , I interval in
, I interval in  , be such that
, be such that

Consider the functions  ,
,  defined as
defined as

then  for
for  are convex.
are convex.
Proof.
Since

that is,  for
for  are convex.
are convex.
Denote

In the above expression,  and
and  are the minimums of
are the minimums of  and
and  , respectively. Similarly,
, respectively. Similarly,  and
and  are the maximums of
are the maximums of  and
and  respectively.
respectively.
Theorem 3.2.
Let  and
and  be two positive
be two positive  -tuples,
-tuples,  , all
, all  's and
's and  's are not equal, and
's are not equal, and  with
with  being defined as in (3.4), then there exists
being defined as in (3.4), then there exists  such that
such that

Proof.
Since  and
and  is compact, then
is compact, then  for
for  Then by applying
Then by applying  and
and  defined in Lemma 3.1 for
defined in Lemma 3.1 for  in Theorem 1.3, we have
in Theorem 1.3, we have

that is,


By combining (3.7) and (3.8)


because  , for
, for  by using Remark 1.4. Using the fact that for
by using Remark 1.4. Using the fact that for  , there exists
, there exists  such that
such that  , we get (3.5).
, we get (3.5).
Theorem 3.3.
Let  and
and  be two positive
be two positive  -tuples,
-tuples,  , all
, all  's and
's and  's are not equa,and
's are not equa,and  , with
, with  being defined as in (3.4), then there exists
being defined as in (3.4), then there exists  such that
such that

provided that  for every
for every  .
.
Proof.
Let a function  be defined as
be defined as

where  and
and  are defined as
are defined as

Then, using Theorem 3.2 with  , we have
, we have

Since

therefore, (3.13) gives

After putting values, we get (3.10). The denominator of left-hand side is nonzero by using  in Theorem 3.2.
in Theorem 3.2.
Corollary 3.4.
Let  and
and  be two positive
be two positive  -tuples such that
-tuples such that  and all
and all  's and
's and  's are not equal, then for
's are not equal, then for  there exists
there exists  , with
, with  being defined as in (3.4), such that
being defined as in (3.4), such that

Proof.
Setting  and
and  ,
,  in (3.10), we get (3.16).
in (3.10), we get (3.16).
Remark 3.5.
Since the function  is invertible, then from (3.16) we have
is invertible, then from (3.16) we have

In fact, similar result can also be given for (3.10). Namely, suppose that  has inverse function. Then from (3.10), we have
has inverse function. Then from (3.10), we have

So, we have that the expression on the right-hand side of (3.18) is also a mean. By the inequality (3.17), we can consider for positive  -tuples
-tuples  and
and  such that
such that  ,
,

for  , as means in broader sense. Moreover we can extend these means in other cases. So passing to the limit, we have
, as means in broader sense. Moreover we can extend these means in other cases. So passing to the limit, we have

Theorem 3.6.
Let  such that
such that  ,
,  , then the following inequality is valid:
, then the following inequality is valid:

Proof.
Since  is log-convex, therefore by (2.15) we get (3.21)
is log-convex, therefore by (2.15) we get (3.21)
Theorem 3.7.
Let  and
and  be two positive decreasing
be two positive decreasing  -tuples, let
-tuples, let  be a real
be a real  -tuple such that conditions (1.10) are satisfied,
-tuple such that conditions (1.10) are satisfied,  is positive defined as in Theorem 2.6, and
is positive defined as in Theorem 2.6, and  with
with  being defined as in (3.4), then there exists
being defined as in (3.4), then there exists  such that
such that

Proof.
As in the proof of Theorem 3.2, we use Theorem 1.5 instead of Theorem 1.3.
Theorem 3.8.
Let  and
and  be two positive decreasing
be two positive decreasing  -tuples,
-tuples,  be a real
be a real  -tuple such that conditions (1.10) are satisfied,
-tuple such that conditions (1.10) are satisfied,  is positive defined as in Theorem 2.6 and
is positive defined as in Theorem 2.6 and  ,
,  is defined as in (3.4). Then there exists
is defined as in (3.4). Then there exists  such that
such that

provided that  for every
for every  .
.
Proof.
Similar to the proof of Theorem 3.3.
Corollary 3.9.
Let  and
and  be two positive decreasing
be two positive decreasing  -tuples, let
-tuples, let  be a real
be a real  -tuple such that conditions (1.10) are satisfied and
-tuple such that conditions (1.10) are satisfied and  is positive defined as in Theorem 2.6, then for
is positive defined as in Theorem 2.6, then for  there exists
there exists  , with
, with  being defined as in (3.4), such that
being defined as in (3.4), such that

Proof.
Setting  and
and  ,
,  in (3.23), we get (3.24).
in (3.23), we get (3.24).
Remark 3.10.
Since the function  is invertible, then from (3.24) we have
is invertible, then from (3.24) we have

In fact, similar result can also be given for (3.23). Namely, suppose that  has inverse function. Then from (3.23), we have
has inverse function. Then from (3.23), we have

So, we have that the expression on the right-hand side of (3.26) is also a mean. By the inequality (3.25), we can consider for positive  -tuples
-tuples  and
and  such that conditions (1.10) are satisfied, and
such that conditions (1.10) are satisfied, and

for  , as means in broader sense. Moreover we can extend these means in other cases. So passing to the limit, we have
, as means in broader sense. Moreover we can extend these means in other cases. So passing to the limit, we have

Theorem 3.11.
Let  such that
such that  ,
,  , then the following inequality is valid:
, then the following inequality is valid:

Proof.
Since  is
is  -convex, therefore by (2.21) we get (3.29)
-convex, therefore by (2.21) we get (3.29)
4. Some Related Results
Let  ,
,  be real valued functions defined on an interval
be real valued functions defined on an interval  such that
such that  ,
,  both exist for all
both exist for all  .
.
Definition 4.1 (see [1, page 324]).

is said to majorize  , in symbol,
, in symbol,  , for
, for  if they are decreasing in
if they are decreasing in  and
and

and equality in (4.1) holds for  .
.
The following theorem can be regarded as a majorization theorem in integral case [1, page 325].
Theorem 4.2.

for  iff they are decreasing in
iff they are decreasing in  and
and

holds for every  that is continuous, and convex in
that is continuous, and convex in  such that the integrals exist.
such that the integrals exist.
The following theorem is a simple consequence of Theorem  in [8] (see also [1, page 328]):
in [8] (see also [1, page 328]):
Theorem 4.3.
Let  ,
,  and
and  are continuous and increasing and let
are continuous and increasing and let  be a function of bounded variation.
be a function of bounded variation.
(a)If


hold, then for every continuous convex function  , one has
, one has

(b)If (4.3) holds, then (4.5) holds for every continuous increasing convex function  .
.
Theorem 4.4.
Let  and
and  be two positive functions defined on an interval
be two positive functions defined on an interval  , decreasing in
, decreasing in  ,
,  ,
,

and  is positive.
is positive.
Then the following statements are valid.
(a)For every  and
and  , the matrix
, the matrix  is a positive semidefinite matrix. Particularly
is a positive semidefinite matrix. Particularly

for 
(b)The function  is exponentially convex.
is exponentially convex.
(c)The function  is
is  -convex on
-convex on  and the following inequality holds for
and the following inequality holds for 

Proof.
As in the proof of Theorem 2.4, we use Theorem 4.2 instead of Theorem 1.3.
Theorem 4.5.
Let  be defined as in Theorem 4.4 and
be defined as in Theorem 4.4 and  such that
such that  ,
,  ,
,  , and
, and  . Then
. Then

Proof.
Similar to the proof of Theorem 2.5.
Denote

In the above expression,  and
and  are the minimums of
are the minimums of  and
and  , respectively. Similarly,
, respectively. Similarly,  and
and  are the maximums of
are the maximums of  and
and  , respectively.
, respectively.
Theorem 4.6.
Let  and
and  be two positive decreasing functions in
be two positive decreasing functions in  such that
such that  ,
,  is positive defined as in Theorem 4.4, and
is positive defined as in Theorem 4.4, and  , with
, with  being defined as in (4.10), then there exists
being defined as in (4.10), then there exists  such that
such that

Proof.
As in the proof of Theorem 3.2, we use Theorem 4.2 instead of Theorem 1.3.
Theorem 4.7.
Let  and
and  be two positive decreasing functions in
be two positive decreasing functions in  such that
such that  ,
,  is positive defined as in Theorem 4.4, and
is positive defined as in Theorem 4.4, and  , with
, with  being defined as in (4.10). Then there exists
being defined as in (4.10). Then there exists  such that
such that

provided that  for every
for every  .
.
Proof.
Similar to the proof of Theorem 3.3.
Corollary 4.8.
Let  and
and  be two positive decreasing functions in
be two positive decreasing functions in  such that
such that  and
and  is positive defined as in Theorem 4.4, then for
is positive defined as in Theorem 4.4, then for  there exists
there exists  , with
, with  being defined as in (4.10), such that
being defined as in (4.10), such that

Proof.
Set  and
and  ,
,  in (4.12), we get (4.13).
in (4.12), we get (4.13).
Remark 4.9.
Since the function  is invertible, then from (4.13) we have
is invertible, then from (4.13) we have

In fact, similar result can also be given for (4.12). Namely, suppose that  has inverse function. Then from (4.12), we have
has inverse function. Then from (4.12), we have

So, we have that the expression on the right-hand side of (4.15) is also a mean. By the inequality (4.14), we can consider for positive functions  and
and  such that
such that  , and
, and

for  , as means in broader sense. Moreover we can extend these means in other cases. So passing to the limit, we have
, as means in broader sense. Moreover we can extend these means in other cases. So passing to the limit, we have

Theorem 4.10.
Let  such that
such that  ,
,  , then the following inequality is valid:
, then the following inequality is valid:

Proof.
Since  is
is  -convex, therefore by (4.9) we get (4.18).
-convex, therefore by (4.9) we get (4.18).
Theorem 4.11.
Let  ,
,  and
and  are positive, continuous, and increasing and let
are positive, continuous, and increasing and let  be a function of bounded variation. Also let
be a function of bounded variation. Also let

such that conditions (4.3) and (4.4) are satisfied and  is positive.
is positive.
Then the following statements are valid.
(a)For every  and
and  , the matrix
, the matrix  is a positive semidefinite matrix. Particularly
is a positive semidefinite matrix. Particularly

for 
(b)The function  is exponentially convex.
is exponentially convex.
(c)The function  is
is  -convex on
-convex on  and the following inequality holds for
and the following inequality holds for 

Proof.
As in the proof of Theorem 2.4, we use Theorem 4.3 instead of Theorem 1.3.
Theorem 4.12.
Let  be defined as in Theorem 4.11 and
be defined as in Theorem 4.11 and  such that
such that  ,
,  ,
,  , and
, and  . Then
. Then

Proof.
Similar to the proof of Theorem 2.5.
Theorem 4.13.
Let  and
and  be positive, continuous, and increasing functions in
be positive, continuous, and increasing functions in  such that conditions (4.3) and (4.4) are satisfied,
such that conditions (4.3) and (4.4) are satisfied,  is positive defined as in Theorem 4.11,
is positive defined as in Theorem 4.11,  with
with  being defined as in (4.10), and
being defined as in (4.10), and  be a function of bounded variation, then there exists
be a function of bounded variation, then there exists  such that
such that

Proof.
As in the proof of Theorem 3.2, we use Theorem 4.3 instead of Theorem 1.3.
Theorem 4.14.
Let  and
and  be positive, continuous and increasing functions in
be positive, continuous and increasing functions in  such that conditions (4.3) and (4.4) are satisfied,
such that conditions (4.3) and (4.4) are satisfied,  be a function of bounded variation,
be a function of bounded variation,  is positive defined as in Theorem 4.11, and
is positive defined as in Theorem 4.11, and  , with
, with  being defined as in (4.10). Then there exists
being defined as in (4.10). Then there exists  such that
such that

provided that  for every
for every  .
.
Proof.
Similar to the proof of Theorem 3.3.
Corollary 4.15.
Let  and
and  be positive, continuous, and increasing functions in
be positive, continuous, and increasing functions in  such that conditions (4.3) and (4.4) be satisfied,
such that conditions (4.3) and (4.4) be satisfied,  is positive defined as in Theorem 4.11, and
is positive defined as in Theorem 4.11, and  be a function of bounded variation, then for
be a function of bounded variation, then for  there exists
there exists  , with
, with  being defined as in (4.10), such that
being defined as in (4.10), such that

Proof.
Setting  and
and  ,
,  in (4.24), we get (4.25).
in (4.24), we get (4.25).
Remark 4.16.
Since the function  is invertible, then from (4.25) we have
is invertible, then from (4.25) we have

In fact, similar result can also be given for (4.24). Namely, suppose that  has inverse function. Then from (4.24), we have
has inverse function. Then from (4.24), we have

So, we have that the expression on the right-hand side of (4.27) is also a mean. By the inequality (4.26), we can consider for positive functions  and
and  such that conditions (4.3) and (4.4) are satisfied, and
such that conditions (4.3) and (4.4) are satisfied, and

for  , as means in broader sense. Moreover we can extend these means in other cases. So passing to the limit, we have
, as means in broader sense. Moreover we can extend these means in other cases. So passing to the limit, we have

Theorem 4.17.
Let  such that
such that  ,
,  , then the following inequality is valid:
, then the following inequality is valid:

Proof.
Since  is
is  -convex, therefore by (4.22) we get (4.30)
-convex, therefore by (4.22) we get (4.30)
Remark 4.18.
Let  such that
such that  and
and  . If we substitute in Theorem 2.6
. If we substitute in Theorem 2.6  , we get (1.2). In fact in such results we have that
, we get (1.2). In fact in such results we have that  is monotonic
is monotonic  -tuple. But since the weights are positive, our results are also valid for arbitrary
-tuple. But since the weights are positive, our results are also valid for arbitrary  .
.
References
Pečarić JE, Proschan F, Tong YL: Convex Functions, Partial Orderings, and Statistical Applications, Mathematics in Science and Engineering. Volume 187. Academic Press, Boston, Mass, USA; 1992:xiv+467.
Anwar M, Pečarić J: On logarithmic convexity for differences of power means and related results. Mathematical Inequalities & Applications 2009, 12(1):81–90.
Marshall AW, Olkin I: Inequalities: Theory of Majorization and Its Applications, Mathematics in Science and Engineering. Volume 143. Academic Press, New York, NY, USA; 1979:xx+569.
Kadelburg Z, Dukić D, Lukić M, Matić I: Inequalities of Karamata. Schur and Muirhead, and some applications. The Teaching of Mathematics 2005, 8(1):31–45.
Fuchs L: A new proof of an inequality of Hardy-Littlewood-Pólya. Mathematics Tidsskr 1947, 53–54.
Anwar M, Jekšetić J, Pečarić J, ur Rehman A: Exponential convexity, positive semi-definite matrices and fundamental inequalities. to appear in Journal of Mathematical Inequalities
Anwar M, Pečarić J: New means of Cauchy's type. Journal of Inequalities and Applications 2008, 2008:-10.
Pečarić JE: On some inequalities for functions with nondecreasing increments. Journal of Mathematical Analysis and Applications 1984, 98(1):188–197. 10.1016/0022-247X(84)90287-7
Acknowledgments
This research work is funded by Higher Education Commission of Pakistan. The research of the third author was supported by the Croatian Ministry of Science, Education and Sports under the Research Grants 117-1170889-0888. The authors thank I. Olkin for many valuable suggestions.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Anwar, M., Latif, N. & Pečarić, J. Positive Semidefinite Matrices, Exponential Convexity for Majorization, and Related Cauchy Means. J Inequal Appl 2010, 728251 (2010). https://doi.org/10.1155/2010/728251
Received:
Accepted:
Published:
DOI: https://doi.org/10.1155/2010/728251
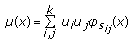
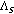 is continuous for
is continuous for  . Then by using Proposition 1.7, we get exponentially convexity of the function
. Then by using Proposition 1.7, we get exponentially convexity of the function 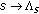 .
. is continuous and strictly convex function for
is continuous and strictly convex function for 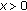 and all
and all  's and
's and 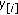 's are not equal, therefore by Theorem 1.3 with
's are not equal, therefore by Theorem 1.3 with  we have
we have