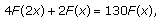- Research Article
- Open access
- Published:
Stability Problems of Quintic Mappings in Quasi- -Normed Spaces
-Normed Spaces
Journal of Inequalities and Applications volume 2010, Article number: 368981 (2010)
Abstract
We investigate the generalized Hyers-Ulam-Rassias stability problem in quasi- -normed spaces and then the stability by using a subadditive function for the quintic function
-normed spaces and then the stability by using a subadditive function for the quintic function  such that
such that  , for all
, for all  .
.
1. Introduction
In 1940 Ulam [1] proposed the problem concerning the stability of group homomorphisms as follows.Let be a group and let
be a group and let be a metric group with the metric
be a metric group with the metric . Given that
. Given that , does there exist a
, does there exist a such that if a function
such that if a function satisfies the inequality
satisfies the inequality for all
for all then there is a homomorphism
then there is a homomorphism with
with for all
for all ? In other words, when is it true that a mapping satisfying a functional equation approximately must be close to the solution of the given functional equation ? In 1941, Hyers [2] considered the case of approximately additive mappings under the assumption that
? In other words, when is it true that a mapping satisfying a functional equation approximately must be close to the solution of the given functional equation ? In 1941, Hyers [2] considered the case of approximately additive mappings under the assumption that  and
and  are Banach spaces.
are Banach spaces.
The famous Hyers stability result that appeared in [2] was generalized by Aoki [3] for the stability of the additive mapping involving a sum of powers of  -norms. In 1978, Th. M. Rassias [4] provided a generalization of Hyers' Theorem for the stability of the linear mapping, which allows the Cauchy difference to be unbounded. This result of Th. M. Rassias lead mathematicians working in stability of functional equations to establish what is known today as Hyers-Ulam-Rassias stability or Cauchy-Rassias stability as well as to introduce new definitions of stability concepts. During the last three decades, several stability problems of a large variety of functional equations have been extensively studied and generalized by a number of authors [5–14]. In particular, J. M. Rassias [15] introduced the quartic functional equation
-norms. In 1978, Th. M. Rassias [4] provided a generalization of Hyers' Theorem for the stability of the linear mapping, which allows the Cauchy difference to be unbounded. This result of Th. M. Rassias lead mathematicians working in stability of functional equations to establish what is known today as Hyers-Ulam-Rassias stability or Cauchy-Rassias stability as well as to introduce new definitions of stability concepts. During the last three decades, several stability problems of a large variety of functional equations have been extensively studied and generalized by a number of authors [5–14]. In particular, J. M. Rassias [15] introduced the quartic functional equation

It is easy to see that  is a solution of (1.1) by virtue of the identity
is a solution of (1.1) by virtue of the identity

For this reason, (1.1) is called a quartic functional equation. Also Chung and Sahoo [16] determined the general solution of (1.1) without assuming any regularity conditions on the unknown function. In fact, they proved that the function  is a solution of (1.1) if and only if
is a solution of (1.1) if and only if  where the function
where the function  is symmetric and additive in each variable.
is symmetric and additive in each variable.
Similar to the quartic functional equation, we may define quintic functional equation as follows.
Definition 1.1.
Let  be a linear space and let
be a linear space and let  be a real complete linear space. Then a mapping
be a real complete linear space. Then a mapping  is called quintic if the quintic functional equation
is called quintic if the quintic functional equation

holds for all 
Note that the mapping  is called quintic because the following algebraic identity
is called quintic because the following algebraic identity

holds for all  .
.
Let  be a real number with
be a real number with  and let
and let  be either
be either  or
or  We will consider the definition and some preliminary results of a quasi-
We will consider the definition and some preliminary results of a quasi- -norm on a linear space.
-norm on a linear space.
Definition 1.2.
Let  be a linear space over a field
be a linear space over a field  A
A  -
- -norm
-norm is a real-valued function on
is a real-valued function on  satisfying the followings.
satisfying the followings.
(1) for all
for all  and
and  if and only if
if and only if  .
.
(2) for all
for all  and all
and all  .
.
(3)There is a constant  such that
such that  for all
for all  .
.
The pair  is called aquasi-
is called aquasi- -normed space if
-normed space if  is a quasi-
is a quasi- -norm on
-norm on  The smallest possible
The smallest possible  is called the modulus of concavity of
is called the modulus of concavity of  A quasi-Banach space is a complete quasi-
A quasi-Banach space is a complete quasi- -normed space.
-normed space.
A quasi- -norm
-norm  is called a
is called a  -norm (
-norm ( if
if  for all
for all  In this case, a quasi-
In this case, a quasi- -Banach space is called a
-Banach space is called a  -Banach space; see [17–19].
-Banach space; see [17–19].
In this paper, we consider the following quintic functional equation:

for all  We investigate the generalized Hyers-Ulam-Rassias stability problem in quasi-
We investigate the generalized Hyers-Ulam-Rassias stability problem in quasi- -normed spaces and then the stability by using a subadditive function for the quintic function
-normed spaces and then the stability by using a subadditive function for the quintic function  satisfying (1.5).
satisfying (1.5).
2. Quintic Functional Equations
Lemma 2.1.
Let  be a quintic mapping satisfying (1.3). Then
be a quintic mapping satisfying (1.3). Then
(1) , for all
, for all  and
and  ,
,
(2)
(3) is an odd mapping,
is an odd mapping,
Proof.
-
(1)
Letting
 in (1.3), we have
in (1.3), we have 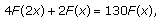 (2.1)
(2.1)
that is,  , for all
, for all  . Now inductively replacing
. Now inductively replacing  by
by  , we have the desired result. (2) Putting
, we have the desired result. (2) Putting  in (1.3),
in (1.3),

Hence  (3) Letting
(3) Letting  in (1.3), we get
in (1.3), we get

for all  By (1) and (2), we have
By (1) and (2), we have

for all  Thus it is an odd mapping.
Thus it is an odd mapping.
Note that  for all
for all  and
and  .
.
3. Stabilities
Throughout this section, let  be a quasi-
be a quasi- -normed space and let
-normed space and let  be a quasi-
be a quasi- -Banach space with a quasi-
-Banach space with a quasi- -norm
-norm  . Let
. Let  be the modulus of concavity of
be the modulus of concavity of  . We will investigate the generalized Hyers-Ulam-Rassias stability problem for the functional equation (1.5). After that we will study the stability by using a subadditive function. For a given mapping
. We will investigate the generalized Hyers-Ulam-Rassias stability problem for the functional equation (1.5). After that we will study the stability by using a subadditive function. For a given mapping  , let
, let

for all  .
.
Theorem 3.1.
Suppose that there exists a mapping  for which a mapping
for which a mapping  satisfies
satisfies

and the series  converges for all
converges for all  Then there exists a unique quintic mapping
Then there exists a unique quintic mapping  which satisfies (1.3) and the inequality
which satisfies (1.3) and the inequality

for all 
Proof.
By letting  in the inequality (3.2), we have
in the inequality (3.2), we have

that is,

for all  Now, replacing
Now, replacing  by
by  and multiplying
and multiplying  in the inequality (3.5), we get
in the inequality (3.5), we get

for all  Combining the two equations (3.5) and (3.6), we have
Combining the two equations (3.5) and (3.6), we have

for all  Inductively, since
Inductively, since  we have
we have

for all 
 For all
For all  and
and  with
with  and inductively switching
and inductively switching  and
and  and multiplying
and multiplying  in the inequality (3.5), we have
in the inequality (3.5), we have

for all  Since the right-hand side of the previous inequality tends to 0 as
Since the right-hand side of the previous inequality tends to 0 as  hence
hence  is a Cauchy sequence in the quasi-
is a Cauchy sequence in the quasi- -Banach space
-Banach space  Thus we may define
Thus we may define

for all  Since
Since  , replacing
, replacing  and
and  by
by  and
and  respectively, and dividing by
respectively, and dividing by  in the inequality (3.2), we have
in the inequality (3.2), we have

for all  By taking
By taking  the definition of
the definition of  implies that
implies that  satisfies (1.3) for all
satisfies (1.3) for all  that is,
that is,  is the quintic mapping. Also, the inequality (3.8) implies the inequality (3.3). Now, it remains to show the uniqueness. Assume that there exists
is the quintic mapping. Also, the inequality (3.8) implies the inequality (3.3). Now, it remains to show the uniqueness. Assume that there exists  satisfying (1.3) and (3.3). Lemma 2.1 implies that
satisfying (1.3) and (3.3). Lemma 2.1 implies that  and
and  for all
for all  . Then
. Then

for all  By letting
By letting  we immediately have the uniqueness of
we immediately have the uniqueness of 
Theorem 3.2.
Suppose that there exists a mapping  for which a mapping
for which a mapping  satisfies
satisfies

and the series  converges for all
converges for all  Then there exists a unique quintic mapping
Then there exists a unique quintic mapping  which satisfies (1.3) and the inequality
which satisfies (1.3) and the inequality

for all 
Proof.
If  is replaced by
is replaced by  in the inequality (3.5), then the proof follows from the proof of Theorem 3.1.
in the inequality (3.5), then the proof follows from the proof of Theorem 3.1.
Now we will recall a subadditive function and then investigate the stability under the condition that the space  is a
is a  -Banach space. The basic definitions of subadditive functions follow from [19].
-Banach space. The basic definitions of subadditive functions follow from [19].
A function  having a domain
having a domain  and a codomain
and a codomain  that are both closed under addition is called
that are both closed under addition is called
(1)a subadditive function if  ,
,
(2)a contractively subadditive function if there exists a constant  with
with  such that
such that  ,
,
(3)an expansively superadditive function if there exists a constant  with
with  such that
such that  ,
,
for all  .
.
Theorem 3.3.
Suppose that there exists a mapping  for which a mapping
for which a mapping  satisfies
satisfies

for all  and the map
and the map  is contractively subadditive with a constant
is contractively subadditive with a constant  such that
such that  Then there exists a unique quintic mapping
Then there exists a unique quintic mapping  which satisfies (1.3) and the inequality
which satisfies (1.3) and the inequality

for all 
Proof.
By the inequalities (3.5) and (3.9) of the proof of Theorem 3.1, we have

that is,

for all  and for all
and for all  and
and  with
with  Hence
Hence  is a Cauchy sequence in the space
is a Cauchy sequence in the space  Thus we may define
Thus we may define

for all  Now, we will show that the map
Now, we will show that the map  is a generalized quintic mapping. Then
is a generalized quintic mapping. Then

for all  Hence the mapping
Hence the mapping  is a quintic mapping. Note that the inequality (3.18) implies the inequality (3.16) by letting
is a quintic mapping. Note that the inequality (3.18) implies the inequality (3.16) by letting  and taking
and taking  Assume that there exists
Assume that there exists  satisfying (1.5) and (3.16). We know that
satisfying (1.5) and (3.16). We know that  for all
for all  Then
Then

that is,

for all  By letting
By letting  , we immediately have the uniqueness of
, we immediately have the uniqueness of  .
.
Theorem 3.4.
Suppose that there exists a mapping  for which a mapping
for which a mapping  satisfies
satisfies

for all  and the map
and the map  is expansively superadditive with a constant
is expansively superadditive with a constant  such that
such that  Then there exists a unique quintic mapping
Then there exists a unique quintic mapping  which satisfies (1.3) and the inequality
which satisfies (1.3) and the inequality

for all 
Proof.
By letting  in (3.23), we have
in (3.23), we have

and then replacing  by
by  ,
,

for all  . For all
. For all  and
and  with
with  , inductively we have
, inductively we have

for all  . The remains follow from the proof of Theorem 3.3.
. The remains follow from the proof of Theorem 3.3.
References
Ulam SM: Problems in Modern Mathematics. Wiley, New York, NY, USA; 1960.
Hyers DH: On the stability of the linear functional equation. Proceedings of the National Academy of Sciences of the United States of America 1941, 27: 222–224. 10.1073/pnas.27.4.222
Aoki T: On the stability of the linear transformation in Banach spaces. Journal of the Mathematical Society of Japan 1950, 2: 64–66. 10.2969/jmsj/00210064
Rassias ThM: On the stability of the linear mapping in Banach spaces. Proceedings of the American Mathematical Society 1978, 72(2):297–300. 10.1090/S0002-9939-1978-0507327-1
Bae J-H, Park W-G: On the generalized Hyers-Ulam-Rassias stability in Banach modules over a -algebra. Journal of Mathematical Analysis and Applications 2004, 294(1):196–205. 10.1016/j.jmaa.2004.02.009
Czerwik S: On the stability of the quadratic mapping in normed spaces. Abhandlungen aus dem Mathematischen Seminar der Universität Hamburg 1992, 62: 59–64. 10.1007/BF02941618
Czerwik S: Functional Equations and Inequalities in Several Variables. World Scientific, River Edge, NJ, USA; 2002:x+410.
Gajda Z: On stability of additive mappings. International Journal of Mathematics and Mathematical Sciences 1991, 14(3):431–434. 10.1155/S016117129100056X
Hyers DH, Rassias ThM: Approximate homomorphisms. Aequationes Mathematicae 1992, 44(2–3):125–153. 10.1007/BF01830975
Rassias ThM: On the stability of functional equations and a problem of Ulam. Acta Applicandae Mathematicae 2000, 62(1):23–130. 10.1023/A:1006499223572
Hyers DH, Isac G, Rassias ThM: Stability of Functional Equations in Several Variables, Progress in Nonlinear Differential Equations and Their Applications, 34. Birkhäuser, Boston, Mass, USA; 1998:vi+313.
Rassias ThM: On the stability of functional equations in Banach spaces. Journal of Mathematical Analysis and Applications 2000, 251(1):264–284. 10.1006/jmaa.2000.7046
Rassias ThM, Šemrl P: On the Hyers-Ulam stability of linear mappings. Journal of Mathematical Analysis and Applications 1993, 173(2):325–338. 10.1006/jmaa.1993.1070
Rassias ThM, Shibata K: Variational problem of some quadratic functionals in complex analysis. Journal of Mathematical Analysis and Applications 1998, 228(1):234–253. 10.1006/jmaa.1998.6129
Rassias JM: Solution of the Ulam stability problem for quartic mappings. Glasnik Matematički. Serija III 1999, 34(2):243–252.
Chung JK, Sahoo PK: On the general solution of a quartic functional equation. Bulletin of the Korean Mathematical Society 2003, 40(4):565–576.
Benyamini Y, Lindenstrauss J: Geometric Nonlinear Functional Analysis. Vol. 1, American Mathematical Society Colloquium Publications. Volume 48. American Mathematical Society, Providence, RI, USA; 2000:xii+488.
Rolewicz S: Metric Linear Spaces. 2nd edition. PWN-Polish Scientific Publishers, Warsaw, Poland; 1984:xi+459.
Rassias JM, Kim H-M: Generalized Hyers-Ulam stability for general additive functional equations in quasi--normed spaces. Journal of Mathematical Analysis and Applications 2009, 356(1):302–309. 10.1016/j.jmaa.2009.03.005
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License ( https://creativecommons.org/licenses/by/2.0 ), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Cho, I., Kang, D. & Koh, H. Stability Problems of Quintic Mappings in Quasi- -Normed Spaces.
J Inequal Appl 2010, 368981 (2010). https://doi.org/10.1155/2010/368981
-Normed Spaces.
J Inequal Appl 2010, 368981 (2010). https://doi.org/10.1155/2010/368981
Received:
Accepted:
Published:
DOI: https://doi.org/10.1155/2010/368981
 in (1.3), we have
in (1.3), we have