- Research Article
- Open access
- Published:
Weighted Composition Operators from Logarithmic Bloch-Type Spaces to Bloch-Type Spaces
Journal of Inequalities and Applications volume 2009, Article number: 964814 (2009)
Abstract
The boundedness and compactness of the weighted composition operators from logarithmic Bloch-type spaces to Bloch-type spaces are studied here.
1. Introduction
Let  be the unit disc in the complex plane
be the unit disc in the complex plane  ,
,  the normalized Lebesgue area measure on
the normalized Lebesgue area measure on  ,
,  the class of all holomorphic functions on
the class of all holomorphic functions on  , and
, and  the space of bounded holomorphic functions on
the space of bounded holomorphic functions on  with the norm
with the norm 
The logarithmic Bloch-type space  ,
,  was recently introduced in [1]. The space consists of all
was recently introduced in [1]. The space consists of all  such that
such that

The norm on  is introduced as follows:
is introduced as follows:

When  ,
,  becomes the
becomes the  -Bloch space
-Bloch space  . For
. For  -Bloch and other Bloch-type spaces, see, for example, [1–9], as well as the related references therein. For
-Bloch and other Bloch-type spaces, see, for example, [1–9], as well as the related references therein. For  ,
,  is the logarithmic Bloch space, which appeared in characterizing the multipliers of the Bloch space (see [3, 9]).
is the logarithmic Bloch space, which appeared in characterizing the multipliers of the Bloch space (see [3, 9]).
The little logarithmic Bloch-type space  ,
,  consists of all
consists of all  such that
such that

The following theorem summarizes the basic properties of the logarithmic Bloch-type spaces. Here, as usual, for fixed 
Theorem 1 A (see [1]).
The following statements are true.
(a)The logarithmic Bloch-type space  is Banach with the norm given in (1.2).
is Banach with the norm given in (1.2).
(b) is a closed subset of
is a closed subset of 
(c)Assume  Then
Then  if and only if
if and only if 
(d)The set of all polynomials is dense in  .
.
(e)Assume  then for each
then for each  ,
,  . Moreover
. Moreover

A positive continuous function  on
on  is called weight.
is called weight.
The Bloch-type space  consists of all
consists of all  such that
such that

where  is a weight. With the norm
is a weight. With the norm

the Bloch-type space becomes a Banach space.
The little Bloch-type space  is a subspace of
is a subspace of  consisting of all
consisting of all  such that
such that

Let  be a holomorphic self-map of
be a holomorphic self-map of  and
and  . For
. For  the corresponding weighted composition operator is defined by
the corresponding weighted composition operator is defined by

It is of interest to provide function-theoretic characterizations for when  and
and  induce bounded or compact weighted composition operators on spaces of holomorphic functions. For some classical results mostly on composition operators, see, for example, [10]. For some recent related results, mostly in
induce bounded or compact weighted composition operators on spaces of holomorphic functions. For some classical results mostly on composition operators, see, for example, [10]. For some recent related results, mostly in  or related to Bloch-type or weighted-type spaces, see, for example, [4, 10–46] and the references therein.
or related to Bloch-type or weighted-type spaces, see, for example, [4, 10–46] and the references therein.
Here we study the boundedness and compactness of the weighted composition operator from the logarithmic Bloch-type space and the little logarithmic Bloch-type space to the Bloch-type or the little Bloch-type space.
In this paper, constants are denoted by  , they are positive and may differ from one occurrence to the other. The notation
, they are positive and may differ from one occurrence to the other. The notation  means that there is a positive constant
means that there is a positive constant  such that
such that  . We say that
. We say that  , if both
, if both  and
and  hold.
hold.
2. Auxiliary Results
In this section we quote several auxiliary results which will be used in the proofs of the main results.
Lemma 2.1.
Assume  ,
,  then the following statements are true.
then the following statements are true.
(a)Assume  then the function
then the function

is increasing on the interval 
(b)The function

is increasing on the interval 
Proof.
-
(a)
We have
 (2.3)
(2.3)
Since  when
when  and
and  , and the function
, and the function  is decreasing on the interval
is decreasing on the interval  , we have
, we have

from which this statement follows.
The proof of (b) is similar, hence it is omitted.
The next lemma regarding the point evaluation functional on  follows from [1, Lemma 3] and some elementary asymptotic relationship, such as
follows from [1, Lemma 3] and some elementary asymptotic relationship, such as

Lemma 2.2.
Let  Then
Then

for some  independent of
independent of 
The proof of the following lemma is similar to [25, Lemma 2.1], so we omit it.
Lemma 2.3.
Assume  is a weight. A closed set
is a weight. A closed set  in
in  is compact if and only if it is bounded and
is compact if and only if it is bounded and

Remark 2.4.
If in Lemma 2.3 we assume that  is not closed, then the word compact can be replaced by relatively compact.
is not closed, then the word compact can be replaced by relatively compact.
The next characterization of compactness is proved in a standard way (see, e.g., the proofs of the corresponding lemmas in [10, 30, 47–49]). Hence we omit it.
Lemma 2.5.
Assume that  ,
,  is a holomorphic self-map of
is a holomorphic self-map of  and
and  is a weight. Let
is a weight. Let  be one of the following spaces
be one of the following spaces  ,
,  and
and  one of the spaces
one of the spaces  ,
,  . Then the operator
. Then the operator  is compact if and only if
is compact if and only if  is bounded and for every bounded sequence
is bounded and for every bounded sequence  converging to
converging to  uniformly on compacts of
uniformly on compacts of  one has
one has

Some concrete examples of the functions belonging to logarithmic Bloch-type spaces can be found in the next lemma.
Lemma 2.6.
The following statements are true.
(a)Assume that  and
and  then
then

where  and
and  is a nonconstant function belonging to
is a nonconstant function belonging to 
(b)Assume that  and
and  then
then

where  and
and  is a nonconstant function belonging to
is a nonconstant function belonging to 
(c)Assume that  then
then

where  and
and  is a nonconstant function belonging to
is a nonconstant function belonging to 
Moreover, for each  , it holds that
, it holds that  belong to the corresponding
belong to the corresponding  space, and for fixed
space, and for fixed  and
and 

Proof. (a) Let  be fixed. Then we have
be fixed. Then we have


where in (2.13) we have used that  and in (2.14) we have used the fact that the function in (2.1) is increasing on the interval
and in (2.14) we have used the fact that the function in (2.1) is increasing on the interval  .
.
From (2.13), since  , and by Lemma 2.1(a), we have that
, and by Lemma 2.1(a), we have that

as  , from which it follows that
, from which it follows that  as desired.
as desired.
-
(b)
For fixed
 , we have
, we have  (2.16)
(2.16)

where in (2.16) we have used the assumption  , while in (2.17), as in (a), we have used the fact that the function in (2.1) is increasing on the interval
, while in (2.17), as in (a), we have used the fact that the function in (2.1) is increasing on the interval  .
.
From (2.16), and by Lemma 2.1(a), we obtain

as  . Hence
. Hence  finishing the proof of this statement.
finishing the proof of this statement.
-
(c)
We have
 (2.19)
(2.19)

where we have used the assumption  and the fact that function (2.1) is increasing on
and the fact that function (2.1) is increasing on  .
.
From (2.19), Lemma 2.1(a), and since  we obtain
we obtain

as  that is,
that is, 
Estimations (2.12) follow from (2.14), (2.17), (2.20) and by using the following facts

we finish the proof of the lemma.
Remark 2.7.
Note that from Lemmas 2.2 and 2.6 the functions  defined in (2.9)–(2.11) have maximal growths in the corresponding logarithmic Bloch-type spaces.
defined in (2.9)–(2.11) have maximal growths in the corresponding logarithmic Bloch-type spaces.
3. Boundedness and Compactness of the Operator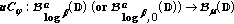
This section studies the boundedness and compactness of the weighted composition operator  .
.
Case 1.

,  .
.
Theorem 3.1.
Assume  ,
,  ,
,  is an analytic self-map of the unit disk,
is an analytic self-map of the unit disk,  , and
, and  is a weight. Then the operator
is a weight. Then the operator  is bounded if and only if
is bounded if and only if


Proof.
First assume that (3.1) and (3.2) hold. Then, by Lemma 2.2 and the definition of  , we have
, we have


Applying (3.1) and (3.2) in (3.4), the boundedness of  follows.
follows.
Now assume the operator  is bounded. By taking the test functions
is bounded. By taking the test functions  and
and  (which obviously belong to
(which obviously belong to  ), we obtain
), we obtain


From (3.5) and (3.6), and since the function  is bounded, it follows that
is bounded, it follows that

For  set
set

We have that  ,
,

and as an easy consequence of Lemma 2.6(a),  and
and  for each
for each 
Using these facts and the boundedness of  , for the test functions
, for the test functions  , where
, where  and
and  , we get
, we get

From (3.10) it follows that

On the other hand, by using (3.7) and Lemma 2.1(b), we have

Hence, (3.11) and (3.12) imply (3.2).
Let

Then

and by Lemma 2.6(a) we get  , and
, and  for every
for every  . Using the boundedness of
. Using the boundedness of  , the test functions
, the test functions  , and equalities (3.14) we get
, and equalities (3.14) we get

for each  ,
,  .
.
From (3.2), (3.5), (3.15), and using the fact that

condition (3.1) follows.
Theorem 3.2.
Assume  ,
,  ,
,  is an analytic self-map of the unit disk,
is an analytic self-map of the unit disk,  , and
, and  is a weight. Then the operator
is a weight. Then the operator  is compact if and only if
is compact if and only if  is bounded
is bounded


Proof.
Suppose that  is compact. Then it is clear that
is compact. Then it is clear that  is bounded. If
is bounded. If  , then (3.17) and (3.18) are vacuously satisfied. Hence assume that
, then (3.17) and (3.18) are vacuously satisfied. Hence assume that  . Let
. Let  be a sequence in
be a sequence in  such that
such that  as
as  , and
, and  where
where  is defined in (3.8). Then
is defined in (3.8). Then  ,
,  uniformly on compacts of
uniformly on compacts of  as
as  ,
,  and
and

Hence from (3.10) and Lemma 2.5 we have that

from which (3.18) follows.
Let  ,
,  where
where  is defined in (3.13). Then
is defined in (3.13). Then  and
and  uniformly on compact subsets of
uniformly on compact subsets of  as
as  . Since
. Since  is compact, we see that
is compact, we see that

From (3.15) we have

which along with (3.16), (3.18), and (3.21) implies

On the other hand, we have

for some positive  . From (3.23) and (3.24), equality (3.17) follows.
. From (3.23) and (3.24), equality (3.17) follows.
Conversely, assume that  is bounded and (3.17) and (3.18) hold. From the proof of Theorem 3.1 we know that
is bounded and (3.17) and (3.18) hold. From the proof of Theorem 3.1 we know that

On the other hand, from (3.17) and (3.18) we have that, for every  , there is a
, there is a  , such that
, such that

whenever  .
.
Assume  is a sequence in
is a sequence in  (or
(or  ) such that
) such that  and
and  converges to
converges to  uniformly on compact subsets of
uniformly on compact subsets of  as
as  Let
Let  . Then from (3.25), (3.26), and by Lemma 2.2, it follows that
. Then from (3.25), (3.26), and by Lemma 2.2, it follows that

Therefore

Since  converges to zero on compact subsets of
converges to zero on compact subsets of  as
as  , by the Weierstrass theorem it follows that the sequence
, by the Weierstrass theorem it follows that the sequence  also converges to zero on compact subsets of
also converges to zero on compact subsets of  as
as  , in particular
, in particular  and
and  . Using these facts and letting
. Using these facts and letting  in the last inequality, we obtain that
in the last inequality, we obtain that

Since  is an arbitrary positive number it follows that the last limit is equal to zero. Applying Lemma 2.5, the implication follows.
is an arbitrary positive number it follows that the last limit is equal to zero. Applying Lemma 2.5, the implication follows.
Theorem 3.3.
Assume  ,
,  ,
,  is an analytic self-map of the unit disk,
is an analytic self-map of the unit disk,  , and
, and  is a weight. Then
is a weight. Then  is bounded if and only if
is bounded if and only if  is bounded
is bounded


Proof.
First assume that  is bounded. Then, it is clear that
is bounded. Then, it is clear that  is bounded, and as usual by taking the test functions
is bounded, and as usual by taking the test functions  and
and  and using the fact
and using the fact  , we obtain (3.30) and (3.31).
, we obtain (3.30) and (3.31).
Conversely, assume that the operator  is bounded,
is bounded,  and condition (3.31) holds.
and condition (3.31) holds.
Then, for each polynomial  , we have
, we have

from which along with conditions (3.30) and (3.31) it follows that  . Since according to Theorem A the set of all polynomials is dense in
. Since according to Theorem A the set of all polynomials is dense in  we see that for every
we see that for every  there is a sequence of polynomials
there is a sequence of polynomials  such that
such that

From this and by the boundedness of the operator  we have that
we have that

as  Hence
Hence  and consequently
and consequently  is bounded.
is bounded.
Remark 3.4.
Note that Theorem 3.3 holds for all  and
and 
Theorem 3.5.
Assume  ,
,  ,
,  is an analytic self-map of the unit disk,
is an analytic self-map of the unit disk,  , and
, and  is a weight. Then the operator
is a weight. Then the operator  is compact if and only if
is compact if and only if


Proof.
If  is compact, then it is bounded so that conditions (3.30) and (3.31) hold. On the other hand,
is compact, then it is bounded so that conditions (3.30) and (3.31) hold. On the other hand,  is compact, which implies that (3.17) and (3.18) hold.
is compact, which implies that (3.17) and (3.18) hold.
By (3.18) we have that, for every  , there exists an
, there exists an  such that
such that

when  . From (3.31), there exists a
. From (3.31), there exists a  such that
such that

when  , and where
, and where  is the function in Lemma 2.1(b).
is the function in Lemma 2.1(b).
Therefore, when  and
and  , we have that
, we have that

On the other hand, if  and
and  , from (3.38) and Lemma 2.1(b) we have
, from (3.38) and Lemma 2.1(b) we have

Combining (3.39) and (3.40), we obtain (3.36). Similarly, from (3.17) and (3.30) is obtained (3.35), as claimed.
Conversely, assume that (3.35) and (3.36) hold. First note that (3.35) implies (3.30). Indeed if (3.30) did not hold then there would be a sequence  and a
and a  such that
such that

and  From this and the continuity of the function
From this and the continuity of the function

we would have that

which is a contradiction with (3.35).
For any  , we have
, we have

Using conditions (3.30), (3.35), and (3.36) in (3.44), it follows that  for each
for each  , moreover the set
, moreover the set

is bounded in  .
.
Taking the supremum in (3.44) over the unit ball of the space  then letting
then letting  and using conditions (3.30), (3.35), and (3.36), we obtain
and using conditions (3.30), (3.35), and (3.36), we obtain

from which along with Lemma 2.3 the compactness of the operator  follows.
follows.
Case 2.

,  .
.
Theorem 3.6.
Assume that  is an analytic self-map of the unit disk,
is an analytic self-map of the unit disk,  , and
, and  is a weight. Then the operator
is a weight. Then the operator  is bounded if and only if
is bounded if and only if

Proof.
The proof of the theorem is similar to the proof of Theorem 3.1. The sufficiency follows by using the triangle inequality in (3.3) and then the third inequality in Lemma 2.2 and the definition of the space  .
.
For the necessity it is enough to follow the lines of the corresponding part of the proof of Theorem 3.1 and use the test functions  ,
,


which belong to  (for the functions in (3.48) and (3.49) it easily follows by Lemma 2.6(b), where
(for the functions in (3.48) and (3.49) it easily follows by Lemma 2.6(b), where  is the function in (2.10). We omit the details.
is the function in (2.10). We omit the details.
The proofs of the following two theorems are similar to the proofs of Theorems 3.2 and 3.5, where the test functions in (3.48) and (3.49) are used as well as the lemmas in Section 2. Hence their proofs are omitted.
Theorem 3.7.
Assume that  is an analytic self-map of the unit disk,
is an analytic self-map of the unit disk,  and
and  is a weight. Then the operator
is a weight. Then the operator  is compact if and only if
is compact if and only if  is bounded
is bounded

Theorem 3.8.
Assume that  is an analytic self-map of the unit disk,
is an analytic self-map of the unit disk,  , and
, and  is a weight. Then the operator
is a weight. Then the operator  is compact if and only if
is compact if and only if

Case 3.

.
The following results were proved in [15]. Hence we quote them for the benefit of the reader, and without any proof.
Theorem 3.9.
Assume that  is an analytic self-map of the unit disk,
is an analytic self-map of the unit disk,  , and
, and  is a weight. Then the operator
is a weight. Then the operator  is bounded if and only if
is bounded if and only if

Theorem 3.10.
Assume that  is an analytic self-map of the unit disk,
is an analytic self-map of the unit disk,  , and
, and  is a weight. Then the operator
is a weight. Then the operator  is compact if and only if
is compact if and only if  is bounded
is bounded

Theorem 3.11.
Assume that  is an analytic self-map of the unit disk,
is an analytic self-map of the unit disk,  , and
, and  is a weight. Then the operator
is a weight. Then the operator  is compact if and only if
is compact if and only if

Case 4.

, or  and
and 
Here we consider the cases  , or
, or  and
and  .
.
Theorem 3.12.
Assume that  , or
, or  and
and  ,
,  ,
,  is a weight, and
is a weight, and  is a holomorphic self-map of
is a holomorphic self-map of  Then
Then  is bounded if and only if
is bounded if and only if  and condition (3.2) holds.
and condition (3.2) holds.
Proof.
The sufficiency follows by using the first inequality in Lemma 2.2 and the definition of the space  in (3.3).
in (3.3).
For the necessity, by using the test functions  we first get conditions (3.5) and (3.7). To get (3.2) for the case
we first get conditions (3.5) and (3.7). To get (3.2) for the case  and
and  we use the test functions
we use the test functions

Note that  ,
,

and similar to Lemma 2.6(b),  and
and  for each
for each 
Hence for the family  we get
we get

from which along with (3.5) and the assumption  , easily follows (3.2) in this case.
, easily follows (3.2) in this case.
When  , condition (3.2) follows as in Theorem 3.1, by using the test functions in (3.8).
, condition (3.2) follows as in Theorem 3.1, by using the test functions in (3.8).
Theorem 3.13.
Assume that  , or
, or  and
and  ,
,  ,
,  is a weight, and
is a weight, and  is a holomorphic self-map of
is a holomorphic self-map of  , and
, and  is bounded. Then
is bounded. Then  is compact if
is compact if

and condition (3.18) holds.
Proof.
The proof is similar to the corresponding parts of the proofs of Theorems 3.2 and 3.7, so is omitted.
Remark 3.14.
Note that if  , or
, or  and
and  and
and  is compact, then condition (3.18) is proved as in Theorems 3.2 and 3.7, by using the test functions in (3.8) and (3.48). If
is compact, then condition (3.18) is proved as in Theorems 3.2 and 3.7, by using the test functions in (3.8) and (3.48). If  then condition (3.58) is vacuously satisfied. At the moment, we are not sure if the compactness implies condition (3.58) in the case
then condition (3.58) is vacuously satisfied. At the moment, we are not sure if the compactness implies condition (3.58) in the case  . Hence for the interested readers we leave this as an open problem.
. Hence for the interested readers we leave this as an open problem.
The following theorem is proved as the corresponding part of Theorem 3.5.
Theorem 3.15.
Assume that  , or
, or  and
and  ,
,  ,
,  is a weight, and
is a weight, and  is a holomorphic self-map of
is a holomorphic self-map of  . Then the operator
. Then the operator  is compact if
is compact if  and condition (3.36) holds.
and condition (3.36) holds.
Remark 3.16.
Note that if  is compact, then clearly
is compact, then clearly  .
.
References
Stević S: On new Bloch-type spaces. Applied Mathematics and Computation 2009,215(2):841–849. 10.1016/j.amc.2009.06.009
Anderson JM, Clunie J, Pommerenke Ch: On Bloch functions and normal functions. Journal für die reine und angewandte Mathematik 1974, 270: 12–37.
Brown L, Shields AL: Multipliers and cyclic vectors in the Bloch space. The Michigan Mathematical Journal 1991,38(1):141–146.
Clahane DD, Stević S: Norm equivalence and composition operators between Bloch/Lipschitz spaces of the ball. Journal of Inequalities and Applications 2006, 2006:-11.
Li S, Stević S: Weighted-Hardy functions with Hadamard gaps on the unit ball. Applied Mathematics and Computation 2009,212(1):229–233. 10.1016/j.amc.2009.02.019
Stević S: On an integral operator on the unit ball in
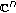 . Journal of Inequalities and Applications 2005,2005(1):81–88. 10.1155/JIA.2005.81
. Journal of Inequalities and Applications 2005,2005(1):81–88. 10.1155/JIA.2005.81Stević S: On Bloch-type functions with Hadamard gaps. Abstract and Applied Analysis 2007, 2007:-8.
Yamashita S: Gap series and
 -Bloch functions. Yokohama Mathematical Journal 1980,28(1–2):31–36.
-Bloch functions. Yokohama Mathematical Journal 1980,28(1–2):31–36.Zhu K: Spaces of Holomorphic Functions in the Unit Ball, Graduate Texts in Mathematics. Volume 226. Springer, New York, NY, USA; 2005:x+271.
Cowen CC, MacCluer BD: Composition Operators on Spaces of Analytic Functions, Studies in Advanced Mathematics. CRC Press, Boca Raton, Fla, USA; 1995:xii+388.
Fang Z-S, Zhou Z-H: Differences of composition operators on the space of bounded analytic functions in the polydisc. Abstract and Applied Analysis 2008, 2008:-10.
Fu X, Zhu X: Weighted composition operators on some weighted spaces in the unit ball. Abstract and Applied Analysis 2008, 2008:-8.
Galanopoulos P: On
 to
to  pullbacks. Journal of Mathematical Analysis and Applications 2008,337(1):712–725. 10.1016/j.jmaa.2007.02.049
pullbacks. Journal of Mathematical Analysis and Applications 2008,337(1):712–725. 10.1016/j.jmaa.2007.02.049Gu D: Weighted composition operators from generalized weighted Bergman spaces to weighted-type spaces. Journal of Inequalities and Applications 2008, 2008:-14.
Krantz SG, Stević S: On the iterated logarithmic Bloch space on the unit ball. Nonlinear Analysis: Theory, Methods & Applications 2009,71(5–6):1772–1795. 10.1016/j.na.2009.01.013
Li S, Stević S: Composition followed by differentiation between Bloch type spaces. Journal of Computational Analysis and Applications 2007,9(2):195–205.
Li S, Stević S: Weighted composition operators from Bergman-type spaces into Bloch spaces. Proceedings of Indian Academy of Sciences: Mathematical Sciences 2007,117(3):371–385. 10.1007/s12044-007-0032-y
Li S, Stević S: Weighted composition operators from
 -Bloch space to
-Bloch space to 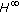 on the polydisc. Numerical Functional Analysis and Optimization 2007,28(7–8):911–925. 10.1080/01630560701493222
on the polydisc. Numerical Functional Analysis and Optimization 2007,28(7–8):911–925. 10.1080/01630560701493222Li S, Stević S: Weighted composition operators from
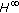 to the Bloch space on the polydisc. Abstract and Applied Analysis 2007, 2007:-13.
to the Bloch space on the polydisc. Abstract and Applied Analysis 2007, 2007:-13.Li S, Stević S: Generalized composition operators on Zygmund spaces and Bloch type spaces. Journal of Mathematical Analysis and Applications 2008,338(2):1282–1295. 10.1016/j.jmaa.2007.06.013
Li S, Stević S: Weighted composition operators between
 and
and  -Bloch spaces in the unit ball. Taiwanese Journal of Mathematics 2008,12(7):1625–1639.
-Bloch spaces in the unit ball. Taiwanese Journal of Mathematics 2008,12(7):1625–1639.Li S, Stević S: Products of integral-type operators and composition operators between Bloch-type spaces. Journal of Mathematical Analysis and Applications 2009,349(2):596–610. 10.1016/j.jmaa.2008.09.014
Lindström M, Wolf E: Essential norm of the difference of weighted composition operators. Monatshefte für Mathematik 2008,153(2):133–143. 10.1007/s00605-007-0493-1
MacCluer BD, Zhao R: Essential norms of weighted composition operators between Bloch-type spaces. The Rocky Mountain Journal of Mathematics 2003,33(4):1437–1458. 10.1216/rmjm/1181075473
Madigan K, Matheson A: Compact composition operators on the Bloch space. Transactions of the American Mathematical Society 1995,347(7):2679–2687. 10.2307/2154848
Montes-Rodríguez A: Weighted composition operators on weighted Banach spaces of analytic functions. Journal of the London Mathematical Society 2000,61(3):872–884. 10.1112/S0024610700008875
Ohno S: Weighted composition operators between
 and the Bloch space. Taiwanese Journal of Mathematics 2001,5(3):555–563.
and the Bloch space. Taiwanese Journal of Mathematics 2001,5(3):555–563.Ohno S, Zhao R: Weighted composition operators on the Bloch space. Bulletin of the Australian Mathematical Society 2001,63(2):177–185. 10.1017/S0004972700019250
Shi J, Luo L: Composition operators on the Bloch space of several complex variables. Acta Mathematica Sinica 2000,16(1):85–98. 10.1007/s101149900028
Stević S: Composition operators between
 and
and  -Bloch spaces on the polydisc. Zeitschrift für Analysis und ihre Anwendungen 2006,25(4):457–466.
-Bloch spaces on the polydisc. Zeitschrift für Analysis und ihre Anwendungen 2006,25(4):457–466.Stević S: Weighted composition operators between mixed norm spaces and
 spaces in the unit ball. Journal of Inequalities and Applications 2007, 2007:-9.
spaces in the unit ball. Journal of Inequalities and Applications 2007, 2007:-9.Stević S: Essential norms of weighted composition operators from
 -Bloch space to a weighted-type space on the unit ball. Abstract and Applied Analysis 2008, 2008:-11.
-Bloch space to a weighted-type space on the unit ball. Abstract and Applied Analysis 2008, 2008:-11.Stević S: Norm of weighted composition operators from Bloch space to
 on the unit ball. Ars Combinatoria 2008, 88: 125–127.
on the unit ball. Ars Combinatoria 2008, 88: 125–127.Stević S: On a new integral-type operator from the weighted Bergman space to the Bloch-type space on the unit ball. Discrete Dynamics in Nature and Society 2008, 2008:-14.
Stević S: Essential norms of weighted composition operators from the Bergman space to weighted-type spaces on the unit ball. Ars Combinatoria 2009, 91: 391–400.
Stević S: On a new integral-type operator from the Bloch space to Bloch-type spaces on the unit ball. Journal of Mathematical Analysis and Applications 2009,354(2):426–434. 10.1016/j.jmaa.2008.12.059
Stević S: Weighted composition operators from weighted Bergman spaces to weighted-type spaces on the unit ball. Applied Mathematics and Computation 2009,212(2):499–504. 10.1016/j.amc.2009.02.057
Ueki S-I: Composition operators on the Privalov spaces of the unit ball of
 . Journal of the Korean Mathematical Society 2005,42(1):111–127.
. Journal of the Korean Mathematical Society 2005,42(1):111–127.Ueki S-I, Luo L: Compact weighted composition operators and multiplication operators between Hardy spaces. Abstract and Applied Analysis 2008, 2008:-12.
Ueki S-I, Luo L: Essential norms of weighted composition operators between weighted Bergman spaces of the ball. Acta Scientiarum Mathematicarum 2008,74(3–4):829–843.
Wolf E: Compact differences of composition operators. Bulletin of the Australian Mathematical Society 2008,77(1):161–165.
Wolf E: Weighted composition operators between weighted Bergman spaces and weighted Bloch type spaces. Journal of Computational Analysis and Applications 2009,11(2):317–321.
Yang W: Weighted composition operators from Bloch-type spaces to weighted-type spaces. to appear in Ars Combinatoria to appear in Ars Combinatoria
Ye S: Weighted composition operator between the little
 -Bloch spaces and the logarithmic Bloch. Journal of Computational Analysis and Applications 2008,10(2):243–252.
-Bloch spaces and the logarithmic Bloch. Journal of Computational Analysis and Applications 2008,10(2):243–252.Zhu X: Generalized weighted composition operators from Bloch type spaces to weighted Bergman spaces. Indian Journal of Mathematics 2007,49(2):139–150.
Zhu X: Weighted composition operators from
 spaces to
spaces to  spaces to spaces. Abstract and Applied Analysis 2009, 2009:-14.
spaces to spaces. Abstract and Applied Analysis 2009, 2009:-14.Li S, Stević S: Riemann-Stieltjes-type integral operators on the unit ball in
 . Complex Variables and Elliptic Equations 2007,52(6):495–517. 10.1080/17476930701235225
. Complex Variables and Elliptic Equations 2007,52(6):495–517. 10.1080/17476930701235225Stević S: Boundedness and compactness of an integral operator on a weighted space on the polydisc. Indian Journal of Pure and Applied Mathematics 2006,37(6):343–355.
Stevich S: Boundedness and compactness of an integral operator in a mixed norm space on the polydisc. Sibirskiĭ Matematicheskiĭ Zhurnal 2007,48(3):559–569.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Stević, S., Agarwal, R.P. Weighted Composition Operators from Logarithmic Bloch-Type Spaces to Bloch-Type Spaces. J Inequal Appl 2009, 964814 (2009). https://doi.org/10.1155/2009/964814
Received:
Accepted:
Published:
DOI: https://doi.org/10.1155/2009/964814

 , we have
, we have 

 . Journal of Inequalities and Applications 2005,2005(1):81–88. 10.1155/JIA.2005.81
. Journal of Inequalities and Applications 2005,2005(1):81–88. 10.1155/JIA.2005.81 -Bloch functions. Yokohama Mathematical Journal 1980,28(1–2):31–36.
-Bloch functions. Yokohama Mathematical Journal 1980,28(1–2):31–36. to
to  pullbacks. Journal of Mathematical Analysis and Applications 2008,337(1):712–725. 10.1016/j.jmaa.2007.02.049
pullbacks. Journal of Mathematical Analysis and Applications 2008,337(1):712–725. 10.1016/j.jmaa.2007.02.049 -Bloch space to
-Bloch space to  on the polydisc. Numerical Functional Analysis and Optimization 2007,28(7–8):911–925. 10.1080/01630560701493222
on the polydisc. Numerical Functional Analysis and Optimization 2007,28(7–8):911–925. 10.1080/01630560701493222 to the Bloch space on the polydisc. Abstract and Applied Analysis 2007, 2007:-13.
to the Bloch space on the polydisc. Abstract and Applied Analysis 2007, 2007:-13. and
and  -Bloch spaces in the unit ball. Taiwanese Journal of Mathematics 2008,12(7):1625–1639.
-Bloch spaces in the unit ball. Taiwanese Journal of Mathematics 2008,12(7):1625–1639. and the Bloch space. Taiwanese Journal of Mathematics 2001,5(3):555–563.
and the Bloch space. Taiwanese Journal of Mathematics 2001,5(3):555–563. and
and  -Bloch spaces on the polydisc. Zeitschrift für Analysis und ihre Anwendungen 2006,25(4):457–466.
-Bloch spaces on the polydisc. Zeitschrift für Analysis und ihre Anwendungen 2006,25(4):457–466. spaces in the unit ball. Journal of Inequalities and Applications 2007, 2007:-9.
spaces in the unit ball. Journal of Inequalities and Applications 2007, 2007:-9. -Bloch space to a weighted-type space on the unit ball. Abstract and Applied Analysis 2008, 2008:-11.
-Bloch space to a weighted-type space on the unit ball. Abstract and Applied Analysis 2008, 2008:-11. on the unit ball. Ars Combinatoria 2008, 88: 125–127.
on the unit ball. Ars Combinatoria 2008, 88: 125–127. . Journal of the Korean Mathematical Society 2005,42(1):111–127.
. Journal of the Korean Mathematical Society 2005,42(1):111–127. -Bloch spaces and the logarithmic Bloch. Journal of Computational Analysis and Applications 2008,10(2):243–252.
-Bloch spaces and the logarithmic Bloch. Journal of Computational Analysis and Applications 2008,10(2):243–252. spaces to
spaces to  spaces to spaces. Abstract and Applied Analysis 2009, 2009:-14.
spaces to spaces. Abstract and Applied Analysis 2009, 2009:-14. . Complex Variables and Elliptic Equations 2007,52(6):495–517. 10.1080/17476930701235225
. Complex Variables and Elliptic Equations 2007,52(6):495–517. 10.1080/17476930701235225