- Research Article
- Open access
- Published:
Generalized Vector Complementarity Problems with Moving Cones
Journal of Inequalities and Applications volume 2009, Article number: 617185 (2009)
Abstract
We introduce and discuss a class of generalized vector complementarity problems with moving cones. We discuss the existence results for the generalized vector complementarity problem under inclusive type condition. We obtain equivalence results between the generalized vector complementarity problem, the generalized vector variational inequality problem, and other related problems. The theorems presented here improve, extend, and develop some earlier and very recent results in the literature.
1. Introduction and Preliminaries
It is well known that vector variational inequalities were initially studied by Giannessi [1] and ever since have been widely studied in infinite-dimensional spaces see, for example, [2–8] and the references therein.
Very recently, Huang et al. [9] considered a class of vector complementarity problems with moving cones. They established existence results of a solution for this class of vector complementarity problems under an inclusive type condition. They also obtained some equivalence results among a vector complementarity problem, a vector variational inequality problem, a vector optimization problem, a weak minimal element problem, and a vector unilateral optimization problem in ordered Banach spaces. Their results generalized the main results in [4].
The purpose of this paper is to introduce and discuss a class of generalized vector complementarity problems with moving cones which is a variable ordering relation. We derive existence of a solution for this class of generalized vector complementarity problems under an inclusive type condition. This inclusive condition requires that any two of the family of closed and convex cones satisfy an inclusion relation so long as their corresponding variables satisfy certain conditions. We also obtain some equivalence results among a generalized vector complementarity problem, a generalized vector variational inequality problem, a generalized vector optimization problem, a generalized weak minimal element problem, and a generalized vector unilateral optimization problem under some monotonicity conditions and some inclusive type conditions in ordered Banach spaces. The theorems presented in this paper improve, extend, and develop some earlier and very recent results in the literature including [4, 9].
Let  be a Banach space, and
be a Banach space, and  a subset of
a subset of  . The topological interior of a subset
. The topological interior of a subset  in
in  is denoted by
is denoted by  . A nonempty subset
. A nonempty subset  in
in  is called a convex cone if
is called a convex cone if  , and
, and  for any
for any  . The relations
. The relations  and
and  in
in  are defined as
are defined as  if
if  and
and  if
if  , for any
, for any  . Similarly, we can define the relations
. Similarly, we can define the relations  and
and  if we replace the set
if we replace the set  by
by  .
.  is called a pointed cone if
is called a pointed cone if  is a cone and
is a cone and  .
.
Let  be the space of all continuous linear mappings from
be the space of all continuous linear mappings from  to
to  . We denote the value of
. We denote the value of  at
at  by
by  .
.
Let  be two Banach spaces, and
be two Banach spaces, and  a set-valued mapping such that, for each
a set-valued mapping such that, for each  ,
,  is a proper closed convex and pointed cone with apex at the origin and
is a proper closed convex and pointed cone with apex at the origin and  , and
, and  . Very recently, Huang et al. [9] introduced the following three kinds of vector complementarity problems.
. Very recently, Huang et al. [9] introduced the following three kinds of vector complementarity problems.
(Weak) vector complementarity problem (VCP): finding  such that
such that

Positive vector complementarity problem (PVCP): finding  such that
such that

Strong vector complementarity problem (SVCP): finding  such that
such that

We remark that if  for all
for all  , where
, where  is a closed, pointed, and convex cone in
is a closed, pointed, and convex cone in  with nonempty interior
with nonempty interior  , then (VCP), (PVCP) and (SVCP) reduce to the problems considered in Chen and Yang [4]. In [9], they actually only studied the first two kinds complementarity problems. For the existence results of (SVCP), we refer the reader to our recent results [Submitted, On the
, then (VCP), (PVCP) and (SVCP) reduce to the problems considered in Chen and Yang [4]. In [9], they actually only studied the first two kinds complementarity problems. For the existence results of (SVCP), we refer the reader to our recent results [Submitted, On the  -implicit vector complementarity problem].
-implicit vector complementarity problem].
Motivated and inspired by the above three kinds of vector complementarity problems, in this paper we introduce three kinds of generalized vector complementarity problems. Let  be two Banach spaces, and
be two Banach spaces, and  a set-valued mapping such that, for each
a set-valued mapping such that, for each  ,
,  is a proper closed convex and pointed cone with apex at the origin and
is a proper closed convex and pointed cone with apex at the origin and  , let
, let  be a single-valued mapping, and
be a single-valued mapping, and  a set-valued mapping, where
a set-valued mapping, where  is a collection of all nonempty subsets of
is a collection of all nonempty subsets of  . We consider the following three kinds of generalized vector complementarity problems.
. We consider the following three kinds of generalized vector complementarity problems.
(Weak) generalized vector complementarity problem (GVCP): finding  and
and  such that
such that

Generalized positive vector complementarity problem (GPVCP): finding  and
and  such that
such that

Generalized strong vector complementarity problem (GSVCP): finding  and
and  such that
such that

We remark that if  the identity mapping of
the identity mapping of  , and
, and  is a single-valued mapping, then three kinds of generalized vector complementarity problems reduce to three kinds of vector complementarity problems in Huang et al. [9], respectively.
is a single-valued mapping, then three kinds of generalized vector complementarity problems reduce to three kinds of vector complementarity problems in Huang et al. [9], respectively.
2. Existence of a Solution for GVCP
Huang et al. [9] established some equivalence results between the positive vector complementarity problem and the vector extremum problem and also sufficient conditions for the existence of a solution of the vector extremum problem. In this section, we extend their results to the cases involving the set-valued mappings.
Let  be an arbitrary real Hausdorff topological vector spaces, and
be an arbitrary real Hausdorff topological vector spaces, and  a Banach space.
a Banach space.  denotes the space of all continuous linear mappings from
denotes the space of all continuous linear mappings from  to
to  . Let
. Let  be a nonempty set of
be a nonempty set of  , and
, and  a set-valued mapping such that, for each
a set-valued mapping such that, for each  ,
,  is a proper closed convex and pointed cone with apex at the origin and
is a proper closed convex and pointed cone with apex at the origin and  . Let
. Let  be a subset of
be a subset of  . For each
. For each  , a point
, a point  is called a minimal point of
is called a minimal point of  with respect to the cone
with respect to the cone  if
if  ;
;  is the set of all minimal points of
is the set of all minimal points of  with respect to the cone
with respect to the cone  ; a point
; a point  is called a weakly minimal point of
is called a weakly minimal point of  with respect to the cone
with respect to the cone  if
if  ;
;  is the set of all weakly minimal points of
is the set of all weakly minimal points of  with respect to the cone
with respect to the cone  , we refer the reader to [10] for more detail.
, we refer the reader to [10] for more detail.
Let  be a single-valued mapping and let
be a single-valued mapping and let  be a set-valued mapping. Now, we consider the following generalized vector complementarity problem (GVCP). Find
be a set-valued mapping. Now, we consider the following generalized vector complementarity problem (GVCP). Find  and
and  such that
such that

A feasible set of (GVCP) is

We consider the following generalized vector optimization problem (GVOP):

A point  is called a weakly minimal solution of (GVOP) with respect to the cone
is called a weakly minimal solution of (GVOP) with respect to the cone  , if
, if  is a weakly minimal point of (GVOP) with respect to the cone
is a weakly minimal point of (GVOP) with respect to the cone  , that is,
, that is,  . We denote the set of all weakly minimal solutions of (GVOP) with respect to the cone
. We denote the set of all weakly minimal solutions of (GVOP) with respect to the cone  by
by  and the set of all weakly minimal solutions of (GVOP) by
and the set of all weakly minimal solutions of (GVOP) by  , that is,
, that is,

Theorem 2.1.
If  and, for some
and, for some  , there exists
, there exists  such that
such that  , then the generalized vector complementarity problem (GVCP) is solvable.
, then the generalized vector complementarity problem (GVCP) is solvable.
Proof.
Let  and
and  . Then
. Then  , and
, and

It follows that  is a solution of (GVCP). This completes the proof.
is a solution of (GVCP). This completes the proof.
We remark that if  the identity mapping of
the identity mapping of  and
and  is a single-valued mapping from
is a single-valued mapping from  to
to  , then Theorem 2.1 coincides with Theorem
, then Theorem 2.1 coincides with Theorem  in Huang et al. [9].
in Huang et al. [9].
Definition 2.2.
Let  ,
,  be two set-valued mappings with
be two set-valued mappings with  for every
for every  ,
,  a single-valued mapping, and
a single-valued mapping, and  a subset of
a subset of  . We say that
. We say that  is inclusive with respect to
is inclusive with respect to  if for any
if for any  ,
,

It is easy to see that, if  for all
for all  , where
, where  is a closed, pointed, and convex cone in
is a closed, pointed, and convex cone in  , then
, then  is inclusive with respect to
is inclusive with respect to  .
.
Example 2.3.
Let  be the identity mapping of
be the identity mapping of  . For each
. For each  , define
, define

and, for each  ,
,

Also, define

Then it is easy to see that  is inclusive with respect to
is inclusive with respect to  . Indeed, for any
. Indeed, for any  with
with  , and
, and  , if
, if  , then
, then  and so
and so  . Therefore,
. Therefore,  and
and  is inclusive with respect to
is inclusive with respect to  .
.
Theorem 2.4.
Suppose that  is inclusive with respect to
is inclusive with respect to  . If there exist at most a finite number of solutions for (GVCP), then (GVCP) is solvable if and only if
. If there exist at most a finite number of solutions for (GVCP), then (GVCP) is solvable if and only if  , and there exists
, and there exists  such that
such that  .
.
Proof.
Let  be a solution of (GVCP). Then there exists
be a solution of (GVCP). Then there exists  such that
such that

If  , then
, then

and hence the conclusion holds. If  , by the definition of a weakly minimal solution, there exists
, by the definition of a weakly minimal solution, there exists  such that
such that

This implies that

Since  , and
, and  is inclusive with respect to
is inclusive with respect to  , it follows that
, it follows that  and this implies that
and this implies that

Thus,  is a solution of (GVCP) and
is a solution of (GVCP) and  . Continuing this process, there exists
. Continuing this process, there exists  such that
such that  is a solution of (GVCP) and
is a solution of (GVCP) and  , since (GVCP) has at most a finite number of solutions. Thus,
, since (GVCP) has at most a finite number of solutions. Thus,  and
and

Combining this result and Theorem 2.1, we have the conclusion of the theorem.
Remark 2.5.
-
(1)
If
 the identity mapping of
the identity mapping of  ,
, 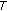 is a single-valued mapping from
is a single-valued mapping from  to
to  , and
, and 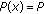 for all
for all 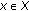 , where
, where  is a closed, pointed, and convex cone in
is a closed, pointed, and convex cone in  , then
, then  satisfies the inclusive condition with respect to
satisfies the inclusive condition with respect to 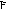 and Theorem 2.4 reduces to Theorem
and Theorem 2.4 reduces to Theorem 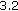 of Chen and Yang [4].
of Chen and Yang [4]. -
(2)
If
 the identity mapping of
the identity mapping of 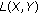 and
and 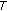 is a single-valued mapping from
is a single-valued mapping from  to
to  , then Theorem 2.4 coincides with Theorem
, then Theorem 2.4 coincides with Theorem  of Huang et al. [9].
of Huang et al. [9].
We next consider the generalized positive vector complementarity problem (GPVCP). Finding  and
and  such that
such that

Let

Consider the following generalized vector optimization problem (GVOP) to be
to be

We denote the set of all minimal points of (GVOP)0 with respect to the cone  by
by  , that is,
, that is,  , and denote the set of all minimal points of (GVOP)
, and denote the set of all minimal points of (GVOP) by
by

Using a similar argument of Theorem 2.1, we have the following results of solvability for (GPVCP).
Theorem 2.6.
If  and there exists
and there exists  such that
such that  , then (GPVCP) is solvable.
, then (GPVCP) is solvable.
Theorem 2.7.
Suppose that  is inclusive with respect to
is inclusive with respect to  . If there exist at most a finite number of solutions of (GPVCP), then (GPVCP) is solvable if and only if
. If there exist at most a finite number of solutions of (GPVCP), then (GPVCP) is solvable if and only if  , and there exists
, and there exists  such that
such that  .
.
One remarks that If  the identity mapping of
the identity mapping of  and
and  is a single-valued mapping from
is a single-valued mapping from  to
to  , then Theorems 2.6 and 2.7 coincide with Theorems
, then Theorems 2.6 and 2.7 coincide with Theorems  and
and  of Huang et al. [9], respectively.
of Huang et al. [9], respectively.
3. Equivalences between Generalized Vector Complementarity
3.1. Problems and Generalized Weak Minimal Element Problems
Let  be two Banach spaces, and
be two Banach spaces, and  a set-valued mapping such that, for each
a set-valued mapping such that, for each  ,
,  is a proper closed convex and pointed cone with apex at the origin and
is a proper closed convex and pointed cone with apex at the origin and  , let
, let  be a single-valued mapping, and
be a single-valued mapping, and  a set-valued mapping, where
a set-valued mapping, where  is a collection of all nonempty subsets of
is a collection of all nonempty subsets of  , and
, and  a given operator.
a given operator.
Define the feasible set associated with  and
and 

We now consider the following five problems.
(i)The generalized vector optimization problem (GVOP) : for a given
: for a given  , finding
, finding  such that
such that

(ii)The generalized weak minimal element problem (GWMEP): finding  such that
such that  .
.
(iii)The generalized vector complementarity problem (GVCP): finding  such that
such that  where
where  is associated with
is associated with  in the definition of
in the definition of  .
.
(iv)The generalized vector variational inequality problem (GVVIP): finding  and
and  such that
such that

(v)The generalized vector unilateral optimization problem (GVUOP): finding  such that
such that  .
.
We remark that if  the identity mapping of
the identity mapping of  and
and  is a single-valued mapping from
is a single-valued mapping from  to
to  , then the (GVOP)
, then the (GVOP) , (GWMEP), (GVCP), (GVVIP), and (GVUOP) reduce to Huang, et al.'s problems (VOP)
, (GWMEP), (GVCP), (GVVIP), and (GVUOP) reduce to Huang, et al.'s problems (VOP) , (WMEP), (VCP), (VVIP), and (VUOP), respectively; see [9] for more details.
, (WMEP), (VCP), (VVIP), and (VUOP), respectively; see [9] for more details.
Definition 3.1 (see [4]).
A linear operator  is called weakly positive if, for any
is called weakly positive if, for any  implies that
implies that  .
.
Definition 3.2.
Let  and
and  be two Banach spaces and
be two Banach spaces and  a linear operator from
a linear operator from  to
to  . If the image of any bounded set in
. If the image of any bounded set in  is a self-sequentially compact set in
is a self-sequentially compact set in  , then
, then  is called completely continuous.
is called completely continuous.
A mapping  is said to be convex if
is said to be convex if

for all  and
and  .
.
Definition 3.3.
Let  and
and  be two mappings.
be two mappings.  is said to be
is said to be  -subdifferentiable at
-subdifferentiable at  if there exists
if there exists  such that
such that

If  is
is  -subdifferentiable at
-subdifferentiable at  , then we define the
, then we define the  -subdifferential of
-subdifferential of  at
at  as follows:
as follows:

If  is
is  -subdifferentiable at each
-subdifferentiable at each  , then we say that
, then we say that  is
is  -subdifferentiable on
-subdifferentiable on  .
.
Remark 3.4.
We note that as the mentions in [9], if  and
and  are two Banach spaces, a mapping
are two Banach spaces, a mapping  is Fréchet differentiable at
is Fréchet differentiable at  if there exists a linear bounded operator
if there exists a linear bounded operator  such that
such that

where  is said to be the Fréchet derivative of
is said to be the Fréchet derivative of  at
at  . The mapping
. The mapping  is said to be Fréchet differentiable on
is said to be Fréchet differentiable on  if
if  is Fréchet differentiable at each point of
is Fréchet differentiable at each point of  . If
. If  is convex and Fréchet differentiable on
is convex and Fréchet differentiable on  , then
, then

If  is Fréchet differentiable at
is Fréchet differentiable at  , then
, then  is
is  -subdifferentiable at
-subdifferentiable at  and
and  .
.
If  is
is  -subdifferentiable on
-subdifferentiable on  , then for each
, then for each  we have
we have

Definition 3.5.
Let  be a Banach space,
be a Banach space,  a proper closed convex and pointed cone with apex at the origin and
a proper closed convex and pointed cone with apex at the origin and  . The norm
. The norm  in
in  is called strictly monotonically increasing on
is called strictly monotonically increasing on  [9] if, for each
[9] if, for each  ,
,

For the example of the strictly monotonically increasing property, we refer the reader to [9, Example  ].
].
Theorem 3.6.
Let  be two Banach spaces,
be two Banach spaces,  a proper closed convex and pointed cone with apex at the origin and
a proper closed convex and pointed cone with apex at the origin and  , and
, and  a set-valued mapping with closed, convex, and pointed cones values such that
a set-valued mapping with closed, convex, and pointed cones values such that  for all
for all  . Suppose that
. Suppose that
(1) is the
is the  -subdifferential of a convex operator
-subdifferential of a convex operator  ;
;
(2) is a weakly positive linear operator;
is a weakly positive linear operator;
(3)there exists  such that
such that  is one to one and completely continuous, where
is one to one and completely continuous, where  is associated with
is associated with  in the definition of
in the definition of  ;
;
(4) is a topological dual space of a real normed space and the norm
is a topological dual space of a real normed space and the norm  in
in  is strictly monotonically increasing on
is strictly monotonically increasing on  .
.
If (GVVIP) is solvable, then (GVOP) , (GWMEP), (GVCP), and (GVUOP) are also solvable.
, (GWMEP), (GVCP), and (GVUOP) are also solvable.
Corollary 3.7 (see [9, Theorem  ]).
]).
Let  be two Banach spaces,
be two Banach spaces,  a proper closed convex and pointed cone with apex at the origin and
a proper closed convex and pointed cone with apex at the origin and  , and
, and  that a family of closed, pointed, and convex cones in
that a family of closed, pointed, and convex cones in  such that
such that  for all
for all  . Suppose that
. Suppose that
(1) is the Fréchet derivative of a convex operator
is the Fréchet derivative of a convex operator  ;
;
(2) is a weakly positive linear operator;
is a weakly positive linear operator;
(3)there exists  such that
such that  is one to one and completely continuous, where
is one to one and completely continuous, where  ;
;
(4) is a topological dual space of a real normed space and the norm
is a topological dual space of a real normed space and the norm  in
in  is strictly monotonically increasing on
is strictly monotonically increasing on  .
.
If (VVIP) is solvable, then (VOP) , (WMEP), (VCP), and (VUOP) are also solvable.
, (WMEP), (VCP), and (VUOP) are also solvable.
Proof.
Since  the identity mapping of
the identity mapping of  and
and  is a single-valued mapping from
is a single-valued mapping from  to
to  , we have
, we have

Utilizing Theorem 3.6, we immediately obtain the desired conclusion.
Remark 3.8.
If  for all
for all  , where
, where  is a closed, pointed, and convex cone in
is a closed, pointed, and convex cone in  , then Corollary 3.7 coincides with Theorem
, then Corollary 3.7 coincides with Theorem  of Chen and Yang [4].
of Chen and Yang [4].
We need the following propositions to prove Theorem 3.6.
Proposition 3.9.
Let  and
and  be two mappings, and let
be two mappings, and let  be the
be the  -subdifferential of
-subdifferential of  . Then
. Then  solves (GVUOP) which implies that
solves (GVUOP) which implies that  solves (GVVIP). If in addition,
solves (GVVIP). If in addition,  is a convex mapping, then conversely,
is a convex mapping, then conversely,  solves (GVVIP) which implies that
solves (GVVIP) which implies that  solves (GVUOP).
solves (GVUOP).
Proof.
Let  be a solution of (GVUOP). Then
be a solution of (GVUOP). Then  and
and  , that is,
, that is,  for all
for all  . Since
. Since  is a convex cone,
is a convex cone,

Also, since  is
is  -subdifferentiable on
-subdifferentiable on  , it follows that for all
, it follows that for all 

This implies that

and hence

Thus,  solves (GVVIP).
solves (GVVIP).
Conversely, let  solve (GVVIP). Then there exists
solve (GVVIP). Then there exists  such that
such that

Since  is
is  -subdifferentiable on
-subdifferentiable on  , we have for all
, we have for all 

and hence

This implies that

Consequently,  solves (GVUOP). This completes the proof.
solves (GVUOP). This completes the proof.
Proposition 3.10.
If  solves (GVVIP), then
solves (GVVIP), then  also solves (GVCP). Conversely, if
also solves (GVCP). Conversely, if  for all
for all  , then
, then  solves (GVCP) which implies that
solves (GVCP) which implies that  solves (GVVIP).
solves (GVVIP).
Proof.
Let  be a solution of (GVVIP). Then there exists
be a solution of (GVVIP). Then there exists  such that
such that

Letting  , we get
, we get  . For
. For  with any
with any  , we have
, we have

Thus,  is a solution of the (GVCP).
is a solution of the (GVCP).
Conversely, let  solve the (GVCP). Then there exists
solve the (GVCP). Then there exists  such that
such that

This implies

and so

This completes the proof.
Proposition 3.11.
Let  be a weakly positive linear operator. Then
be a weakly positive linear operator. Then  solves (GWMEP) which implies that
solves (GWMEP) which implies that  solves (GVOP)
solves (GVOP) .
.
Proof.
Let  be a solution of (GWMEP). Then
be a solution of (GWMEP). Then  and
and  , that is, for any
, that is, for any  ,
,  . Since
. Since  is a weakly positive linear operator, it follows that
is a weakly positive linear operator, it follows that  and so
and so

hence  solves (GVOP)
solves (GVOP) . This completes the proof.
. This completes the proof.
Definition 3.12 (see [9]).
Let  be a Banach space,
be a Banach space,  a proper closed convex and pointed cone with apex at the origin and
a proper closed convex and pointed cone with apex at the origin and  ,
,  a nonempty subset of
a nonempty subset of  .
.
(1)If, for some  , then
, then  is called a section of the set
is called a section of the set  .
.
(2) is called weakly closed if
is called weakly closed if  for all
for all  , then
, then  .
.
(3) is called bounded below if there exists a point
is called bounded below if there exists a point  in
in  such that
such that  .
.
Lemma 3.13 (see [11]).
Let  be a Banach space,
be a Banach space,  a proper closed convex and pointed cone with apex at the origin and
a proper closed convex and pointed cone with apex at the origin and  ,
,  a nonempty subset of
a nonempty subset of  and
and  the topological dual space of a real normed space
the topological dual space of a real normed space  . Suppose there exists
. Suppose there exists  such that the section
such that the section  is weakly closed and bounded below and the norm
is weakly closed and bounded below and the norm  in
in  is strictly monotonically increasing, then the set
is strictly monotonically increasing, then the set  has at least one weakly minimal point.
has at least one weakly minimal point.
Lemma 3.14.
If (GVVIP) is solvable, then the feasible set  is nonempty.
is nonempty.
Proof.
Let  be a solution of (GVVIP). Then there exists
be a solution of (GVVIP). Then there exists  such that
such that

Taking  with any
with any  , we know that
, we know that  and
and

Thus,  . This completes the proof.
. This completes the proof.
Let  be a Banach space,
be a Banach space,  a proper closed convex and pointed cone with apex at the origin and
a proper closed convex and pointed cone with apex at the origin and  . For any
. For any  ,
,  is called an order interval.
is called an order interval.
Lemma 3.15 (see [4]).
Let  be a Banach space,
be a Banach space,  a proper closed convex and pointed cone with apex at the origin and
a proper closed convex and pointed cone with apex at the origin and  . If the norm
. If the norm  in
in  is strictly monotonically increasing, then the order intervals in
is strictly monotonically increasing, then the order intervals in  are bounded.
are bounded.
Proposition 3.16.
Suppose that (GVVIP) is solvable and
(1)there exists  in
in  such that
such that  is one to one and completely continuous, where
is one to one and completely continuous, where  is associated with
is associated with  in the definition of
in the definition of  ;
;
(2) is the topological dual space of a real normed space
is the topological dual space of a real normed space  and the norm
and the norm  in
in  is strictly monotonically increasing.
is strictly monotonically increasing.
Then (GWMEP) has at least one solution.
Proof.
By the assumption and Lemma 3.14,  . Let
. Let  be a point such that
be a point such that  is one to one and completely continuous, where
is one to one and completely continuous, where  is associated with
is associated with  in the definition of
in the definition of  , and let
, and let  with
with  (weakly). Since
(weakly). Since

by Lemma 3.15,  is bounded and so is
is bounded and so is  . Since
. Since  is completely continuous,
is completely continuous,  is a self-sequentially compact set and so
is a self-sequentially compact set and so  implies that there exists a subsequence
implies that there exists a subsequence  which converges to
which converges to  . We get a point
. We get a point  such that
such that

On the other hand, since  (weakly) and
(weakly) and  is completely continuous,
is completely continuous,

By the uniqueness of the limit, we get  . Since
. Since  is one to one,
is one to one,  , and so
, and so  . Since
. Since  is weakly closed, it follows from Lemma 3.13 that
is weakly closed, it follows from Lemma 3.13 that  has a weakly minimal point
has a weakly minimal point  such that
such that  for all
for all  . Therefore, (GWMEP) has at least one solution. This completes the proof.
. Therefore, (GWMEP) has at least one solution. This completes the proof.
Definition 3.17.
Let  be two Banach spaces,
be two Banach spaces,  a proper closed convex and pointed cone with apex at the origin and
a proper closed convex and pointed cone with apex at the origin and  , and
, and  a set-valued mapping with closed, convex and pointed cones values such that
a set-valued mapping with closed, convex and pointed cones values such that  for all
for all  . Let
. Let  be a single-valued mapping and
be a single-valued mapping and  a set-valued mapping.
a set-valued mapping.  is called
is called  -positive if
-positive if

We now consider the generalized positive vector complementarity problem (GPVCP). Finding  and
and  such that
such that

The feasible set related to (GPVCP) is defined as

Let us consider the following problems.
The generalized vector optimization problem  : finding
: finding  such that
such that  .
.
The generalized weak minimal element problem  : finding
: finding  such that
such that  .
.
The generalized positive vector complementarity problem (GPVCP): finding  such that
such that

where  is associated with
is associated with  in the definition of
in the definition of  .
.
The generalized vector variational inequality problem (GVVIP): finding  and
and  such that
such that

The generalized vector unilateral optimization problem (GVUOP): for a given mapping  , finding
, finding  such that
such that  .
.
Definition 3.18.
A set-valued mapping  is said to be
is said to be  -strictly monotone where
-strictly monotone where  is single-valued, if
is single-valued, if

Definition 3.19 (see [9]).
We say that  satisfies an inclusive condition if, for any
satisfies an inclusive condition if, for any  ,
,

It is easy to see that, if  for all
for all  , where
, where  is a closed, pointed, and convex cone in
is a closed, pointed, and convex cone in  , then
, then  satisfies the inclusive condition.
satisfies the inclusive condition.
Example 3.20.
Let  , and
, and

for all  . Then it is easy to check that
. Then it is easy to check that  satisfies the inclusive condition.
satisfies the inclusive condition.
Proposition 3.21.
Let  be
be  -strictly monotone and
-strictly monotone and  a solution of (GPVCP). If
a solution of (GPVCP). If  satisfies the inclusive condition, then
satisfies the inclusive condition, then  is a weakly minimal point of
is a weakly minimal point of  (i.e.,
(i.e.,  solves (GWMEP)
solves (GWMEP) ).
).
Proof.
It is easy to see that  . If
. If  (where
(where  denotes the boundary of
denotes the boundary of  ), then
), then  solves (GWMEP)
solves (GWMEP) . Otherwise, there exists
. Otherwise, there exists  such that
such that  and so
and so

which is a contradiction. If  , by the
, by the  -strict monotonicity of
-strict monotonicity of  ,
,

Suppose  . Since
. Since  is
is  -positive,
-positive,  and
and

By the assumption, we get  and so
and so

It follows that

and thus

for some  . This implies
. This implies

Since  and
and  ,
,

and so

which leads to a contradiction. Therefore,  does not hold, that is,
does not hold, that is,  for all
for all  . It follows that
. It follows that  solves (GWMEP)
solves (GWMEP) . This completes the proof.
. This completes the proof.
Proposition 3.22.
If  solves (GPVCP), then
solves (GPVCP), then  also solves (GVVIP).
also solves (GVVIP).
Proof.
Suppose that  solves (GPVCP). Then
solves (GPVCP). Then  and there exists
and there exists  such that
such that

If  , then
, then

and so

which is a contradiction. It follows that

and  solves (GVVIP). This completes the proof.
solves (GVVIP). This completes the proof.
Similarly, we can obtain other equivalence conditions. We have the following theorem.
Theorem 3.23.
Let  be two Banach spaces,
be two Banach spaces,  a proper closed convex and pointed cone with apex at the origin and
a proper closed convex and pointed cone with apex at the origin and  , and
, and  a family of closed, pointed, and convex cones in
a family of closed, pointed, and convex cones in  such that
such that  for all
for all  . Suppose that
. Suppose that  satisfies the inclusive condition and
satisfies the inclusive condition and
(1) is the
is the  -subdifferential of the convex operator
-subdifferential of the convex operator  ;
;
(2) is a weakly positive linear operator;
is a weakly positive linear operator;
(3) is
is  -strictly monotone.
-strictly monotone.
If (GPVCP) is solvable, then (GVOP) , (GWMEP)
, (GWMEP) , (GPVCP), (GVVIP), and (GVUOP) have at least a common solution.
, (GPVCP), (GVVIP), and (GVUOP) have at least a common solution.
Corollary 3.24 (see [9, Theorem  ]).
]).
Let  be two Banach spaces,
be two Banach spaces,  a proper closed convex and pointed cone with apex at the origin and
a proper closed convex and pointed cone with apex at the origin and  , and
, and  a family of closed, pointed, and convex cones in
a family of closed, pointed, and convex cones in  such that
such that  for all
for all  . Suppose that
. Suppose that  satisfies the inclusive condition and
satisfies the inclusive condition and
(1) is the Fréchet derivative of the convex operator
is the Fréchet derivative of the convex operator  ;
;
(2) is a weakly positive linear operator;
is a weakly positive linear operator;
(3) is strictly monotone.
is strictly monotone.
If (PVCP) is solvable, then (VOP) , (WMEP)
, (WMEP) , (PVCP), (VVIP), and (VUOP) have at least a common solution.
, (PVCP), (VVIP), and (VUOP) have at least a common solution.
Proof.
Note that  the identity mapping of
the identity mapping of  and
and  is a single-valued mapping from
is a single-valued mapping from  to
to  . From Theorem 3.23, we immediately obtain the desired conclusion.
. From Theorem 3.23, we immediately obtain the desired conclusion.
Remark 3.25.
If  for all
for all  , where
, where  is a closed, pointed, and convex cone in
is a closed, pointed, and convex cone in  , then Corollary 3.24 coincides with Theorem
, then Corollary 3.24 coincides with Theorem  of Chen and Yang [4].
of Chen and Yang [4].
References
Giannessi F: Theorems of alternative, quadratic programs and complementarity problems. In Variational Inequalities and Complementarity Problems (Proc. Internat. School, Erice, 1978). Edited by: Cottle RW, Giannessi F, Lions JL. John Wiley & Sons, Chichester, UK; 1980:151–186.
Ansari QH, Lai TC, Yao JC: On the equivalence of extended generalized complementarity and generalized least-element problems. Journal of Optimization Theory and Applications 1999,102(2):277–288. 10.1023/A:1021724306242
Chen GY: Existence of solutions for a vector variational inequality: an extension of the Hartmann-Stampacchia theorem. Journal of Optimization Theory and Applications 1992,74(3):445–456. 10.1007/BF00940320
Chen GY, Yang XQ: The vector complementary problem and its equivalences with the weak minimal element in ordered spaces. Journal of Mathematical Analysis and Applications 1990,153(1):136–158. 10.1016/0022-247X(90)90270-P
Fang Y-P, Huang N-J: The vector
 -complementary problems with demipseudomonotone mappings in Banach spaces. Applied Mathematics Letters 2003,16(7):1019–1024. 10.1016/S0893-9659(03)90089-9
-complementary problems with demipseudomonotone mappings in Banach spaces. Applied Mathematics Letters 2003,16(7):1019–1024. 10.1016/S0893-9659(03)90089-9Giannessi F (Ed): Vector Variational Inequalities and Vector Equilibria: Mathematical Theories, Nonconvex Optimization and Its Applications. Volume 38. Kluwer Academic Publishers, Dordrecht, The Netherlands; 2000:xiv+523.
Huang N-J, Gao C-J: Some generalized vector variational inequalities and complementarity problems for multivalued mappings. Applied Mathematics Letters 2003,16(7):1003–1010. 10.1016/S0893-9659(03)90087-5
Yang XQ: Vector complementarity and minimal element problems. Journal of Optimization Theory and Applications 1993,77(3):483–495. 10.1007/BF00940446
Huang NJ, Yang XQ, Chan WK: Vector complementarity problems with a variable ordering relation. European Journal of Operational Research 2007,176(1):15–26. 10.1016/j.ejor.2005.06.046
Lin YC: On
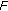 -implicit generalized vector variational inequalities. Journal of Optimization Theory and Applications 2009,142(3):557–568. 10.1007/s10957-009-9543-2
-implicit generalized vector variational inequalities. Journal of Optimization Theory and Applications 2009,142(3):557–568. 10.1007/s10957-009-9543-2Jahn J: Existence theorems in vector optimization. Journal of Optimization Theory and Applications 1986,50(3):397–406. 10.1007/BF00938627
Acknowledgments
This research of the first author was partially supported by the National Science Foundation of China (10771141), Ph.D. Program Foundation of Ministry of Education of China (20070270004), and Science and Technology Commission of Shanghai Municipality Grant (075105118). The second author would like to thank the support of NSC97-2115-M-039-001- Grant from the National Science Council of Taiwan.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Ceng, LC., Lin, YC. Generalized Vector Complementarity Problems with Moving Cones. J Inequal Appl 2009, 617185 (2009). https://doi.org/10.1155/2009/617185
Received:
Accepted:
Published:
DOI: https://doi.org/10.1155/2009/617185
 the identity mapping of
the identity mapping of  ,
, 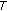 is a single-valued mapping from
is a single-valued mapping from  to
to  , and
, and 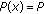 for all
for all 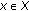 , where
, where  is a closed, pointed, and convex cone in
is a closed, pointed, and convex cone in  , then
, then  satisfies the inclusive condition with respect to
satisfies the inclusive condition with respect to 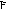 and Theorem 2.4 reduces to Theorem
and Theorem 2.4 reduces to Theorem 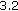 of Chen and Yang [
of Chen and Yang [ the identity mapping of
the identity mapping of 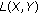 and
and 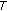 is a single-valued mapping from
is a single-valued mapping from  to
to  , then Theorem 2.4 coincides with Theorem
, then Theorem 2.4 coincides with Theorem  of Huang et al. [
of Huang et al. [ -complementary problems with demipseudomonotone mappings in Banach spaces. Applied Mathematics Letters 2003,16(7):1019–1024. 10.1016/S0893-9659(03)90089-9
-complementary problems with demipseudomonotone mappings in Banach spaces. Applied Mathematics Letters 2003,16(7):1019–1024. 10.1016/S0893-9659(03)90089-9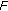 -implicit generalized vector variational inequalities. Journal of Optimization Theory and Applications 2009,142(3):557–568. 10.1007/s10957-009-9543-2
-implicit generalized vector variational inequalities. Journal of Optimization Theory and Applications 2009,142(3):557–568. 10.1007/s10957-009-9543-2