- Research Article
- Open access
- Published:
Superstability of Generalized Multiplicative Functionals
Journal of Inequalities and Applications volume 2009, Article number: 486375 (2009)
Abstract
Let  be a set with a binary operation
be a set with a binary operation  such that, for each
such that, for each  , either
, either  , or
, or  . We show the superstability of the functional equation
. We show the superstability of the functional equation  . More explicitly, if
. More explicitly, if  and
and  satisfies
satisfies  for each
for each  , then
, then  for all
for all  , or
, or  for all
for all  . In the latter case, the constant
. In the latter case, the constant  is the best possible.
is the best possible.
1. Introduction
It seems that the stability problem of functional equations had been first raised by S. M. Ulam (cf. [1, Chapter VI]). "For what metric groups  is it true that an
is it true that an  -automorphism of
-automorphism of  is necessarily near to a strict automorphism? (An
is necessarily near to a strict automorphism? (An  -automorphism of
-automorphism of  means a transformation
means a transformation  of
of  into itself such that
into itself such that  for all
for all  .)" D. H. Hyers [2] gave an affirmative answer to the problem: if
.)" D. H. Hyers [2] gave an affirmative answer to the problem: if  and
and  is a mapping between two real Banach spaces
is a mapping between two real Banach spaces  and
and  satisfying
satisfying  for all
for all  , then there exists a unique additive mapping
, then there exists a unique additive mapping  such that
such that  for all
for all  . If, in addition, the mapping
. If, in addition, the mapping  is continuous for each fixed
is continuous for each fixed  , then
, then  is linear. This result is called Hyers-Ulam stability of the additive Cauchy equation
is linear. This result is called Hyers-Ulam stability of the additive Cauchy equation  . J. A. Baker [3, Theorem
. J. A. Baker [3, Theorem  ] considered stability of the multiplicative Cauchy equation
] considered stability of the multiplicative Cauchy equation  : if
: if  and
and  is a complex valued function on a semigroup
is a complex valued function on a semigroup  such that
such that  for all
for all  , then
, then  is multiplicative, or
is multiplicative, or  for all
for all  . This result is called superstability of the functional equation
. This result is called superstability of the functional equation  . Recently, A. Najdecki [4, Theorem
. Recently, A. Najdecki [4, Theorem  ] proved the superstability of the functional equation
] proved the superstability of the functional equation  : if
: if  ,
,  is a (real or complex valued) functional from a commutative semigroup
is a (real or complex valued) functional from a commutative semigroup  and
and  is a mapping from
is a mapping from  into itself such that
into itself such that  for all
for all  , then
, then  holds for all
holds for all  , or
, or  is bounded.
is bounded.
In this paper, we show that superstability of the functional equation  holds for a set
holds for a set  with a binary operation
with a binary operation  under an additional assumption.
under an additional assumption.
2. Main Result
Theorem 2.1.
Let  and
and  a set with a binary operation
a set with a binary operation  such that, for each
such that, for each  , either
, either

If  satisfies
satisfies

then  for all
for all  , or
, or  for all
for all  . In the latter case, the constant
. In the latter case, the constant  is the best possible.
is the best possible.
Proof.
Let  be a functional satisfying (2.2). Suppose that
be a functional satisfying (2.2). Suppose that  is bounded. There exists a constant
is bounded. There exists a constant  such that
such that  for all
for all  . Set
. Set  . By (2.2), we have, for each
. By (2.2), we have, for each  ,
,  , and therefore
, and therefore

Thus,  . Now it is easy to see that
. Now it is easy to see that  . Consequently, if
. Consequently, if  is bounded, then
is bounded, then  for all
for all  . The constant
. The constant  is the best possible since
is the best possible since  for
for  satisfies
satisfies  for each
for each  . It should be mentioned that the above proof is essentially due to P. Šemrl [5, Proof of Theorem
. It should be mentioned that the above proof is essentially due to P. Šemrl [5, Proof of Theorem  and Proposition
and Proposition  ] (cf. [6, Proposition
] (cf. [6, Proposition  ]).
]).
Suppose that  is an unbounded functional satisfying the inequality (2.2). Since
is an unbounded functional satisfying the inequality (2.2). Since  is unbounded, there exists a sequence
is unbounded, there exists a sequence  such that
such that  . Take
. Take  arbitrarily. Set
arbitrarily. Set

By (2.1),  . Thus either
. Thus either  or
or  is an infinite subset of
is an infinite subset of  . First we consider the case when
. First we consider the case when  is infinite. Take
is infinite. Take  arbitrarily. Choose
arbitrarily. Choose  with
with  . Since
. Since  is assumed to be infinite, for each
is assumed to be infinite, for each  there exists
there exists  such that
such that  . Then
. Then  is a subsequence of
is a subsequence of  with
with  for every
for every  . By the choice of
. By the choice of  , we have
, we have

Thus we may and do assume that  for every
for every  . By (2.2) we have, for each
. By (2.2) we have, for each  and
and  ,
,  . According to (2.5), we have
. According to (2.5), we have

Consequently, we have, for each 

Since  , we have
, we have  for every
for every  . Applying (2.7), we have
. Applying (2.7), we have

By (2.2) and (2.5), we have

Consequently, we have by (2.8) and (2.7)

Next we consider the case when  is infinite. By a quite similar argument as in the case when
is infinite. By a quite similar argument as in the case when  is infinite, we see that there exists a subsequence
is infinite, we see that there exists a subsequence  such that
such that  for every
for every  . Then
. Then

In the same way as in the proof of (2.7), we have

for every  . According to (2.2) and (2.11), we have
. According to (2.2) and (2.11), we have

Since  for every
for every  , (2.11) and (2.12) show that
, (2.11) and (2.12) show that

Consequently, if  is unbounded, then
is unbounded, then  for all
for all  .
.
Remark 2.2.
Let  be a mapping from a commutative semigroup
be a mapping from a commutative semigroup  into itself. We define the binary operation
into itself. We define the binary operation  by
by  for each
for each  . Then
. Then  satisfies (2.1) since
satisfies (2.1) since

for all  . Therefore, Theorem 2.1 is a generalization of Najdecki [4, Theorem
. Therefore, Theorem 2.1 is a generalization of Najdecki [4, Theorem  ] and Baker [3, Theorem
] and Baker [3, Theorem  ].
].
Remark 2.3.
Let  be a set, and
be a set, and  . Suppose that
. Suppose that  has a binary operation
has a binary operation  such that, for each
such that, for each  , either
, either

If  satisfies (2.2) for some
satisfies (2.2) for some  , then by quite similar arguments to the proof of Theorem 2.1, we can prove that
, then by quite similar arguments to the proof of Theorem 2.1, we can prove that  for all
for all  , or
, or  for all
for all  . Thus, Theorem 2.1 is still true under the weaker condition (2.16) instead of (2.2). This was pointed out by the referee of this paper. The condition (2.16) is related to that introduced by Kannappan [7].
. Thus, Theorem 2.1 is still true under the weaker condition (2.16) instead of (2.2). This was pointed out by the referee of this paper. The condition (2.16) is related to that introduced by Kannappan [7].
Example 2.4.
Let  and
and  be mappings from a semigroup
be mappings from a semigroup  into itself with the following properties.
into itself with the following properties.
(a) for every
for every  .
.
(b) .
.
(c) for every
for every  .
.
If we define  for each
for each  , then we have
, then we have  for every
for every  . In fact, if
. In fact, if  , then we have
, then we have

as claimed.
Let  be a ring homomorphism from
be a ring homomorphism from  into itself, that is,
into itself, that is,  and
and  for each
for each  . It is well known that there exist infinitely many such homomorphisms on
. It is well known that there exist infinitely many such homomorphisms on  (cf. [8, 9]). If
(cf. [8, 9]). If  is not identically
is not identically  , then we see that
, then we see that  for every
for every  , the field of all rational real numbers. Thus, if we consider the case when
, the field of all rational real numbers. Thus, if we consider the case when  ,
,  a nonzero ring homomorphism, and
a nonzero ring homomorphism, and  , then
, then  satisfies the conditions (a), (b), and (c).
satisfies the conditions (a), (b), and (c).
If we define  for each
for each  , then
, then  holds for every
holds for every  . In fact,
. In fact,

Example 2.5.
Let  and, let
and, let  . We define the binary operation
. We define the binary operation  by
by

for each  . Then
. Then  satisfies the condition (2.1). In fact, let
satisfies the condition (2.1). In fact, let  .
.
(a)If  , then we have
, then we have

(b)If  , then
, then

(c)If  and
and  , then
, then

(d)If  and
and  , then
, then

(e)If  , then we have
, then we have

Therefore,  satisfies the condition (2.1). On the other hand, if
satisfies the condition (2.1). On the other hand, if  , then
, then

Thus,  in general. In the same way, we see that if
in general. In the same way, we see that if  , then
, then  need not to be true.
need not to be true.
References
Ulam SM: A Collection of Mathematical Problems, Interscience Tracts in Pure and Applied Mathematics, no. 8. Interscience, New York, NY, USA; 1960:xiii+150.
Hyers DH: On the stability of the linear functional equation. Proceedings of the National Academy of Sciences of the United States of America 1941, 27: 222–224. 10.1073/pnas.27.4.222
Baker JA: The stability of the cosine equation. Proceedings of the American Mathematical Society 1980,80(3):411–416. 10.1090/S0002-9939-1980-0580995-3
Najdecki A: On stability of a functional equation connected with the Reynolds operator. Journal of Inequalities and Applications 2007, 2007:-3.
Šemrl P: Non linear perturbations of homomorphisms on
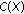 The Quarterly Journal of Mathematics. Series 2 1999, 50: 87–109. 10.1093/qjmath/50.197.87
The Quarterly Journal of Mathematics. Series 2 1999, 50: 87–109. 10.1093/qjmath/50.197.87Jarosz K: Perturbations of Banach Algebras, Lecture Notes in Mathematics. Volume 1120. Springer, Berlin, Germany; 1985:ii+118.
Kannappan Pl: On quadratic functional equation. International Journal of Mathematical and Statistical Sciences 2000,9(1):35–60.
Charnow A: The automorphisms of an algebraically closed field. Canadian Mathematical Bulletin 1970, 13: 95–97. 10.4153/CMB-1970-019-3
Kestelman H: Automorphisms of the field of complex numbers. Proceedings of the London Mathematical Society. Second Series 1951, 53: 1–12. 10.1112/plms/s2-53.1.1
Acknowledgments
The authors would like to thank the referees for valuable suggestions and comments to improve the manuscript. The first and fourth authors were partly supported by the Grant-in-Aid for Scientific Research.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Miura, T., Takagi, H., Tsukada, M. et al. Superstability of Generalized Multiplicative Functionals. J Inequal Appl 2009, 486375 (2009). https://doi.org/10.1155/2009/486375
Received:
Accepted:
Published:
DOI: https://doi.org/10.1155/2009/486375
 The Quarterly Journal of Mathematics. Series 2 1999, 50: 87–109. 10.1093/qjmath/50.197.87
The Quarterly Journal of Mathematics. Series 2 1999, 50: 87–109. 10.1093/qjmath/50.197.87